How do you analyze the circuit shown in the image?

Steps to Solve

1. Identify the Components and Connections

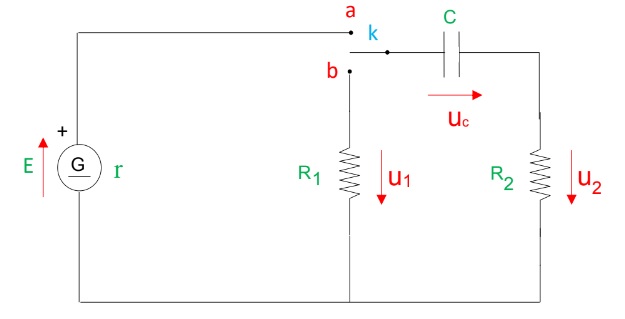
In the circuit, identify the components: a voltage source (EMF $E$), a resistor $R_1$, a capacitor $C$, and a second resistor $R_2$. Note the points $a$, $b$, and $k$ indicating connection points.

2. Apply Kirchhoff's Voltage Law

Use Kirchhoff's Voltage Law (KVL), which states that the sum of the electrical potential differences (voltage) around any closed circuit loop must equal zero.

For the loop $E - U_1 - U_C - U_2 = 0$, we can express the voltages as:

$$ E - U_1 - U_C - U_2 = 0 $$

3. Express Voltages in Terms of Currents and Resistances

From Ohm's Law, we know that the voltage across a resistor is given by $U = IR$, where $I$ is the current through the resistor. Therefore, we can express $U_1$ and $U_2$ as:

$$ U_1 = I_1 R_1 $$

$$ U_2 = I_2 R_2 $$

4. Consider Capacitor Voltage Relation

The voltage across the capacitor $C$ is given by:

$$ U_C = \frac{q}{C} $$

where $q$ is the charge stored in the capacitor. The relationship between current $I$ and charge $q$ is defined as:

$$ I = \frac{dq}{dt} $$

5. Set Up the Differential Equation

Substituting for $U_1$, $U_2$, and $U_C$ into the KVL equation gives:

$$ E - I_1 R_1 - \frac{q}{C} - I_2 R_2 = 0 $$

This forms the basis for a differential equation that describes the circuit's behavior over time.