∑(n=1 to ∞) (3/4)^n = 1.
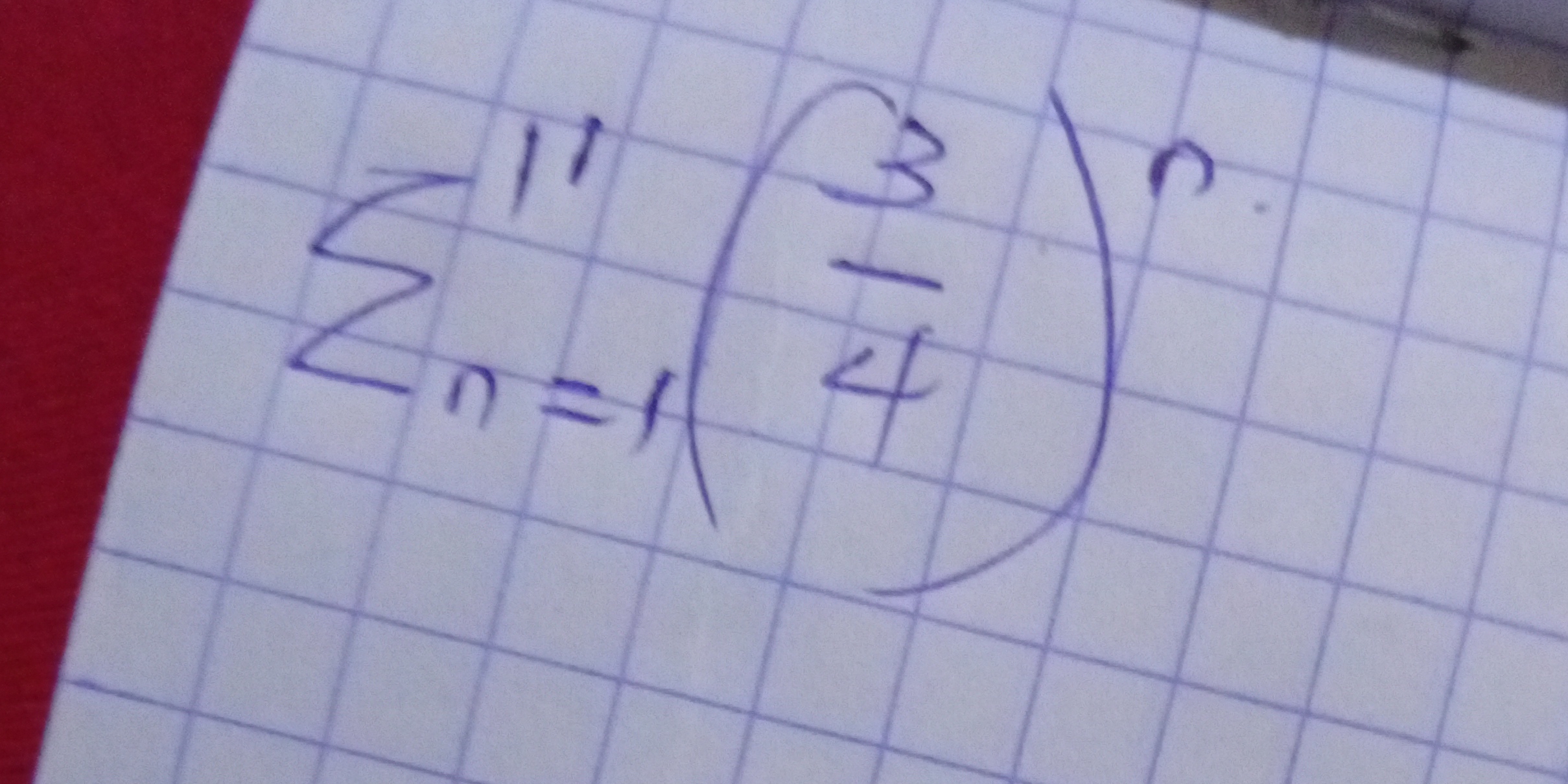
Understand the Problem
The question is addressing a mathematical series or summation with parameters involving both series notation and a fraction. It seems to ask for the evaluation of this series, likely summing from n=1 to some limit with a specific expression provided.
Answer
The sum of the series is \( S = 3 \).
Answer for screen readers
The sum of the series is ( S = 3 ).
Steps to Solve
-
Identify the series The series presented is $\sum_{n=1}^{\infty} \left(\frac{3}{4}\right)^n$. This is an infinite geometric series where the first term $a$ is $\frac{3}{4}$ and the common ratio $r$ is also $\frac{3}{4}$.
-
Use the formula for the sum of an infinite geometric series The formula for the sum ( S ) of an infinite geometric series is given by: $$ S = \frac{a}{1 - r} $$ where ( |r| < 1 ). Both conditions are satisfied since ( r = \frac{3}{4} ) is less than 1.
-
Substitute the values into the formula Substituting ( a = \frac{3}{4} ) and ( r = \frac{3}{4} ) into the formula: $$ S = \frac{\frac{3}{4}}{1 - \frac{3}{4}} $$
-
Simplify the expression Calculate the denominator: $$ 1 - \frac{3}{4} = \frac{1}{4} $$
Now substitute this back into the expression for ( S ): $$ S = \frac{\frac{3}{4}}{\frac{1}{4}} $$
- Final computation Perform the division: $$ S = \frac{3}{4} \times 4 = 3 $$
The sum of the series is ( S = 3 ).
More Information
This infinite geometric series converges rapidly since the common ratio is less than 1. Geometric series are fundamental in mathematics, appearing in various applications from finance to physics.
Tips
- Confusing the formula for finite and infinite geometric series.
- Miscalculating the denominator; ensure you subtract correctly when using the formula.
AI-generated content may contain errors. Please verify critical information