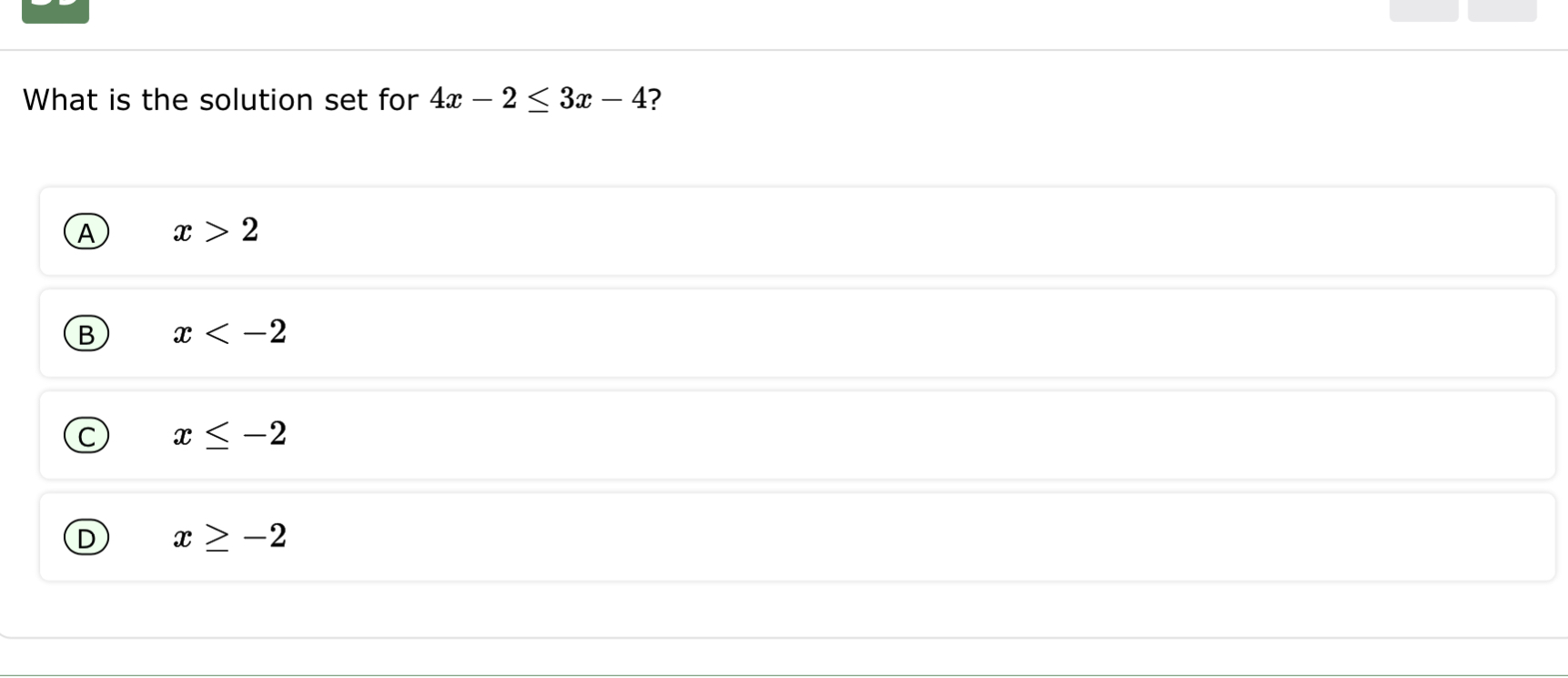
What is the solution set for 4x - 2 ≤ 3x - 4?
Understand the Problem
The question is asking to solve the inequality provided (4x - 2 ≤ 3x - 4) and to identify the solution set in terms of x. This involves isolating x and determining the range of values that satisfy the inequality.
Answer
The solution set is $x \leq -2$.
Answer for screen readers
The solution set is $x \leq -2$.
Steps to Solve
- Initial Inequality Setup
We start with the inequality:
$$ 4x - 2 \leq 3x - 4 $$
- Isolate x
Subtract $3x$ from both sides of the inequality:
$$ 4x - 3x - 2 \leq -4 $$
This simplifies to:
$$ x - 2 \leq -4 $$
- Add 2 to Both Sides
Next, we add 2 to both sides to isolate $x$:
$$ x \leq -4 + 2 $$
This simplifies further to:
$$ x \leq -2 $$
- Solution Set
The solution set is therefore:
$$ x \leq -2 $$
The solution set is $x \leq -2$.
More Information
This means that any value of $x$ that is less than or equal to -2 will satisfy the original inequality. Inequalities such as this one are useful in understanding ranges of values for algebraic expressions.
Tips
- Forgetting to flip the inequality sign when multiplying or dividing by a negative number (not applicable here as no such operation is involved).
- Miscalculating the arithmetic (such as incorrectly adding or subtracting the constants).
AI-generated content may contain errors. Please verify critical information