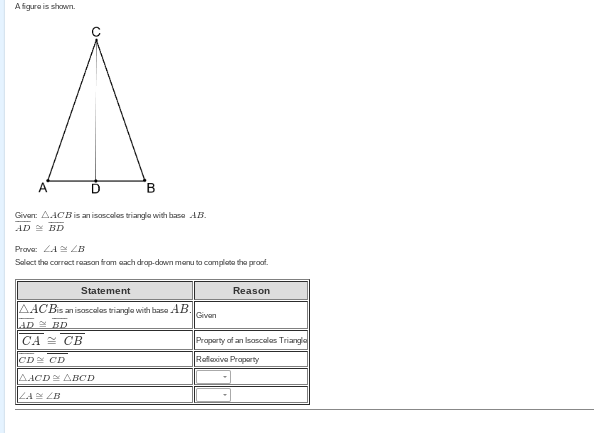
A figure is shown. Given: Triangle ACB is an isosceles triangle with base AB. AD is congruent to BD. Prove: Angle A is congruent to Angle B. Select the correct reason from each dro... A figure is shown. Given: Triangle ACB is an isosceles triangle with base AB. AD is congruent to BD. Prove: Angle A is congruent to Angle B. Select the correct reason from each drop-down menu to complete the proof.
Understand the Problem
The question is asking to complete a proof related to an isosceles triangle, specifically proving that angles A and B are equal given certain conditions about triangle ACB.
Answer
$$ \angle A \cong \angle B $$
Answer for screen readers
$$ \angle A \cong \angle B $$
Steps to Solve
- Identify Given Information
The problem states that triangle $ACB$ is an isosceles triangle with base $AB$. The given information includes:
- $AD \cong BD$.
- Define Importance of Isosceles Triangle
In isosceles triangles, the angles opposite the equal sides are also equal. Since $AD \cong BD$, we can state that:
$$ \angle ACB \cong \angle ABC $$
This is a property of isosceles triangles.
- Use Reflexive Property
We also know that $CD \cong CD$ because any segment is equal to itself. This can be noted as:
$$ CD = CD $$
This is known as the Reflexive Property.
- Conclude Angle Equality
Since we have established that $AD \cong BD$ and $CD \cong CD$, we can conclude that:
$$ \angle ACD \cong \angle BCD $$
Thus, from the triangle, it follows that:
$$ \angle A \cong \angle B $$
$$ \angle A \cong \angle B $$
More Information
In an isosceles triangle, the two angles at the base are equal. This property stems from the definition of isosceles triangles, which have at least two sides of equal length.
Tips
- Assuming that equal sides guarantee that the angles are adjacent, when they are, in fact, opposite to the equal sides.
- Neglecting to clearly state the properties used in each step of the proof.
AI-generated content may contain errors. Please verify critical information