∫xe^x dx के लिए चार विकल्प दिए गए हैं। यदि A=[2+10−i0] और B=[1-i2i0] का मान बताओ। tan^(-1)(√3)−sec^−1(−2) का मान ज्ञात कीजिये। ∫xe^x dx के लिए चार विकल्प दिए गए हैं। यदि A=[2+10−i0] और B=[1-i2i0] का मान बताओ। tan^(-1)(√3)−sec^−1(−2) का मान ज्ञात कीजिये।
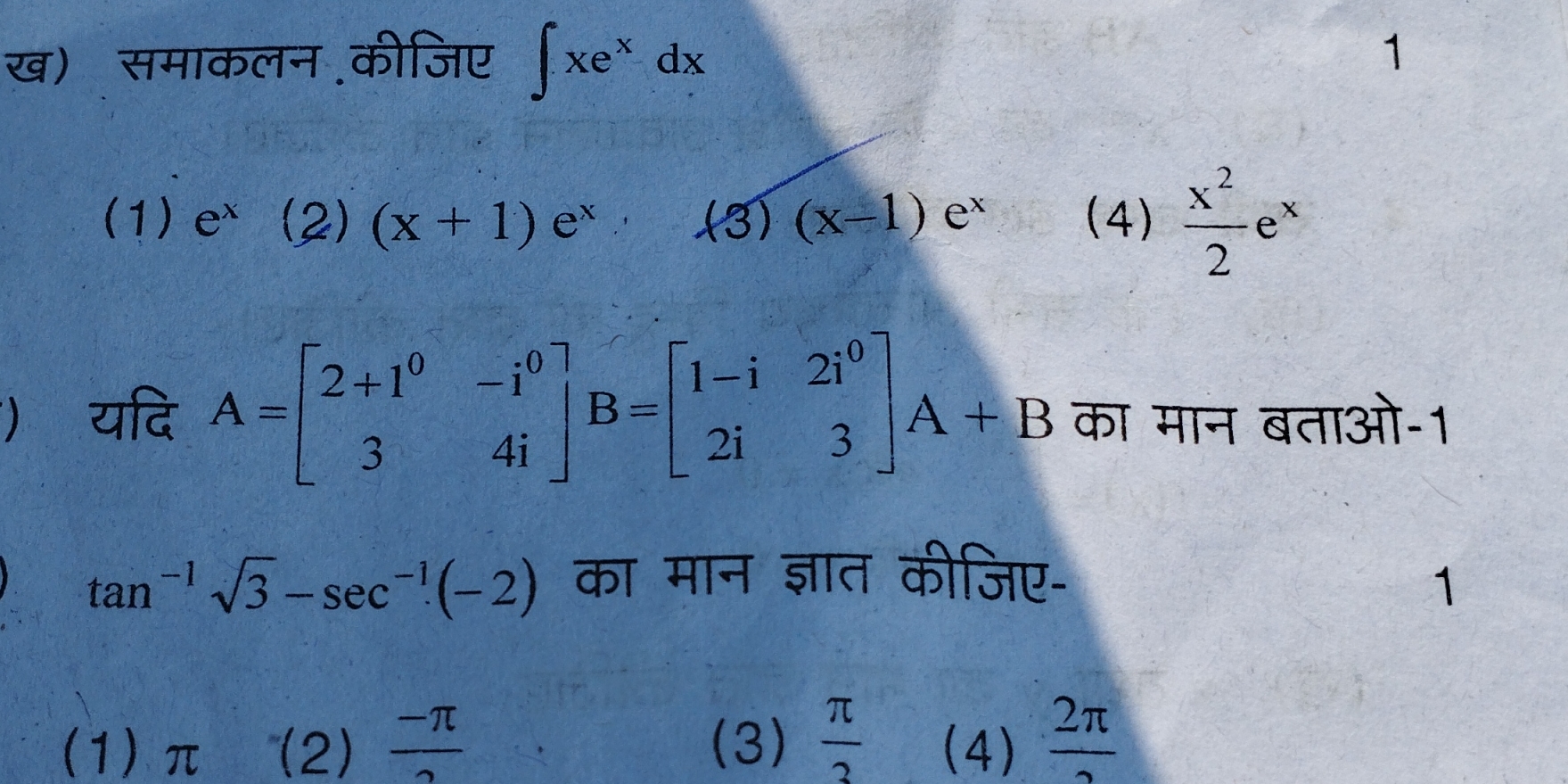
Understand the Problem
प्रश्न कुछ गणनाएँ करने के लिए कह रहा है, जिनमें एक दी गई कानूनी समीकरण का हल निकालना और दो मैट्रिक्स A और B का योग करना शामिल है।
Answer
The answers are: 1. $ (x - 1)e^x + C $ 2. $ A + B = \begin{bmatrix} 3 + 9i & 2i \\ 3 & 3 + 4i \end{bmatrix} $ 3. $ -\frac{\pi}{3} $
Answer for screen readers
The final answers are:
- $\int xe^x , dx = (x - 1)e^x + C$ (Option 3)
- $A + B = \begin{bmatrix} 3 + 9i & 2i \ 3 & 3 + 4i \end{bmatrix}$
- $tan^{-1}(\sqrt{3}) - sec^{-1}(-2) = -\frac{\pi}{3}$
Steps to Solve
- SIntegral Calculation of $\int xe^x dx$
To solve $\int xe^x dx$, we can use integration by parts. Let:
- $u = x$
- $dv = e^x dx$
Then, we find:
- $du = dx$
- $v = e^x$
Using the integration by parts formula:
$$ \int u , dv = uv - \int v , du $$
This gives:
$$ \int xe^x dx = x e^x - \int e^x dx $$
Now, calculating:
$$ \int e^x dx = e^x $$
So we have:
$$ \int xe^x dx = x e^x - e^x + C = (x - 1)e^x + C $$
- Identify the Correct Option
From the options given:
- $e^x$
- $(x + 1)e^x$
- $(x - 1)e^x$
- $\frac{x^2}{2} e^x$
The correct answer is option (3) $(x - 1)e^x$.
- Matrix Addition $(A + B)$
Given matrices:
$$ A = \begin{bmatrix} 2 + 10i & 0 \ 3 & 4i \end{bmatrix}, \quad B = \begin{bmatrix} 1 - i & 2i \ 0 & 3 \end{bmatrix} $$
To find $A + B$, we add corresponding elements:
$$ A + B = \begin{bmatrix} (2 + 10i) + (1 - i) & 0 + 2i \ 3 + 0 & 4i + 3 \end{bmatrix} $$
Calculating the elements:
- First element: $(2 + 10i) + (1 - i) = 3 + 9i$
- Second element: $0 + 2i = 2i$
- Third element: $3 + 0 = 3$
- Fourth element: $4i + 3 = 3 + 4i$
So,
$$ A + B = \begin{bmatrix} 3 + 9i & 2i \ 3 & 3 + 4i \end{bmatrix} $$
- Calculate $tan^{-1}(\sqrt{3}) - sec^{-1}(-2)$
Calculating these values separately:
-
$tan^{-1}(\sqrt{3}) = \frac{\pi}{3}$ (because $\tan \frac{\pi}{3} = \sqrt{3}$)
-
For $sec^{-1}(-2)$, we find: $$ sec^{-1}(-2) = \pi - sec^{-1}(2) $$ Since $sec^{-1}(2) = \frac{\pi}{3}$, thus: $$ sec^{-1}(-2) = \pi - \frac{\pi}{3} = \frac{2\pi}{3} $$
Putting it all together:
$$ tan^{-1}(\sqrt{3}) - sec^{-1}(-2) = \frac{\pi}{3} - \frac{2\pi}{3} = -\frac{\pi}{3} $$
The final answers are:
- $\int xe^x , dx = (x - 1)e^x + C$ (Option 3)
- $A + B = \begin{bmatrix} 3 + 9i & 2i \ 3 & 3 + 4i \end{bmatrix}$
- $tan^{-1}(\sqrt{3}) - sec^{-1}(-2) = -\frac{\pi}{3}$
More Information
The integration by parts technique is crucial for solving integrals involving products of polynomials and exponentials. For matrices, element-wise addition is straightforward. The inverse trigonometric functions can have their values derived from known angle identities.
Tips
- Confusing the order of operations when using integration by parts.
- Neglecting to add corresponding elements correctly in matrix addition.
- Misapplying properties of inverse trigonometric functions, especially with negative values.
AI-generated content may contain errors. Please verify critical information