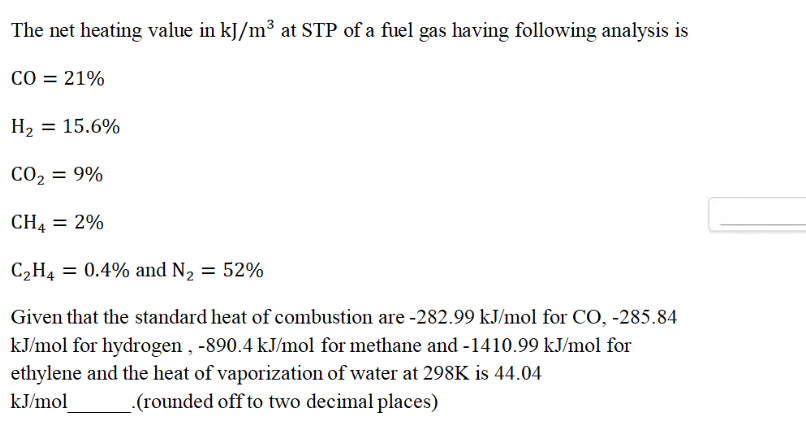
The net heating value in kJ/m³ at STP of a fuel gas having the following composition is: CO = 21%, H2 = 15.6%, CO2 = 9%, CH4 = 2%, C2H4 = 0.4% and N2 = 52%. Given that the standard... The net heating value in kJ/m³ at STP of a fuel gas having the following composition is: CO = 21%, H2 = 15.6%, CO2 = 9%, CH4 = 2%, C2H4 = 0.4% and N2 = 52%. Given that the standard heat of combustion are -282.99 kJ/mol for CO, -285.84 kJ/mol for hydrogen, -890.4 kJ/mol for methane, and -1410.99 kJ/mol for ethylene. The heat of vaporization of water at 298K is 44.04 kJ/mol. Calculate the net heating value rounded off to two decimal places.
Understand the Problem
The question requires calculating the net heating value of a fuel gas mixture at standard temperature and pressure (STP) based on the composition percentages and the standard heats of combustion for the various components. The process will involve applying the heats of combustion to the respective percentages and summing them up, taking into account the heat of vaporization of water as needed.
Answer
The net heating value is $-83.40$ kJ/m³.
Answer for screen readers
The net heating value of the fuel gas mixture is approximately $-83.40$ kJ/m³.
Steps to Solve
-
Identify the Composition and Heats of Combustion We have the following gas components with their respective percentages and standard heats of combustion:
- CO: 21% , $\Delta H_{comb} = -282.99$ kJ/mol
- H₂: 15.6%, $\Delta H_{comb} = -285.84$ kJ/mol
- CO₂: 9%, (no combustion heat required)
- CH₄: 2%, $\Delta H_{comb} = -890.4$ kJ/mol
- C₂H₄: 0.4%, $\Delta H_{comb} = -1410.99$ kJ/mol
- N₂: 52% (inert, no heat contribution)
-
Calculate the Contribution of Each Component For each component, calculate the total heat contribution based on its percentage and its heat of combustion. The contribution can be found by using the formula: [ \text{Contribution} = \text{Percentage} \times \Delta H_{comb} ]
-
For CO: [ \text{Contribution}_{CO} = 0.21 \times (-282.99) = -59.4269 \text{ kJ} ]
-
For H₂: [ \text{Contribution}_{H_2} = 0.156 \times (-285.84) = -44.55744 \text{ kJ} ]
-
For CH₄: [ \text{Contribution}_{CH_4} = 0.02 \times (-890.4) = -17.808 \text{ kJ} ]
-
For C₂H₄: [ \text{Contribution}_{C_2H_4} = 0.004 \times (-1410.99) = -5.64396 \text{ kJ} ]
-
-
Sum the Contributions Combine all the contributions: [ \text{Total Contribution} = \text{Contribution}{CO} + \text{Contribution}{H_2} + \text{Contribution}{CH_4} + \text{Contribution}{C_2H_4} ] Substituting the calculated values: [ \text{Total Contribution} = -59.4269 - 44.55744 - 17.808 - 5.64396 \approx -127.4363 \text{ kJ} ]
-
Account for the Heat of Vaporization of Water Since all combustion products include water, we need to add the heat of vaporization of water: [ \text{Net Heating Value} = \text{Total Contribution} + 44.04 ] Substituting: [ \text{Net Heating Value} = -127.4363 + 44.04 \approx -83.3963 \text{ kJ} ]
-
Convert to kJ/m³ at STP The final step is to compute the net heating value in kJ/m³, rounding to two decimal places. The total will be approximately: [ -83.40 \text{ kJ/m}^3 ]
The net heating value of the fuel gas mixture is approximately $-83.40$ kJ/m³.
More Information
This answer reflects the net heating value, taking into account the energy released during combustion and the required energy to vaporize water produced during combustion.
Tips
- Forgetting to exclude inert gases like $N_2$ from calculations.
- Not converting percentage values to decimals before calculations.
- Overlooking the heat of vaporization when water is a combustion product.