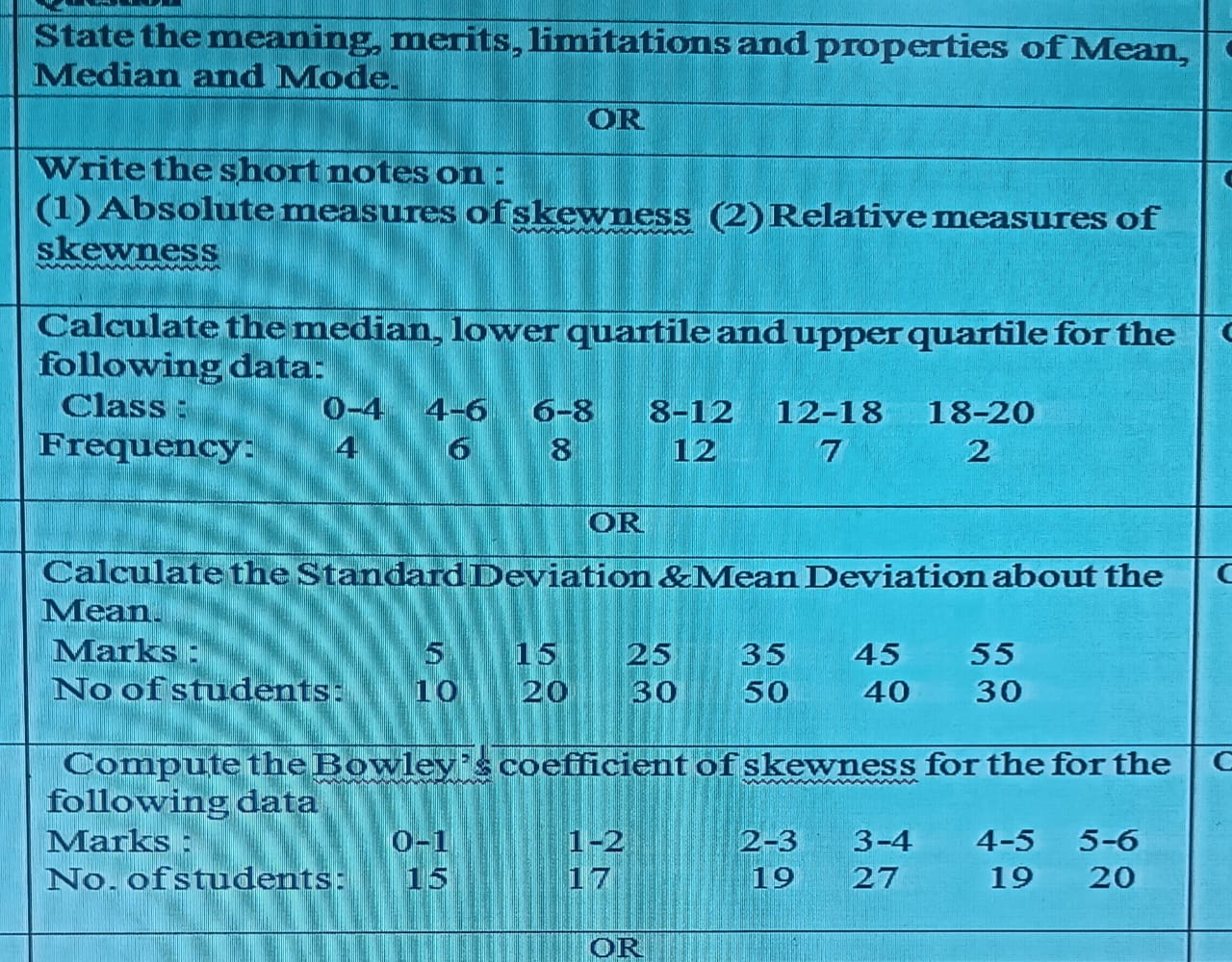
State the meaning, merits, limitations and properties of Mean, Median and Mode. Write short notes on: (1) Absolute measures of skewness (2) Relative measures of skewness. Calculate... State the meaning, merits, limitations and properties of Mean, Median and Mode. Write short notes on: (1) Absolute measures of skewness (2) Relative measures of skewness. Calculate the median, lower quartile, and upper quartile for the following data: Class: 0-4, 4-6, 6-8, 8-12, 12-18, 18-20; Frequency: 4, 6, 8, 12, 7, 2. Calculate the Standard Deviation & Mean Deviation about the Mean. Marks: 5, 15, 25, 35, 45, 55; No of students: 10, 20, 30, 50, 40, 30. Compute the Bowley's coefficient of skewness for the following data: Marks: 0-1, 1-2, 2-3, 3-4, 4-5, 5-6; No of students: 15, 17, 19, 27, 19, 20. Read more

Steps to Solve

1. Organize the Data The data is provided in classes and frequencies. For calculating the median and quartiles, we first create a cumulative frequency table.

   Class	Frequency	Cumulative Frequency
   0-4	4	4
   4-6	6	10
   6-8	8	18
   8-12	12	30
   12-18	7	37
   18-20	2	39

2. Calculate the Total Frequency The total frequency (N) is the sum of all frequencies. $$ N = 4 + 6 + 8 + 12 + 7 + 2 = 39 $$

3. Find the Median To find the median, identify the median class. Since $N = 39$, the median will be at position $19.5$. The median class is the one where the cumulative frequency reaches 19.

   • Median class: 8-12 (cumulative frequency 30)
   • Median calculated by: $$ \text{Median} = L + \left(\frac{N/2 - CF}{f}\right) \times h $$ Where:
   • $L = 8$ (lower boundary of median class)
   • $CF = 18$ (cumulative frequency of the class before median class)
   • $f = 12$ (frequency of median class)
   • $h = 4$ (class width)

   Calculate median: $$ \text{Median} = 8 + \left(\frac{19.5 - 18}{12}\right) \times 4 $$ $$ \text{Median} = 8 + \left(\frac{1.5}{12}\right) \times 4 $$ $$ \text{Median} = 8 + 0.5 = 8.5 $$

4. Calculate Lower and Upper Quartiles (Q1 and Q3)

   • For Q1 (lower quartile):

     • Position: $N/4 = 9.75$, so the Q1 class is 6-8.
     • Using a similar formula: $$ Q1 = L + \left(\frac{N/4 - CF}{f}\right) \times h $$ $$ Q1 = 6 + \left(\frac{9.75 - 10}{8}\right) \times 2 $$ $$ Q1 = 6 + \left(-0.03125\right) \times 2 $$ $$ Q1 = 6 - 0.0625 = 5.9375 $$

   • For Q3 (upper quartile):

     • Position: $3N/4 = 29.25$, so the Q3 class is 12-18.
     • Using a similar formula: $$ Q3 = 12 + \left(\frac{29.25 - 30}{7}\right) \times 6 $$ $$ Q3 = 12 + \left(-0.10714\right) \times 6 $$ $$ Q3 = 12 - 0.6428 = 11.3572 $$

5. Summarize Results

   • Median: $8.5$
   • Lower Quartile (Q1): $5.9375$
   • Upper Quartile (Q3): $11.3572$