Which expression represents the surface area of the prism?
Understand the Problem
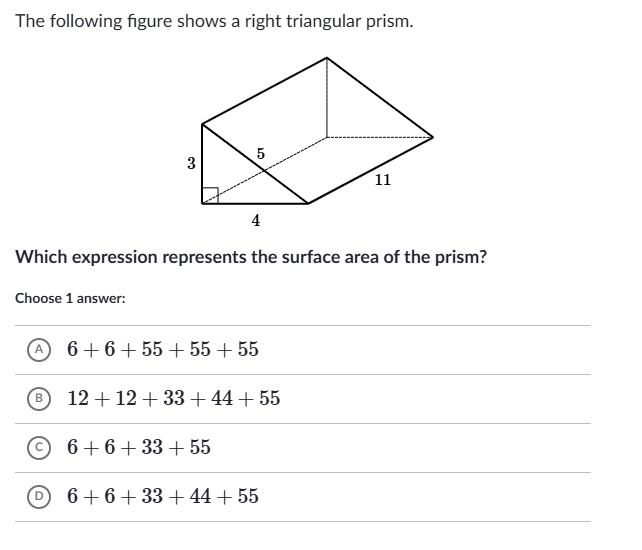
The question is asking for an expression that represents the surface area of a right triangular prism based on the dimensions provided in the figure. To solve it, we need to calculate the areas of the triangular and rectangular faces, then combine them.
Answer
$$ 6 + 6 + 33 + 44 + 55 $$
Answer for screen readers
The expression that represents the surface area of the prism is: $$ 6 + 6 + 33 + 44 + 55 $$
Steps to Solve
-
Identify the triangular base area The base of the prism is a right triangle. To find the area of this triangle, use the formula: $$ A = \frac{1}{2} \times \text{base} \times \text{height} $$ Here, the base is 4 and the height is 3: $$ A = \frac{1}{2} \times 4 \times 3 = 6 $$
-
Calculate the lateral surface area The lateral surface area consists of three rectangles. Each rectangle's area is calculated as:
- Rectangle 1: Area = Base × Height = 4 × 11 = 44
- Rectangle 2: Area = Height × Height = 3 × 11 = 33
- Rectangle 3: Area = Hypotenuse × Height. The hypotenuse can be calculated using the Pythagorean theorem: $$ \text{Hypotenuse} = \sqrt{4^2 + 3^2} = 5 $$ So, Rectangle 3: Area = 5 × 11 = 55.
- Sum the areas of all faces Now, add the area of the triangular bases and the lateral areas:
- Area of two triangular bases: $2 \times 6 = 12$
- Lateral areas: $44 + 33 + 55 = 132$
Total surface area is: $$ \text{Total Area} = 12 + 132 = 144 $$
But since the problem asks for the expression representing this surface area, we'll write it in terms of the summed up components: $$ \text{Total Area} = 6 + 6 + 44 + 33 + 55 $$
The expression that represents the surface area of the prism is: $$ 6 + 6 + 33 + 44 + 55 $$
More Information
The calculation reflects the surface area of a right triangular prism, which includes both the triangular bases and the rectangular sides. The indicators show the contributions from each section of the prism.
Tips
- Forgetting to multiply by 2 for both triangular bases: Make sure to account for both triangles when calculating the surface area.
- Miscalculating rectangle dimensions: Carefully double-check each dimension when calculating the areas of the rectangle faces.
AI-generated content may contain errors. Please verify critical information