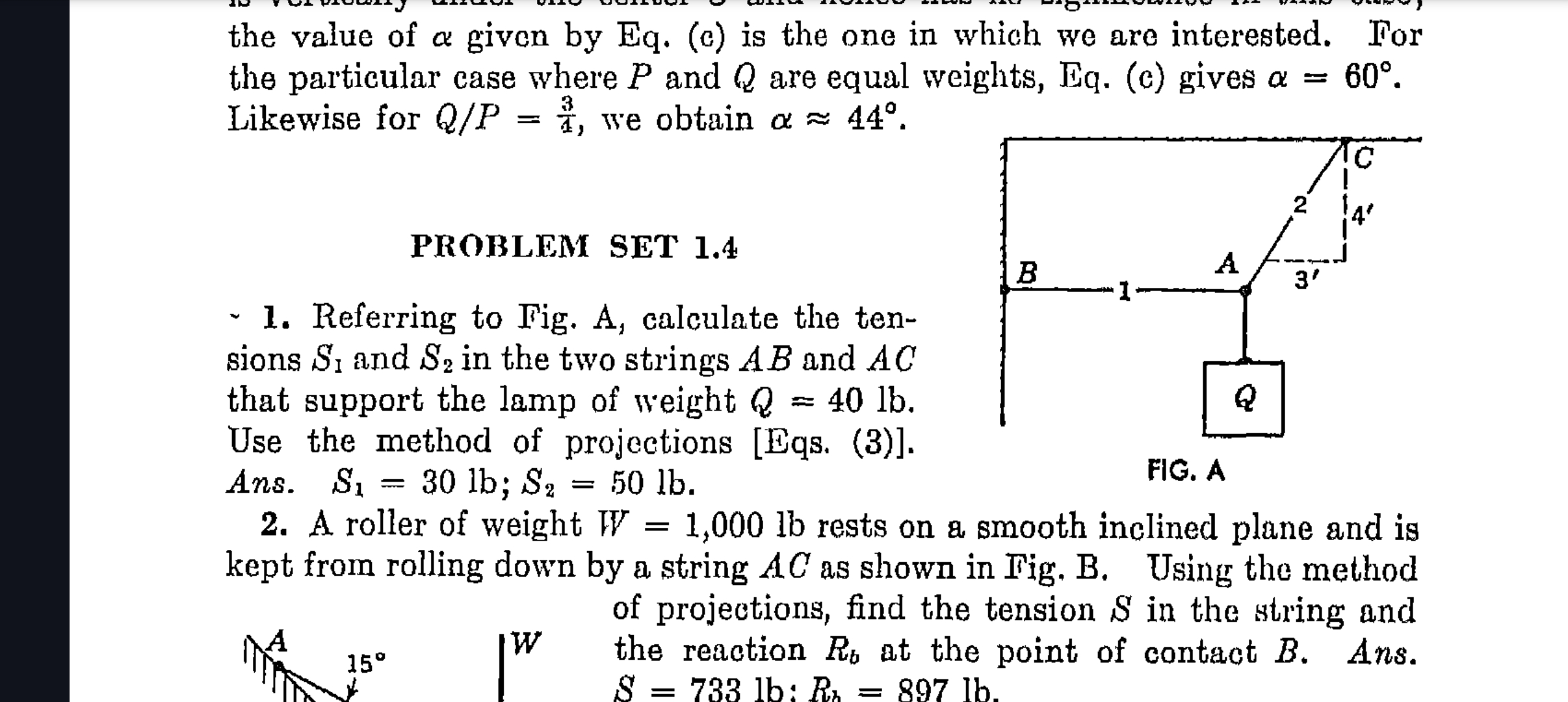
Referring to Fig. A, calculate the tensions S1 and S2 in the two strings AB and AC that support the lamp of weight Q = 40 lb. Use the method of projections [Eqs. (3)].

Steps to Solve

1. Identify Forces Acting on the Lamp The lamp has a weight $Q = 40 \text{ lb}$ acting downward. There are also tension forces $S_1$ in string AB and $S_2$ in string AC acting upward.

2. Resolve Tensions into Components Since the strings create angles with the horizontal, we will resolve the tensions into their vertical ($y$) and horizontal ($x$) components:

• For string AB (let's assume it makes an angle $\theta_1$ with the horizontal):

  • Vertical component: $S_1 \sin(\theta_1)$
  • Horizontal component: $S_1 \cos(\theta_1)$

• For string AC (assuming it makes an angle $\theta_2$ with the horizontal):

  • Vertical component: $S_2 \sin(\theta_2)$
  • Horizontal component: $S_2 \cos(\theta_2)$

3. Set up Equilibrium Equations For static equilibrium, the sum of vertical forces and the sum of horizontal forces must equal zero:

• Vertical forces: $$ S_1 \sin(\theta_1) + S_2 \sin(\theta_2) = Q $$

• Horizontal forces: $$ S_1 \cos(\theta_1) = S_2 \cos(\theta_2) $$

4. Substitute Given Values and Angles Using the known angles (determined from the figure, you need the angles for proper calculation), and substituting for $Q$: Assuming $\theta_1 = 60^\circ$ and $\theta_2 = 30^\circ$:

• From the vertical forces: $$ S_1 \sin(60^\circ) + S_2 \sin(30^\circ) = 40 $$

5. Solve for One Tension in Terms of the Other Using the horizontal forces equation to express one tension in terms of the other: $$ S_1 = S_2 \frac{\cos(\theta_2)}{\cos(\theta_1)} $$

6. Combine and Solve the Equations Substituting the expression from the horizontal forces into the vertical forces equation will give you one equation with one unknown. Solve for $S_2$ and then substitute back to find $S_1$.