∫(1 + 3x)x² dx
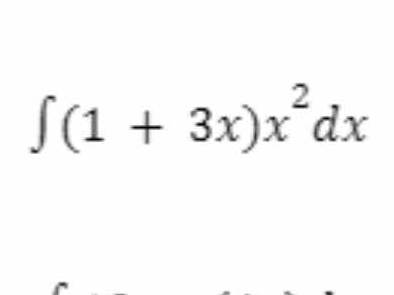
Understand the Problem
The question is asking for the integration of the expression (1 + 3x)x² with respect to x. This involves finding the antiderivative of the function, which may require expanding the expression and then applying integration rules.
Answer
$$\frac{x^{3}}{3} + \frac{3x^{4}}{4} + C$$
Answer for screen readers
$$\int (1 + 3x)x^2 , dx = \frac{x^{3}}{3} + \frac{3x^{4}}{4} + C$$
Steps to Solve
- Expand the Expression
First, distribute $x^2$ in the integrand:
$$(1 + 3x)x^2 = x^2 + 3x^3$$
- Set Up the Integral
Now, rewrite the integral with the expanded expression:
$$\int (x^2 + 3x^3) , dx$$
- Integrate Each Term
Next, integrate each term separately:
- The integral of $x^n$ is given by the formula:
$$\int x^n , dx = \frac{x^{n+1}}{n+1} + C$$
- For $x^2$, the integral is:
$$\int x^2 , dx = \frac{x^{3}}{3}$$
- For $3x^3$, the integral is:
$$\int 3x^3 , dx = 3 \cdot \frac{x^{4}}{4} = \frac{3x^{4}}{4}$$
- Combine the Results
Combine the results of the integrals:
$$\int (x^2 + 3x^3) , dx = \frac{x^{3}}{3} + \frac{3x^{4}}{4} + C$$
- Final Expression
The final expression for the integral is:
$$\frac{x^{3}}{3} + \frac{3x^{4}}{4} + C$$
$$\int (1 + 3x)x^2 , dx = \frac{x^{3}}{3} + \frac{3x^{4}}{4} + C$$
More Information
The resulting expression is the antiderivative of the given function. When integrating polynomial functions, expanding the product before integrating can simplify the process significantly. The constant ( C ) represents the constant of integration.
Tips
- Forgetting to include the constant of integration ( C ) at the end of the problem.
- Making errors during the distribution step or when applying the power rule for integration.
AI-generated content may contain errors. Please verify critical information