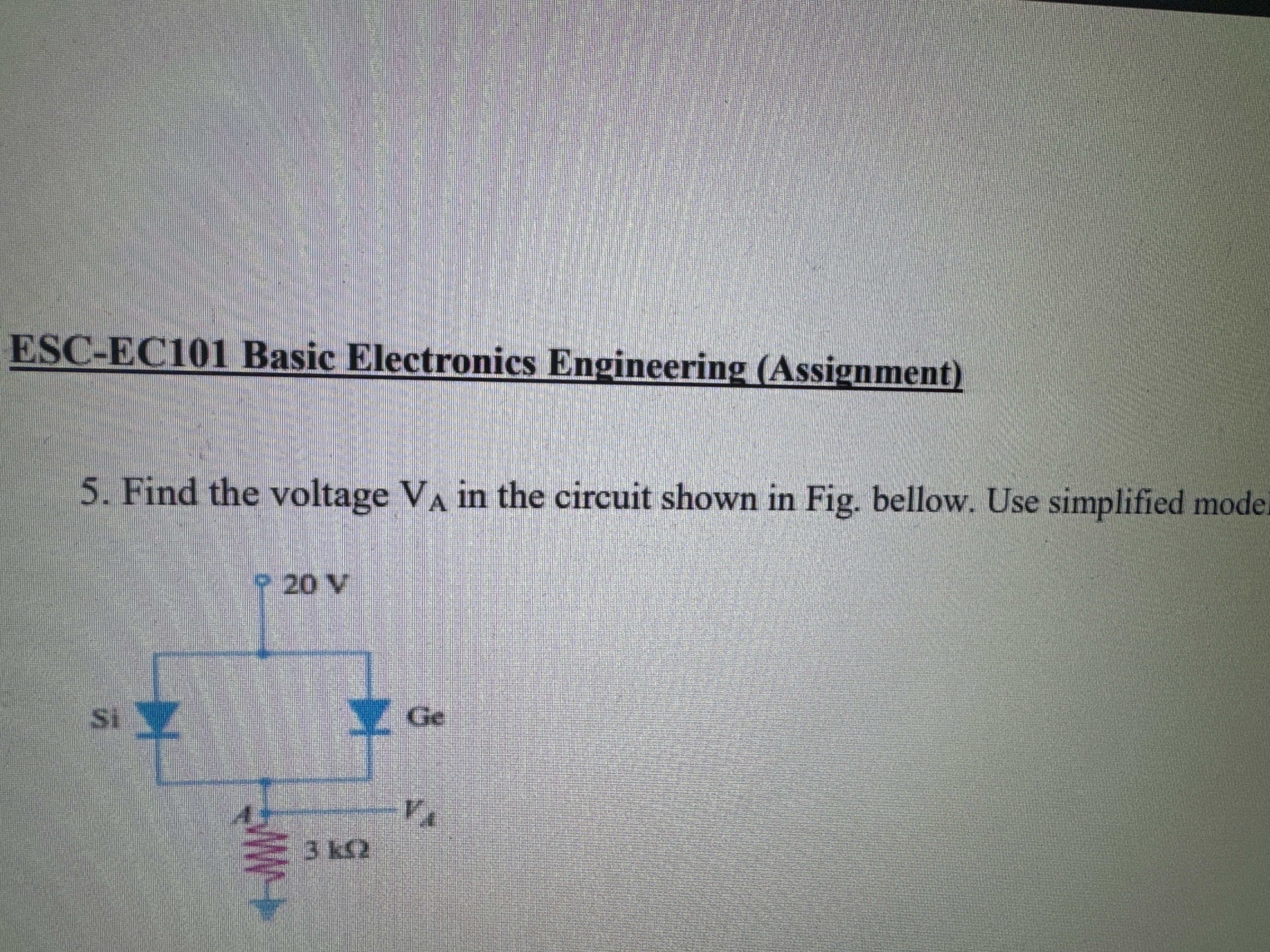
Find the voltage VA in the circuit shown in Fig. below. Use simplified model.
Understand the Problem
The question is asking to find the voltage VA in a circuit diagram based on the provided information and using simplified models. This involves applying concepts from basic electronics, likely involving Ohm's law and series/parallel circuit analysis.
Answer
The voltage $V_A$ is $20 \, V$.
Answer for screen readers
The voltage $V_A$ is $20 , V$.
Steps to Solve
- Identify the components in the circuit
From the circuit diagram, we have a voltage source of $20 , V$ and a resistor of $3 , k\Omega$. We need to find the voltage $V_A$ across the resistor.
- Apply Ohm's Law
Ohm's law states that $V = IR$, where $V$ is the voltage, $I$ is the current, and $R$ is the resistance.
- Calculate the total current in the circuit
The total voltage in the circuit is $20 , V$ applied across the resistor of $3 , k\Omega$. The current $I$ can be calculated as: $$ I = \frac{V}{R} = \frac{20 , V}{3000 , \Omega} $$
- Substitute and solve for the current
Calculating the current: $$ I = \frac{20}{3000} = 0.00667 , A \text{ or } 6.67 , mA $$
- Determine the voltage across the resistor
The voltage $V_A$ across the resistor is given by Ohm’s Law as follows: $$ V_A = I \times R = 0.00667 , A \times 3000 , \Omega $$
- Calculate and conclude
Substituting in the values gives: $$ V_A = 20 , V $$
The voltage $V_A$ is $20 , V$.
More Information
In this scenario, the voltage across the resistor equals the total applied voltage as it is in series with the circuit components, and the same current flows through them.
Tips
- Miscalculating the current: Ensure you properly divide the voltage by the resistance.
- Confusing series and parallel circuits: Make sure to recognize the configuration; in this case, the resistor is in series with the voltage source.
AI-generated content may contain errors. Please verify critical information