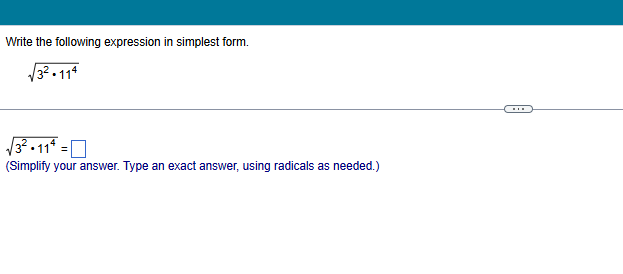
Write the following expression in simplest form: √(3² · 11⁴)
Understand the Problem
The question is asking to simplify the expression under the square root, which is (\sqrt{3^2 \cdot 11^4}). We need to simplify it to its simplest form.
Answer
The simplest form is \( 363 \).
Answer for screen readers
The simplest form of the expression is ( 363 ).
Steps to Solve
- Evaluate the expression inside the square root
Start by evaluating the powers inside the square root, which gives us: $$ \sqrt{3^2 \cdot 11^4} $$
- Apply the square root to each term
Use the property of square roots that states $\sqrt{a \cdot b} = \sqrt{a} \cdot \sqrt{b}$. So we can separate the square roots: $$ \sqrt{3^2} \cdot \sqrt{11^4} $$
- Calculate the values of each square root
Now, evaluate each square root: $$ \sqrt{3^2} = 3 \quad \text{and} \quad \sqrt{11^4} = 11^2 = 121 $$
- Combine the results
Now, multiply the results: $$ 3 \cdot 121 = 363 $$
The simplest form of the expression is ( 363 ).
More Information
The expression demonstrates the properties of exponents and square roots. Simplifying can reveal that ( \sqrt{a^2} = a ) and ( \sqrt{b^4} = b^2 ).
Tips
- Forgetting to simplify each component of the square root separately.
- Not applying square root properties correctly when separating terms.
AI-generated content may contain errors. Please verify critical information