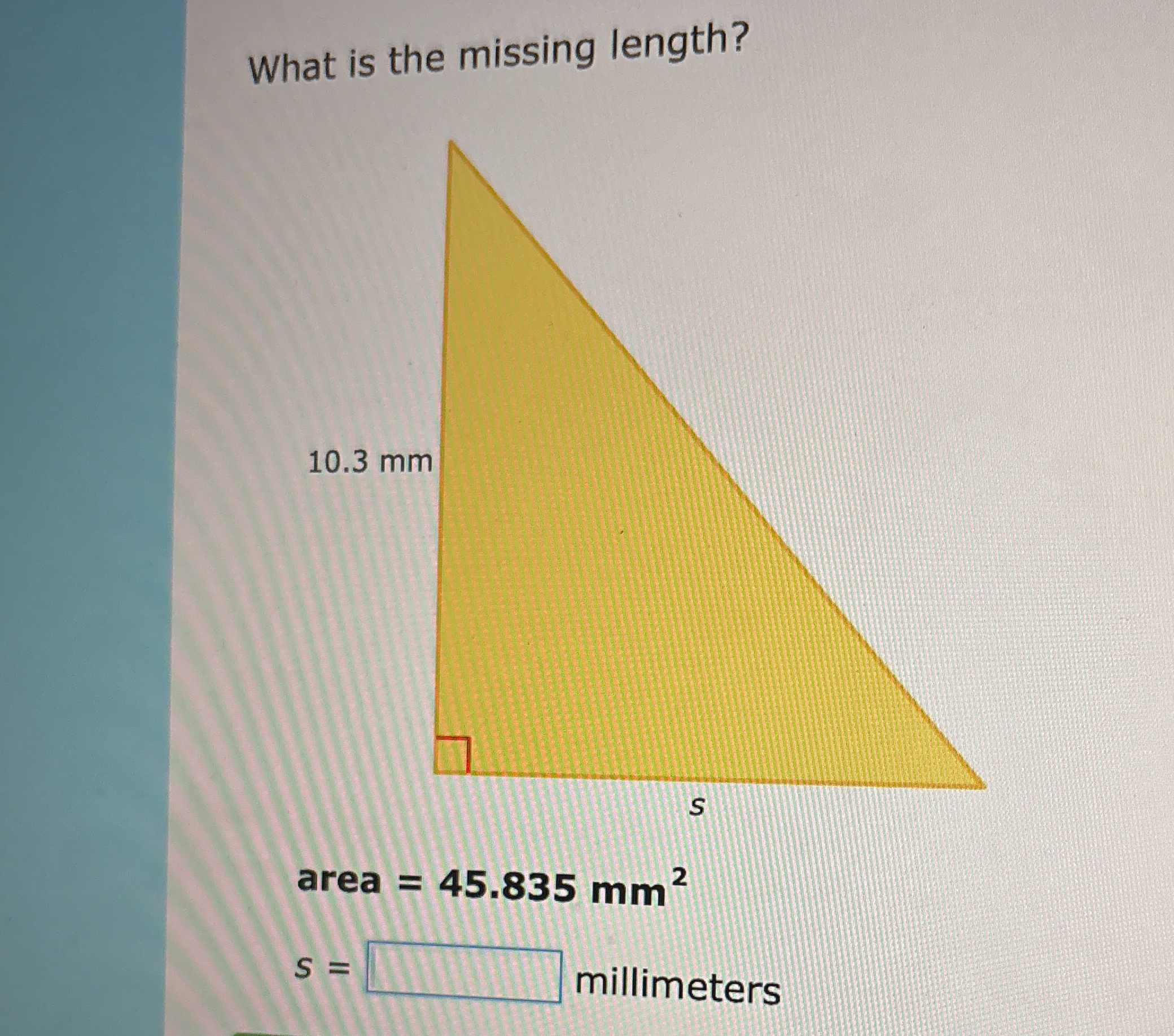
What is the missing length?

Steps to Solve

1. Set up the area formula for a triangle

We know the formula for the area of a triangle is:

$$ \text{Area} = \frac{1}{2} \times \text{base} \times \text{height} $$

Here, the area is given as 45.835 mm², and the base is 10.3 mm.

2. Substitute the known values into the formula

Plugging in the values we have:

$$ 45.835 = \frac{1}{2} \times 10.3 \times s $$

3. Isolate 's'

To find 's', first multiply both sides by 2:

$$ 2 \times 45.835 = 10.3 \times s $$

This simplifies to:

$$ 91.67 = 10.3 \times s $$

Next, divide both sides by 10.3 to isolate 's':

$$ s = \frac{91.67}{10.3} $$

4. Calculate the value of 's'

Now we perform the division:

$$ s \approx 8.9 \text{ mm} $$