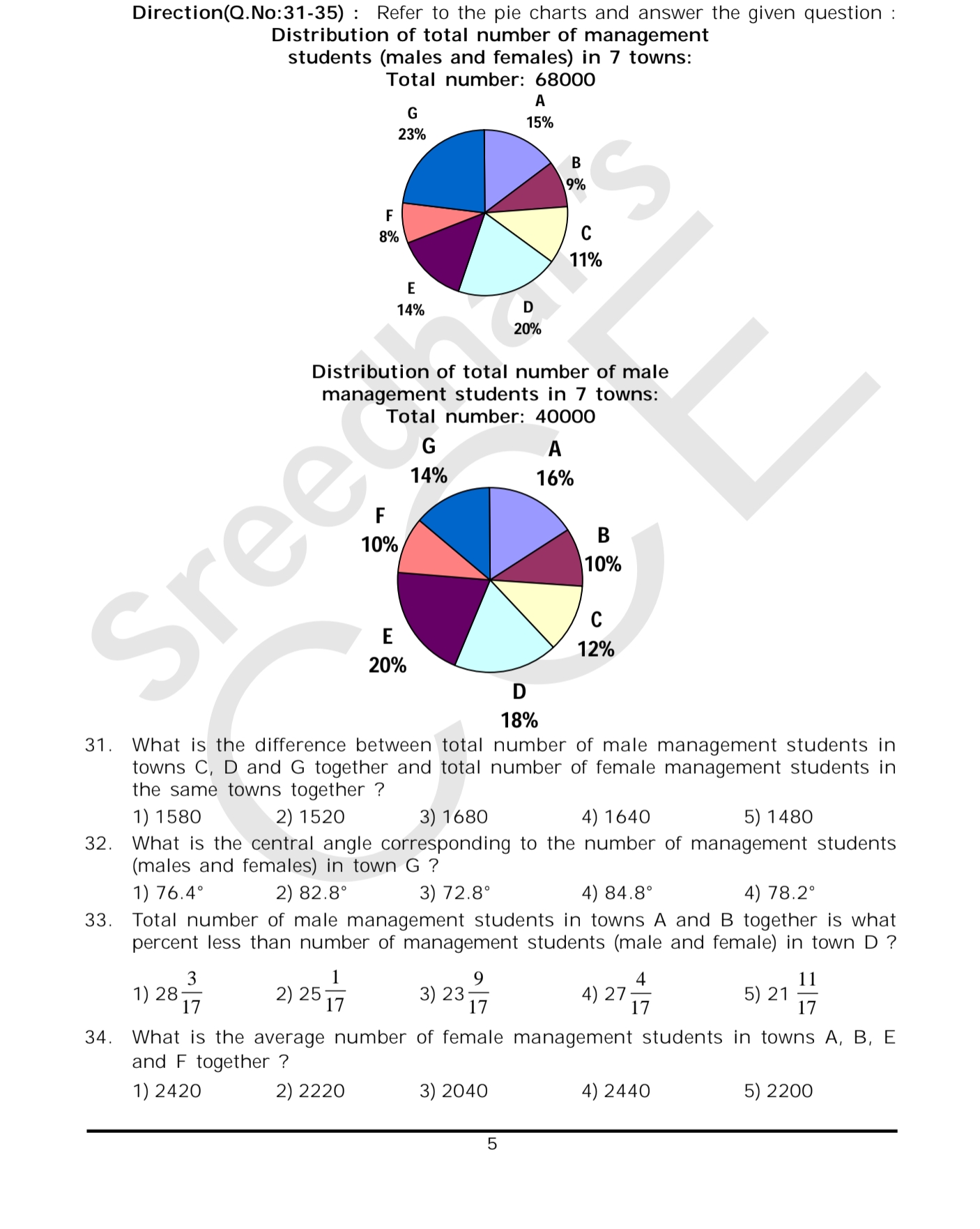
What is the difference between total number of male management students in towns C, D and G together and total number of female management students in the same towns together? What... What is the difference between total number of male management students in towns C, D and G together and total number of female management students in the same towns together? What is the central angle corresponding to the number of management students (males and females) in town G? Total number of male management students in towns A and B together is what percent less than number of management students (male and female) in town D? What is the average number of female management students in towns A, B, E and F together?
Understand the Problem
The question is asking about various aspects related to the distribution of management students in seven towns based on pie charts. Each part requires calculations involving the number of students in specific towns and categories.
Answer
- Difference: $19080$ - Angle: $82.4^{\circ}$ - Average: $7970$
Answer for screen readers
-
The absolute difference is $19080$.
-
The central angle for town G (females) is $82.4^{\circ}$.
-
The average number of female management students in towns A, B, E, and F together is $7970$.
Steps to Solve
- Calculate total management students in towns C, D, and G (males)
The total number of management students is 40,000. We need to find the number of male students in towns C, D, and G.
From the pie chart:
- C: $12%$ of 40,000
- D: $18%$ of 40,000
- G: $14%$ of 40,000
Calculating each:
- For C: $$ \text{Number of male students in C} = 0.12 \times 40000 = 4800 $$
- For D: $$ \text{Number of male students in D} = 0.18 \times 40000 = 7200 $$
- For G: $$ \text{Number of male students in G} = 0.14 \times 40000 = 5600 $$
Total for towns C, D, and G:
$$ \text{Total male in } C, D, G = 4800 + 7200 + 5600 = 17600 $$
- Calculate total female management students in towns C, D, and G
Next, we find the total female students in towns C, D, and G from the first pie chart, which has 68,000 students.
From the pie chart:
- C: $11%$ of 68,000
- D: $20%$ of 68,000
- G: $23%$ of 68,000
Calculating each:
- For C: $$ \text{Number of female students in C} = 0.11 \times 68000 = 7480 $$
- For D: $$ \text{Number of female students in D} = 0.20 \times 68000 = 13600 $$
- For G: $$ \text{Number of female students in G} = 0.23 \times 68000 = 15640 $$
Total for towns C, D, and G:
$$ \text{Total female in } C, D, G = 7480 + 13600 + 15640 = 36680 $$
- Calculate the difference between male and female students
Now we find the difference between the total male students and the total female students in towns C, D, and G.
$$ \text{Difference} = \text{Total males} - \text{Total females} = 17600 - 36680 = -19080 $$
However, since the question asks for the absolute difference:
$$ \text{Absolute Difference} = |17600 - 36680| = 19080 $$
- Calculate central angle for town G (female)
The central angle for town G's female students can be calculated using the total female students from the pie chart.
Number of female students in G: $$ \text{Number of female students in G} = 15640 $$ The formula for the central angle is: $$ \text{Angle} = \left( \frac{\text{Number of students in town}}{\text{Total students}} \right) \times 360^{\circ} $$ Calculating for town G: $$ \text{Angle for G} = \left( \frac{15640}{68000} \right) \times 360^{\circ} \approx 82.4^{\circ} $$
- Average female management students in towns A, B, E, and F
Finding the total female students in towns A, B, E, and F.
From the pie chart:
- A: $15%$ of 68,000
- B: $9%$ of 68,000
- E: $14%$ of 68,000
- F: $8%$ of 68,000
Calculating each:
- For A: $$ \text{A} = 0.15 \times 68000 = 10200 $$
- For B: $$ \text{B} = 0.09 \times 68000 = 6120 $$
- For E: $$ \text{E} = 0.14 \times 68000 = 9520 $$
- For F: $$ \text{F} = 0.08 \times 68000 = 5440 $$
Total for towns A, B, E, and F:
$$ \text{Total females} = 10200 + 6120 + 9520 + 5440 = 31880 $$
Average:
$$ \text{Average} = \frac{31880}{4} = 7970 $$
-
The absolute difference is $19080$.
-
The central angle for town G (females) is $82.4^{\circ}$.
-
The average number of female management students in towns A, B, E, and F together is $7970$.
More Information
The management students' distribution is analyzed to find key statistics such as differences, angles for representation, and averages. This aids in understanding demographics in an academic context.
Tips
- Forgetting to convert percentages to decimal before multiplication.
- Not taking the absolute value when calculating differences.
- Miscalculating totals by not adding all relevant components.
AI-generated content may contain errors. Please verify critical information