What is the angle of inclination θ?
Understand the Problem
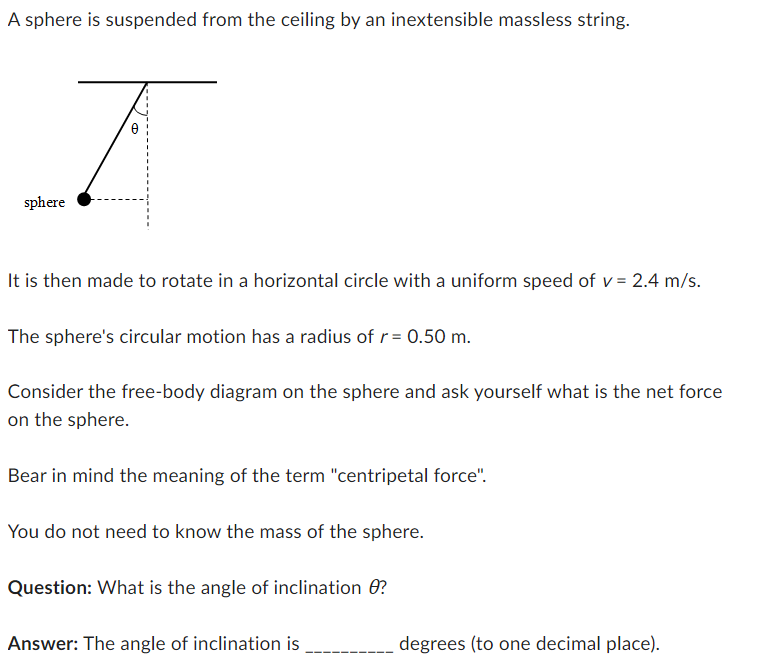
The question is asking for the angle of inclination θ of a sphere suspended by a string as it rotates in a horizontal circle with a given speed. To find this angle, one would consider the forces acting on the sphere and utilize the centripetal force concept.
Answer
The angle of inclination is approximately $50.1$ degrees.
Answer for screen readers
The angle of inclination is approximately $50.1$ degrees.
Steps to Solve
-
Identify forces acting on the sphere
The sphere has two main forces acting on it: the tension ($T$) in the string and the gravitational force ($mg$). The tension can be broken into two components: a vertical component ($T_y$) and a horizontal component ($T_x$). -
Set up the equations for vertical and horizontal components
In the vertical direction, the tension's vertical component balances the gravitational force: $$ T_y = mg $$
In the horizontal direction, the tension's horizontal component provides the necessary centripetal force for circular motion: $$ T_x = \frac{mv^2}{r} $$ -
Use trigonometric relationships
The components of tension can be related to the angle of inclination $\theta$:
- For vertical: $T_y = T \cos(\theta)$
- For horizontal: $T_x = T \sin(\theta)$
-
Combine the equations
From the vertical component: $$ T \cos(\theta) = mg $$
From the horizontal component: $$ T \sin(\theta) = \frac{mv^2}{r} $$
Dividing the second equation by the first gives: $$ \frac{T \sin(\theta)}{T \cos(\theta)} = \frac{mv^2/r}{mg} $$
This simplifies to: $$ \tan(\theta) = \frac{v^2}{rg} $$ -
Substitute known values
We know:
- $v = 2.4 , \text{m/s}$
- $r = 0.50 , \text{m}$
- $g \approx 9.81 , \text{m/s}^2$
Now substitute the values into the equation: $$ \tan(\theta) = \frac{(2.4 , \text{m/s})^2}{(0.50 , \text{m})(9.81 , \text{m/s}^2)} $$
-
Calculate $\tan(\theta)$ and find $\theta$
Calculate the left-hand side: $$ \tan(\theta) = \frac{5.76}{4.905} \approx 1.173 $$
Now find $\theta$ using the arctangent: $$ \theta = \tan^{-1}(1.173) $$
Calculating that gives $\theta \approx 50.1^\circ$.
The angle of inclination is approximately $50.1$ degrees.
More Information
This problem illustrates the application of forces in circular motion and how tension in a string can create components that balance gravitational force and provide centripetal force. The angle of inclination depends on the balance of these forces and does not require the mass of the sphere.
Tips
- Forgetting to break the tension into its components or mixing them up.
- Not using the correct values for gravitational acceleration or the radius in calculations.
- Confusing centripetal force with other types of forces.
AI-generated content may contain errors. Please verify critical information