What does the table of compound interest represent for different rates and time periods?
Understand the Problem
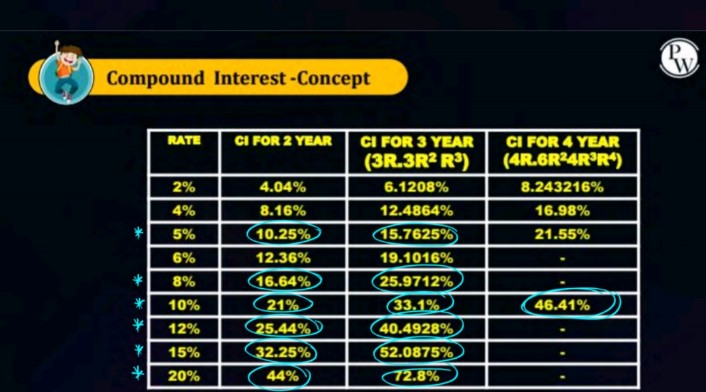
The question appears to be referencing a table showing the compound interest rates for different periods based on various annual interest rates. The user is likely asking for clarification about the table's content or a specific calculation related to compound interest.
Answer
The compound interest for a 10% rate over 3 years is 33.1%.
Answer for screen readers
The compound interest for a 10% rate over 3 years is 33.1%.
Steps to Solve
-
Understanding Compound Interest Compound interest refers to the interest calculated on the initial principal, which also includes all of the accumulated interest from previous periods.
-
Identify the Rate From the table, you can see various interest rates (2%, 4%, 5%, up to 20%) and their respective compound interests for 2, 3, and 4 years.
-
Select a Rate and Year Choose a specific interest rate and period from the table. For example, if we select a rate of 10% for 3 years, we can find that the compound interest is 33.1%.
-
Use the Formula for Compound Interest The formula for calculating compound interest is: $$ A = P(1 + \frac{r}{n})^{nt} $$ Where:
- ( A ) is the amount of money accumulated after n years, including interest.
- ( P ) is the principal amount (the initial sum of money).
- ( r ) is the annual interest rate (decimal).
- ( n ) is the number of times that interest is compounded per year.
- ( t ) is the time the money is invested or borrowed in years.
-
Calculate Compound Interest
If calculating the compound interest yourself:
For example, for a principal ( P = 1000 ), rate ( r = 0.10 ), compounded annually ( (n = 1) ), and time ( t = 3 ): $$ A = 1000(1 + \frac{0.10}{1})^{1 \times 3} = 1000(1.10)^3 \approx 1331 $$ The compound interest would be: $$ CI = A - P = 1331 - 1000 = 331 $$
The compound interest for a 10% rate over 3 years is 33.1%.
More Information
Compound interest generates growth on both your initial investment and any previous interest earned, leading to exponential growth over time.
Tips
- Forgetting to convert the interest rate from a percentage to a decimal when using formulas.
- Not properly applying the formula for compound interest, particularly regarding compounding frequency.
AI-generated content may contain errors. Please verify critical information