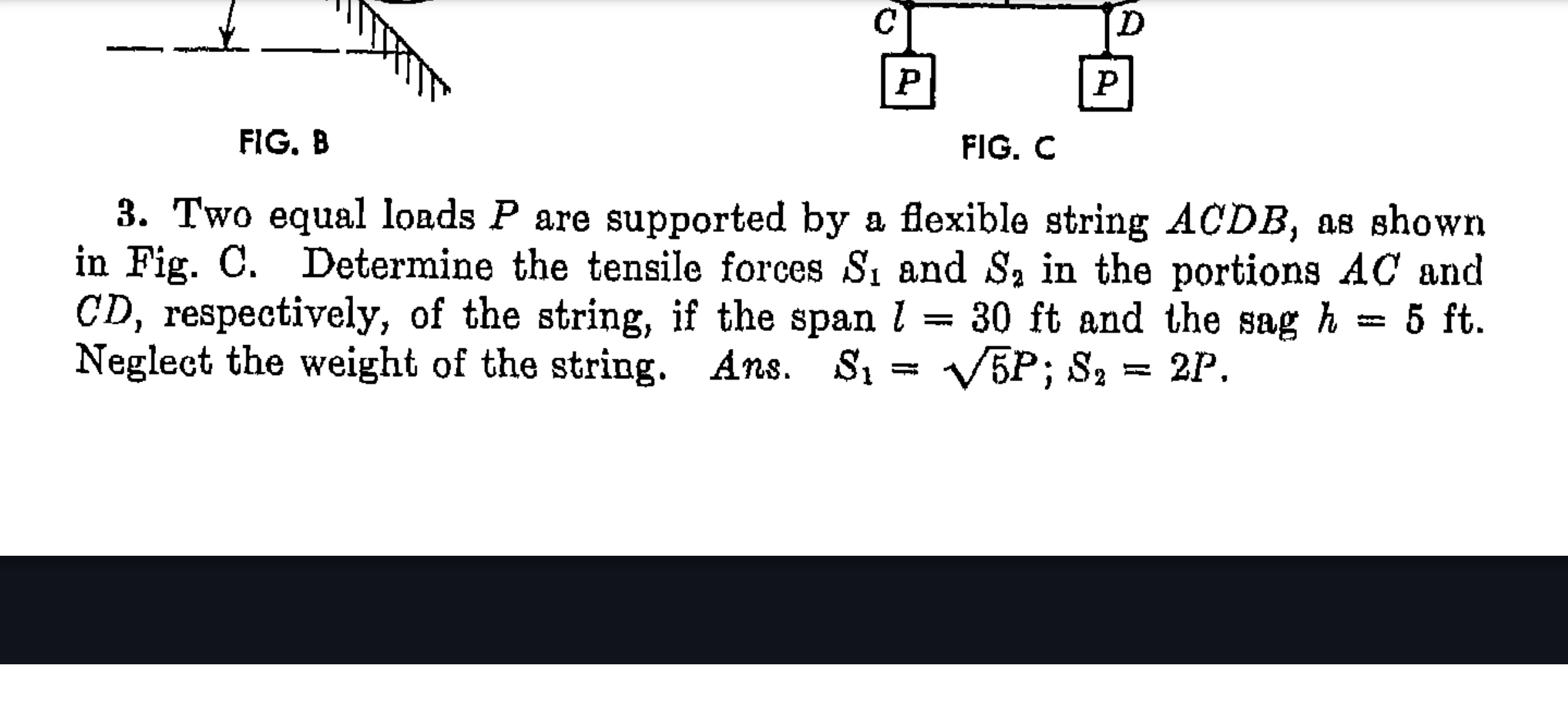
Two equal loads P are supported by a flexible string ACDB. Determine the tensile forces S1 and S2 in the portions AC and CD, respectively, of the string, if the span l = 30 ft and... Two equal loads P are supported by a flexible string ACDB. Determine the tensile forces S1 and S2 in the portions AC and CD, respectively, of the string, if the span l = 30 ft and the sag h = 5 ft. Neglect the weight of the string.
Understand the Problem
The question is asking to determine the tensile forces S1 and S2 in a string supporting two equal loads. It provides specific parameters for the length and sag of the string, indicating that the weight of the string itself can be neglected. The goal is to calculate the forces based on the provided information.
Answer
$$ S_1 = \sqrt{5} P, \, S_2 = 2P $$
Answer for screen readers
The tensile forces are: $$ S_1 = \sqrt{5} P \quad \text{and} \quad S_2 = 2P $$
Steps to Solve
-
Identify the Parameters Given parameters are the span ( l = 30 , \text{ft} ) and the sag ( h = 5 , \text{ft} ).
-
Calculate the Horizontal Component of Force Using the right triangle formed by the span and sag, the horizontal component of the tension can be calculated. The length of each side of the span is ( \frac{l}{2} ).
The horizontal component ( H ) can be calculated using: $$ H = \frac{l}{2} \frac{h}{\sqrt{(\frac{l}{2})^2 + h^2}} $$
-
Determine the Tension Forces The vertical component of force for each load ( P ) is equal to the tension times the sine of the angle ( \theta ) formed by the tension: $$ P = S_1 \sin(\theta) $$
From the right triangle, we can find ( \sin(\theta) ) using: $$ \sin(\theta) = \frac{h}{\sqrt{(\frac{l}{2})^2 + h^2}} $$
Hence for ( S_1 ): $$ S_1 = \frac{P}{\sin(\theta)} $$
-
Calculate ( S_1 ) Substituting ( \sin(\theta) ) into the equation for ( S_1 ): $$ S_1 = P \cdot \frac{\sqrt{(\frac{l}{2})^2 + h^2}}{h} $$
Plugging in the values: $$ S_1 = P \cdot \frac{\sqrt{(15)^2 + (5)^2}}{5} $$ Which simplifies to: $$ S_1 = P \cdot \sqrt{5} $$
-
Calculate ( S_2 ) Since ( S_2 ) is simply twice the vertical load as it directly supports both loads: $$ S_2 = 2P $$
The tensile forces are: $$ S_1 = \sqrt{5} P \quad \text{and} \quad S_2 = 2P $$
More Information
The calculated tensile forces ( S_1 ) and ( S_2 ) represent the tension in the segments AC and CD of the string, respectively. This demonstrates how the sag and span of a flexible string affect the tensile forces when supporting loads.
Tips
- Neglecting to calculate the horizontal component properly can lead to incorrect tension values.
- Misidentifying the angle ( \theta ) formed by the tension and the horizontal may also result in errors.
- Forgetting to apply the trigonometric concepts correctly can lead to miscalculations.
AI-generated content may contain errors. Please verify critical information