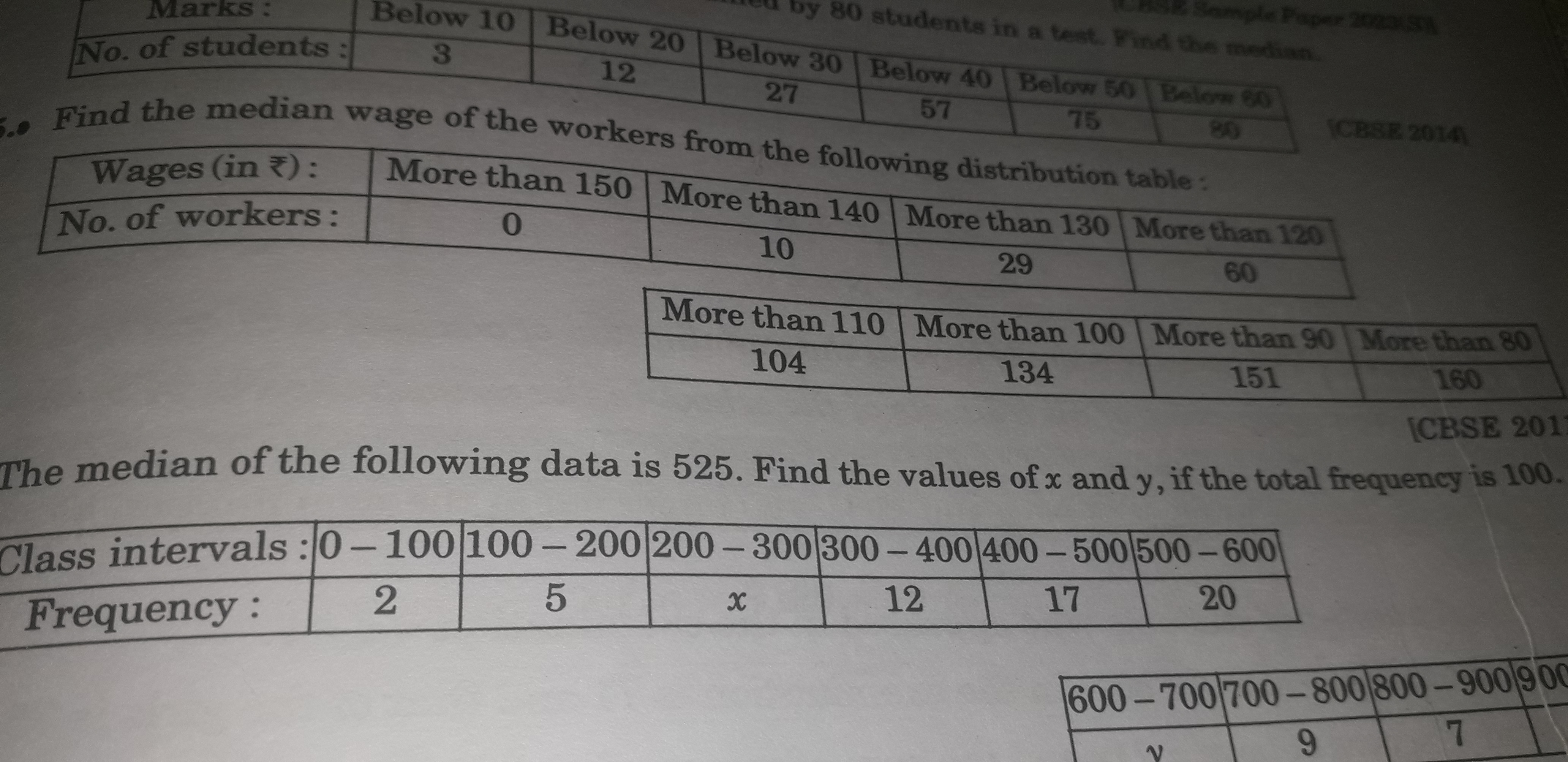
The median of the following data is 525. Find the values of x and y, if the total frequency is 100.

Steps to Solve

1. Determine total frequency equation

Given that the total frequency is 100, we can set up the equation:

$$ 2 + 5 + x + 12 + 17 + 20 + y = 100 $$

2. Simplify and solve for x + y

Calculating the sum of known frequencies:

$$ 2 + 5 + 12 + 17 + 20 = 56 $$

Now, we have:

$$ 56 + x + y = 100 $$

This simplifies to:

$$ x + y = 44 $$

3. Determine the median class interval

To find the median, we need to first determine the cumulative frequency. We need to find the median position:

$$ \frac{N}{2} = \frac{100}{2} = 50 $$

4. Calculate cumulative frequencies

Calculate cumulative frequency for each class:

• For 0-100: 2
• For 100-200: 2 + 5 = 7
• For 200-300: 7 + x = 7 + x
• For 300-400: 7 + x + 12 = 19 + x
• For 400-500: 19 + x + 17 = 36 + x
• For 500-600: 36 + x + 20 = 56 + x
• Cumulative frequency for 600-700: $56 + x + y$

5. Identify median class

The median class is the first class where the cumulative frequency is at least 50.

This means we need $56 + x \geq 50$:

• If $x < 44$, the cumulative frequency for the class 500-600 will be the median class.

6. Use median calculation to find x

The median class is 500-600. Now we need to find a suitable value for $x$ that satisfies $x + y = 44$ and keeps a cumulative frequency of at least 50.

Using the median formula: $$ \text{Median} = L + \left(\frac{\frac{N}{2} - CF}{f}\right) \times c $$

Where:

• $L = 500$ (lower limit of median class),
• $CF = 36 + x$ (cumulative frequency before median class),
• $f = 20$ (frequency of the median class),
• $c = 100$ (class width).

7. Set up median equation

Set the median equation as follows:

$$ 525 = 500 + \left(\frac{50 - (36 + x)}{20}\right) \times 100 $$

Now simplify and solve for $x$.

8. Solve for y using x value

After calculating $x$, use the equation $x + y = 44$ to find $y$.