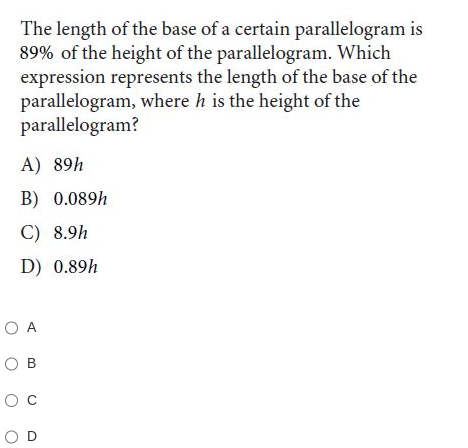
The length of the base of a certain parallelogram is 89% of the height of the parallelogram. Which expression represents the length of the base of the parallelogram, where h is the... The length of the base of a certain parallelogram is 89% of the height of the parallelogram. Which expression represents the length of the base of the parallelogram, where h is the height of the parallelogram?
Understand the Problem
The question is asking for the expression that represents the length of the base of a parallelogram, given that it is 89% of the height. We need to translate this percentage into a mathematical expression using the variable h, which represents the height.
Answer
The expression for the length of the base is $0.89h$.
Answer for screen readers
The expression that represents the length of the base of the parallelogram is $0.89h$.
Steps to Solve
- Identify the Given Information
The problem states that the base of the parallelogram is 89% of the height. Let the height be represented by the variable $h$.
- Convert Percentage to Decimal
To express 89% as a decimal, divide by 100: $$ 0.89 = \frac{89}{100} $$
- Write the Expression for the Base
The length of the base (we'll call it $b$) can be expressed as: $$ b = 0.89 \cdot h $$
- Select the Correct Option
Now, check which of the given options represents this expression:
- A) $89h$
- B) $0.089h$
- C) $8.9h$
- D) $0.89h$
The correct expression is $0.89h$, which corresponds to option D.
The expression that represents the length of the base of the parallelogram is $0.89h$.
More Information
In this problem, converting percentages to decimals is a common technique in algebra. The conversion allows for easier manipulation when creating expressions based on relationships described in the problem.
Tips
- Confusing percentage with decimal: Some might mistakenly interpret 89% as 89 rather than $0.89$.
- Misplacing the decimal or removing it entirely, leading to expressions like $89h$ or $8.9h$.
AI-generated content may contain errors. Please verify critical information