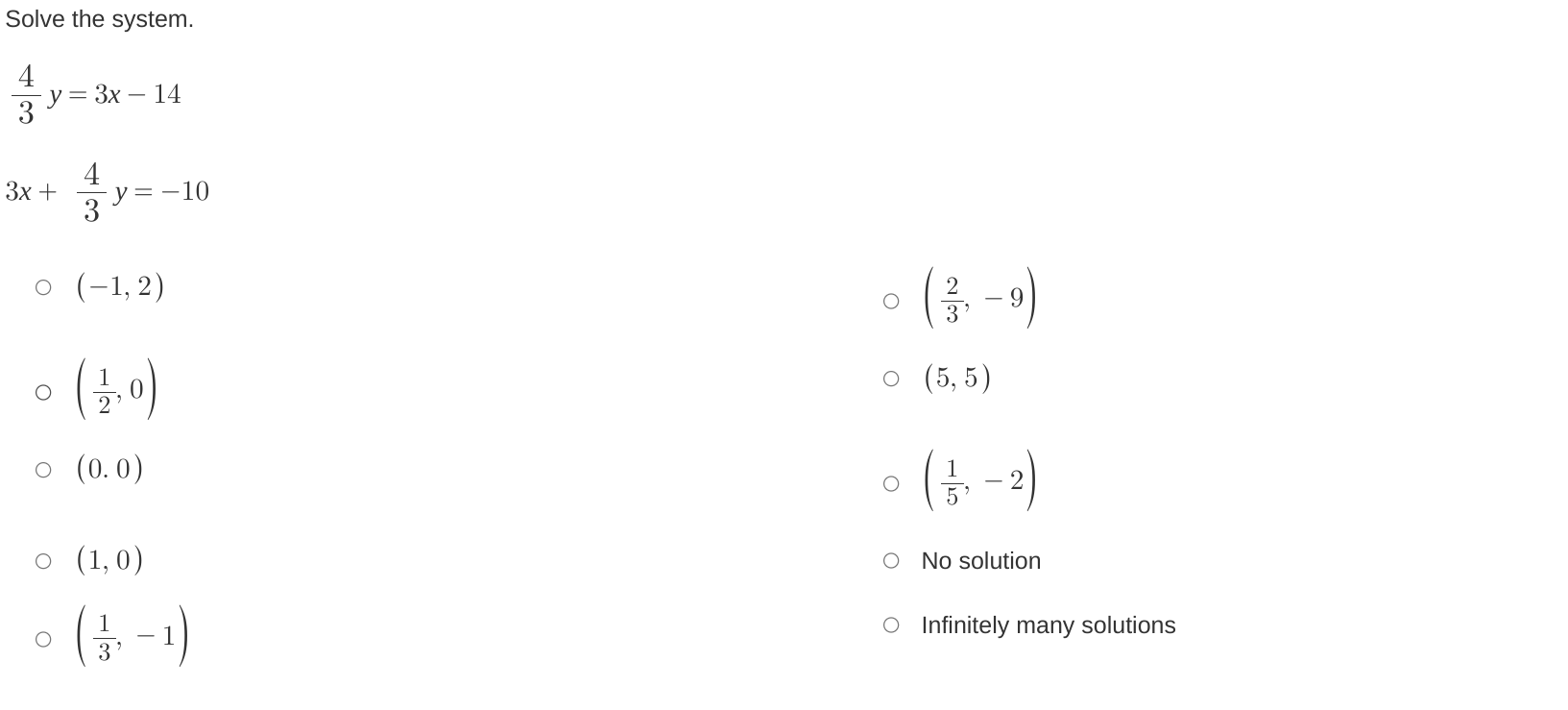
Solve the system: 4/3y = 3x - 14 and 3x + 4/3y = -10.

Steps to Solve

1. Rewrite the Equations First, we rewrite the given equations for clarity:

• Equation 1: $$ \frac{4}{3}y = 3x - 14 $$
• Equation 2: $$ 3x + \frac{4}{3}y = -10 $$

2. Isolate y in the First Equation To isolate $y$, multiply both sides of the first equation by $\frac{3}{4}$: $$ y = \frac{3}{4}(3x - 14) $$ Simplifying gives: $$ y = \frac{9}{4}x - \frac{42}{4} $$ or $$ y = \frac{9}{4}x - \frac{21}{2} $$

3. Substitute y in the Second Equation Next, substitute this expression for $y$ into the second equation: $$ 3x + \frac{4}{3} \left(\frac{9}{4}x - \frac{21}{2}\right) = -10 $$

4. Clear the Fraction Multiply through by 3 to eliminate the fractions: $$ 9x + 4\left(\frac{9}{4}x - \frac{21}{2}\right) = -30 $$

5. Distribute and Combine Terms This simplifies to: $$ 9x + 9x - 42 = -30 $$ Combine like terms: $$ 18x - 42 = -30 $$

6. Solve for x Add 42 to both sides: $$ 18x = 12 $$ Now divide by 18: $$ x = \frac{12}{18} = \frac{2}{3} $$

7. Find y using x Substituting $x = \frac{2}{3}$ back into the equation for $y$: $$ y = \frac{9}{4}\left(\frac{2}{3}\right) - \frac{21}{2} $$ This simplifies to: $$ y = \frac{6}{4} - \frac{21}{2} = \frac{3}{2} - \frac{21}{2} = \frac{-18}{2} = -9 $$

8. Final Solution Thus, the solution to the system is: $$ \left(\frac{2}{3}, -9\right) $$