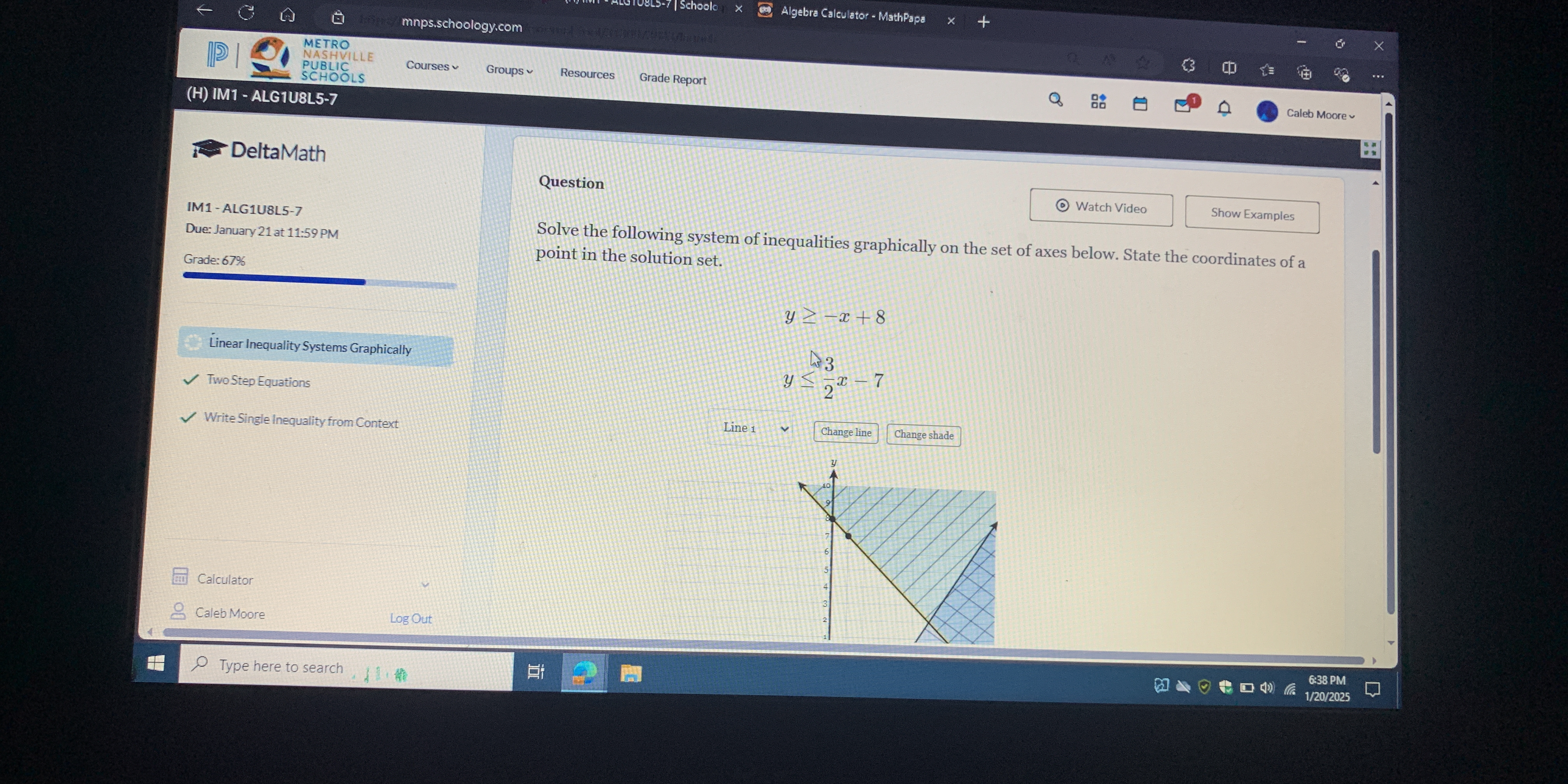
Solve the following system of inequalities graphically on the set of axes below. State the coordinates of a point in the solution set.
Understand the Problem
The question is asking to solve a system of inequalities graphically. It requires plotting the inequalities on a graph and identifying the solution set, as well as stating the coordinates of at least one point that lies within that solution set.
Answer
The point in the solution set is \( (-2, 5) \).
Answer for screen readers
The coordinates of a point in the solution set are ( (-2, 5) ).
Steps to Solve
- Identify the inequalities The given inequalities are:
- ( y \geq x + 8 )
- ( y \leq \frac{3}{2}x - 7 )
- Graph the first inequality To graph ( y \geq x + 8 ):
- Start with the line ( y = x + 8 ).
- The y-intercept is ( 8 ) (the point where the line crosses the y-axis).
- The slope is ( 1 ), meaning you rise 1 unit and run 1 unit to the right.
- Since it’s a "greater than or equal to" inequality, draw a solid line and shade above the line.
- Graph the second inequality To graph ( y \leq \frac{3}{2}x - 7 ):
- Start with the line ( y = \frac{3}{2}x - 7 ).
- The y-intercept is ( -7 ) (the point where the line crosses the y-axis).
- The slope is ( \frac{3}{2} ), meaning you rise 3 units and run 2 units to the right.
- Since it’s a "less than or equal to" inequality, draw a solid line and shade below the line.
- Identify the solution set The solution set is the overlapping shaded region of the two inequalities. To confirm, choose a test point, such as the origin ( (0, 0) ):
- For ( y \geq x + 8 ): ( 0 \geq 0 + 8 ) (false)
- For ( y \leq \frac{3}{2}x - 7 ): ( 0 \leq \frac{3}{2}(0) - 7 ) (true)
Since the origin is not in the solution set, check other points within the shaded region for confirmation.
- Select a point from the solution set Choose a point in the overlapping region; for example, ( (-2, 5) ):
- Check ( y \geq x + 8 ): ( 5 \geq -2 + 8 ) (true)
- Check ( y \leq \frac{3}{2}x - 7 ): ( 5 \leq \frac{3}{2}(-2) - 7 ) (true)
The coordinates of a point in the solution set are ( (-2, 5) ).
More Information
Graphical solutions for systems of inequalities help visualize the range of solutions and can illustrate relationships between different functions. Choosing points within the intersection of shaded regions confirms if they satisfy the system.
Tips
- Forgetting to shade the correct region above or below the line according to the inequality.
- Using dashed lines instead of solid lines for inclusive inequalities (≥ or ≤).
- Not verifying points within the shaded regions to confirm they satisfy both inequalities.
AI-generated content may contain errors. Please verify critical information