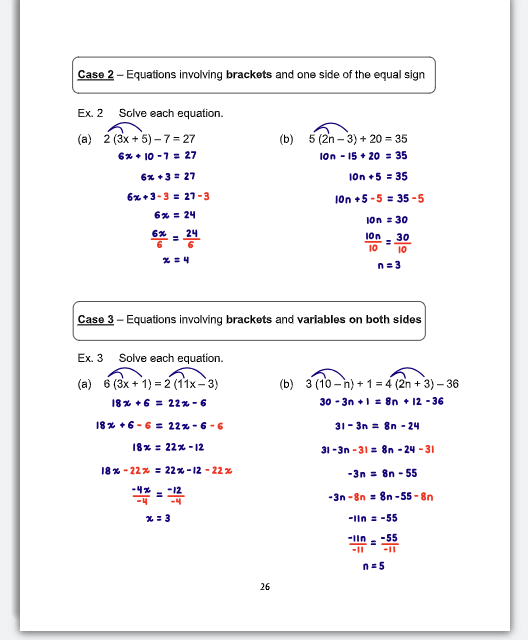
Solve the equations: (a) 2(3x + 5) - 7 = 27 and (b) 5(2n - 3) + 20 = 35. Also solve for (a) 6(3(x + 1)) = 2(11(x - 3)) and (b) 3(10 - n) + 1 = 4(2n + 3) - 36.
Understand the Problem
The question consists of several algebraic equations that require solving for the unknown variables. The problems involve both brackets and variables, and they are structured into two cases. The objective is to simplify and isolate the variable on one side of the equation to find its value.
Answer
$x = 4$, $n = 3$, $x = 21$, $n = 5$
Answer for screen readers
- For Equation (a) from Case 2: $x = 4$
- For Equation (b) from Case 2: $n = 3$
- For Equation (a) from Case 3: $x = 21$
- For Equation (b) from Case 3: $n = 5$
Steps to Solve
-
Solve Equation (a) from Case 2 First, distribute the 2 to the brackets: $$ 2(3x + 5) - 7 = 27 $$ This becomes: $$ 6x + 10 - 7 = 27 $$ Now simplify: $$ 6x + 3 = 27 $$ Next, isolate $x$ by subtracting 3 from both sides: $$ 6x = 24 $$ Now, divide by 6: $$ x = 4 $$
-
Solve Equation (b) from Case 2 Start with the equation: $$ 5(2n - 3) + 20 = 35 $$ Distribute the 5: $$ 10n - 15 + 20 = 35 $$ Now simplify: $$ 10n + 5 = 35 $$ Subtract 5 from both sides: $$ 10n = 30 $$ Finally, divide by 10: $$ n = 3 $$
-
Solve Equation (a) from Case 3 The equation is: $$ 6(3(x+1)) = 2(11(x-3)) $$ Distributing yields: $$ 18(x + 1) = 22(x - 3) $$ Now distribute on both sides: $$ 18x + 18 = 22x - 66 $$ Rearranging gives: $$ 18 + 66 = 22x - 18x $$ So: $$ 84 = 4x $$ Finally, divide by 4: $$ x = 21 $$
-
Solve Equation (b) from Case 3 Start with: $$ 3(10 - n) + 1 = 4(2n + 3) - 36 $$ Distributing: $$ 30 - 3n + 1 = 8n + 12 - 36 $$ Simplifying yields: $$ 31 - 3n = 8n - 24 $$ Rearranging gives: $$ 31 + 24 = 8n + 3n $$ So: $$ 55 = 11n $$ Dividing by 11 results in: $$ n = 5 $$
- For Equation (a) from Case 2: $x = 4$
- For Equation (b) from Case 2: $n = 3$
- For Equation (a) from Case 3: $x = 21$
- For Equation (b) from Case 3: $n = 5$
More Information
These equations illustrate how to solve simple linear equations by isolating the variable through distribution and simplification. The techniques used include the distributive property, combining like terms, and basic algebraic operations.
Tips
- Misapplying the distributive property: Always ensure to distribute correctly; for example, forgetting to distribute to both terms inside the bracket can lead to wrong equations.
- Neglecting to combine like terms: After distribution, don't forget to combine any like terms before isolating the variable.
- Switching operation signs: Ensure that when moving terms across the equals sign, the operation changes accordingly (e.g., from positive to negative).
AI-generated content may contain errors. Please verify critical information