Show that a geometric sequence with first term 3 and seventh term 1/243 has two possible sums to infinity and find them.
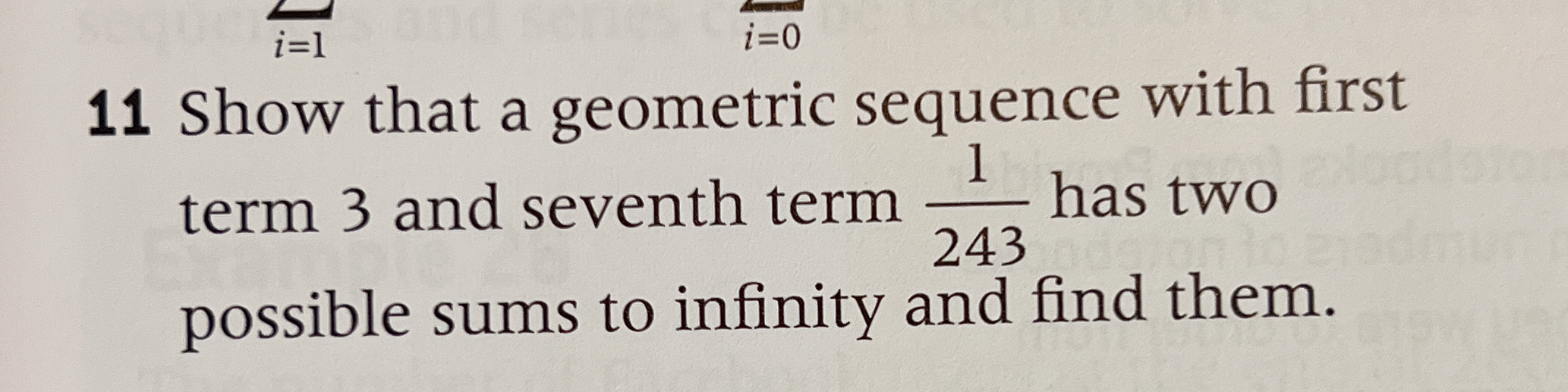
Understand the Problem
The question is asking to demonstrate that a geometric sequence with specific first and seventh terms has two possible sums to infinity and to determine what those sums are.
Answer
The sums are $S_1 = \frac{9}{2}$ and $S_2 = \frac{9}{4}$.
Answer for screen readers
The two possible sums to infinity are $S_1 = \frac{9}{2}$ and $S_2 = \frac{9}{4}$.
Steps to Solve
- Define the geometric sequence terms
In a geometric sequence, the $n$-th term can be expressed as $$ a_n = a \cdot r^{n-1} $$ where $a$ is the first term and $r$ is the common ratio.
Given that the first term, $a_1 = 3$, we have: $$ a = 3 $$
- Express the seventh term
The seventh term is given as $$ a_7 = a \cdot r^{6} = \frac{1}{243} $$
Substituting the first term into this equation: $$ 3 \cdot r^{6} = \frac{1}{243} $$
- Solve for $r^{6}$
To isolate $r^{6}$, divide both sides by 3: $$ r^{6} = \frac{1}{729} $$
This simplifies to: $$ r^{6} = (3^{-6}) $$ $$ \implies r = 3^{-1} \text{ or } r = -3^{-1} $$
This gives: $$ r = \frac{1}{3} \text{ or } r = -\frac{1}{3} $$
- Calculate the sums to infinity
The sum to infinity of a geometric series is given by: $$ S = \frac{a}{1 - r} $$
For the first case when $r = \frac{1}{3}$: $$ S_1 = \frac{3}{1 - \frac{1}{3}} = \frac{3}{\frac{2}{3}} = \frac{3 \cdot 3}{2} = \frac{9}{2} = 4.5 $$
For the second case when $r = -\frac{1}{3}$: $$ S_2 = \frac{3}{1 - (-\frac{1}{3})} = \frac{3}{1 + \frac{1}{3}} = \frac{3}{\frac{4}{3}} = \frac{3 \cdot 3}{4} = \frac{9}{4} = 2.25 $$
The two possible sums to infinity are $S_1 = \frac{9}{2}$ and $S_2 = \frac{9}{4}$.
More Information
In a geometric sequence, if the absolute value of the common ratio is less than 1, the series converges, allowing a finite sum. In this problem, the first term is 3, and the flexibility with positive and negative ratios leads to two valid sums.
Tips
- Confusing the common ratio $r$; ensure both positive and negative possibilities are considered.
- Miscalculating the sum formula for geometric series; remember to verify that $|r| < 1$ for convergence.
AI-generated content may contain errors. Please verify critical information