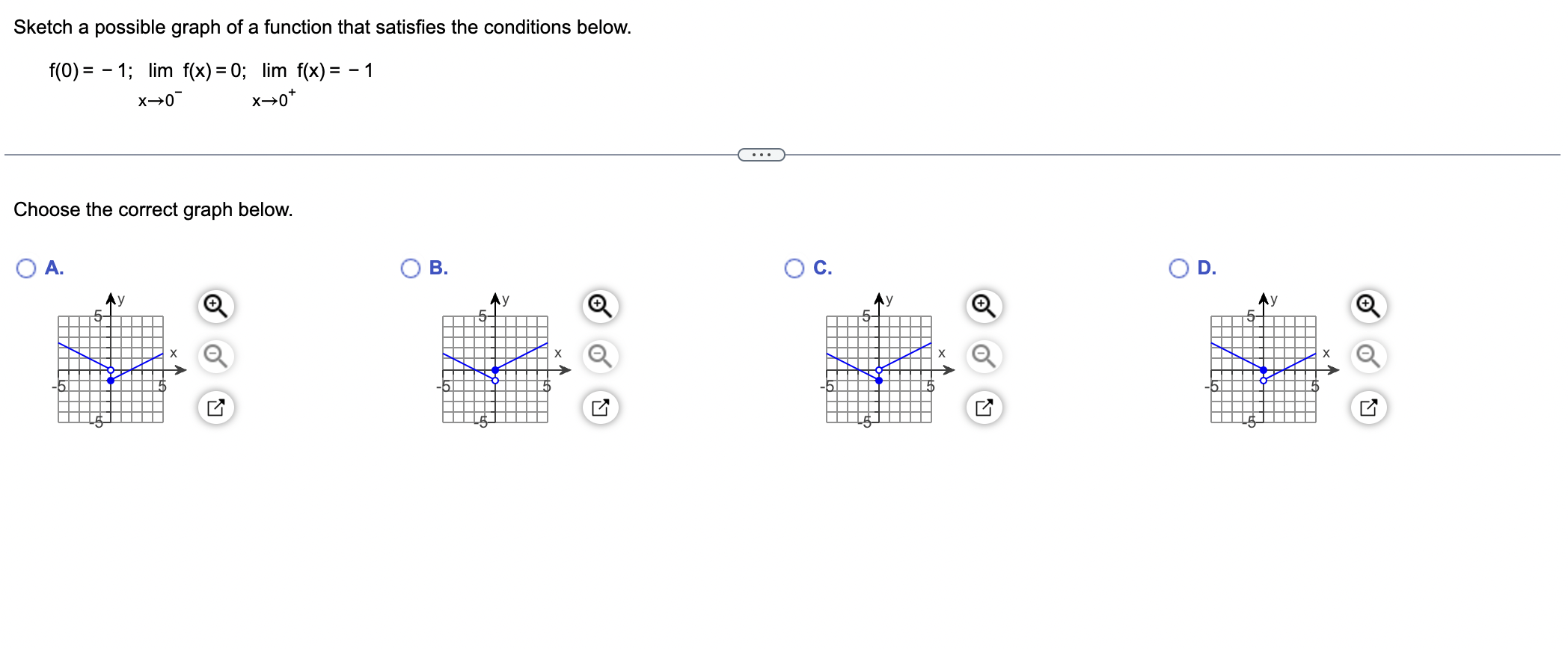
Sketch a possible graph of a function that satisfies the conditions below. f(0) = -1; lim f(x) = 0; lim f(x) = -1.

Steps to Solve

1. Identify the function values at specific points

We know from the problem that

• $f(0) = -1$
• $\lim_{{x \to 0^-}} f(x) = 0$
• $\lim_{{x \to 0^+}} f(x) = -1$

This indicates the function has a hole at $x = 0$ and approaches two different values from the left and right.

2. Analyze the left-hand limit

The left-hand limit $\lim_{{x \to 0^-}} f(x) = 0$ means that as $x$ approaches 0 from the left, $f(x)$ should approach 0. Therefore, for values of $x < 0$, the function should be heading towards the point (just before 0 on the y-axis, which is the point (0,0) but not including it).

3. Analyze the right-hand limit

The right-hand limit $\lim_{{x \to 0^+}} f(x) = -1$ indicates that as $x$ approaches 0 from the right, the function approaches -1. So for values of $x > 0$, the graph will be near the point (0, -1).

4. Sketch key points

• At $x = 0$, place a point for $f(0) = -1$. This should be a solid dot at (0, -1).
• As $x$ approaches 0 from the left, the graph must go up towards (0, 0) but without actually touching it (indicating a hole).
• As $x$ approaches 0 from the right, the graph should start from (0, -1) downwards (solid point).

5. Choose the graph

Compare the drawn characteristics to the provided graph options A, B, C, and D. Choose the one that shows the described behavior.