Rewrite the equation y = x² - 6x - 5 into vertex (graphing) form.
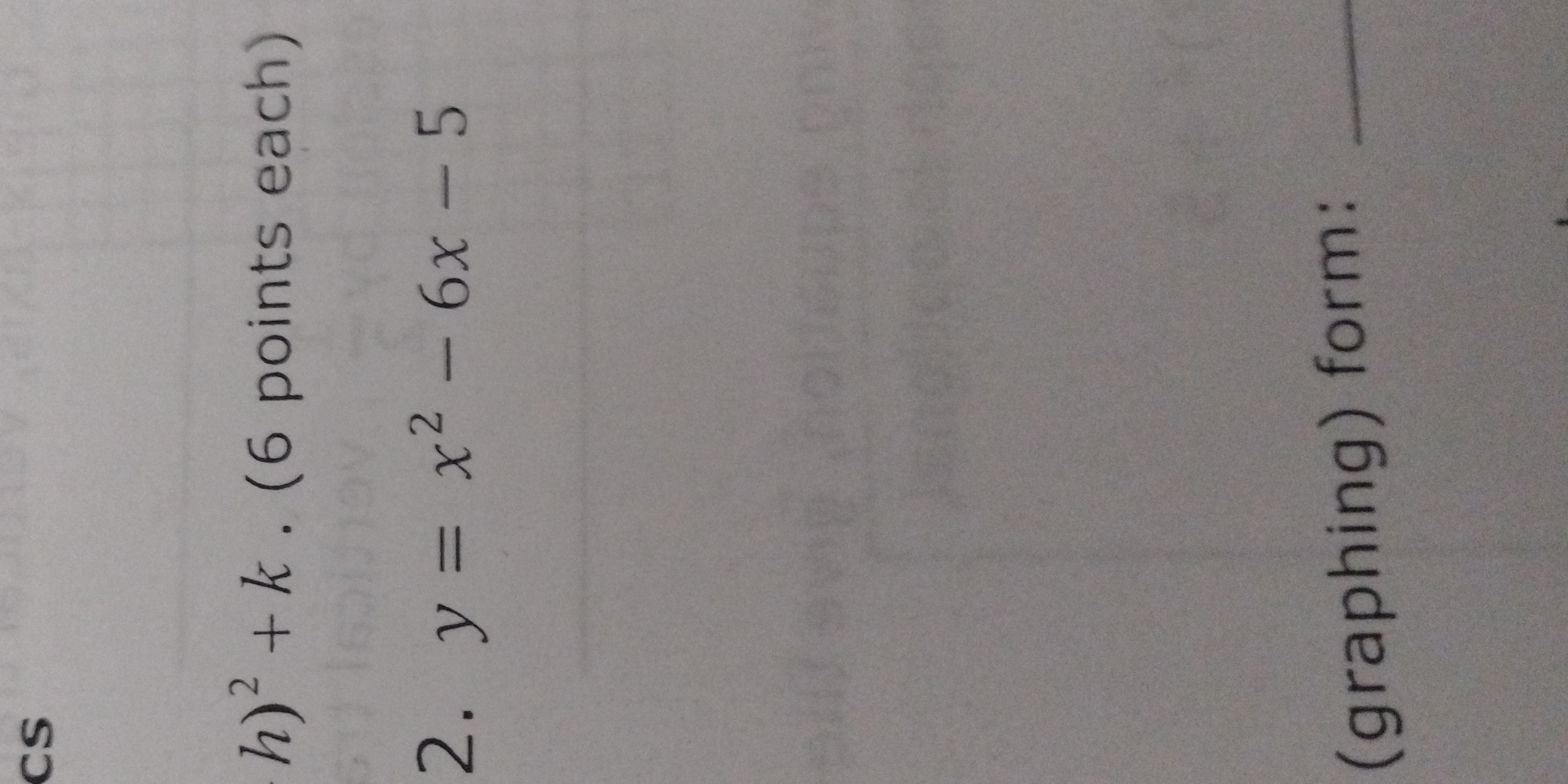
Understand the Problem
The question is asking to rewrite the given quadratic equation, which is y = x² - 6x - 5, into a vertex (graphing) form, presumably to identify its vertex and potentially graph it.
Answer
The vertex form of the equation is \( y = (x - 3)^2 - 14 \).
Answer for screen readers
The vertex form of the equation is ( y = (x - 3)^2 - 14 ).
Steps to Solve
-
Identify the quadratic equation The given quadratic equation is ( y = x^2 - 6x - 5 ).
-
Complete the square To write it in vertex form, we need to complete the square for the expression ( x^2 - 6x ).
- Start by focusing on ( x^2 - 6x ).
- To complete the square, take half of the coefficient of ( x ) (which is (-6)), square it, and add/subtract it.
$$ \left(\frac{-6}{2}\right)^2 = 9 $$
Therefore, we rewrite the equation as: $$ y = (x^2 - 6x + 9 - 9) - 5 $$
-
Rewrite the equation in vertex form Now, factor the completed square and simplify:
-
Rearranging gives: $$ y = (x - 3)^2 - 9 - 5 $$
-
Combine the constant terms: $$ y = (x - 3)^2 - 14 $$
-
-
Final vertex form The equation in vertex form is:
$$ y = (x - 3)^2 - 14 $$
From this equation, we can identify that the vertex is at the point ( (3, -14) ).
The vertex form of the equation is ( y = (x - 3)^2 - 14 ).
More Information
The vertex of the quadratic function indicates the minimum point of the graph. In this case, the vertex ( (3, -14) ) shows that the graph opens upwards and reaches its lowest point at this coordinate. The vertex form is particularly useful for graphing and understanding the behavior of quadratic functions.
Tips
- A common mistake is to forget to adjust the constant when completing the square. Always remember to add and subtract the same number.
- Another mistake is incorrect factoring of the completed square expression, which can lead to errors in the vertex form.
AI-generated content may contain errors. Please verify critical information