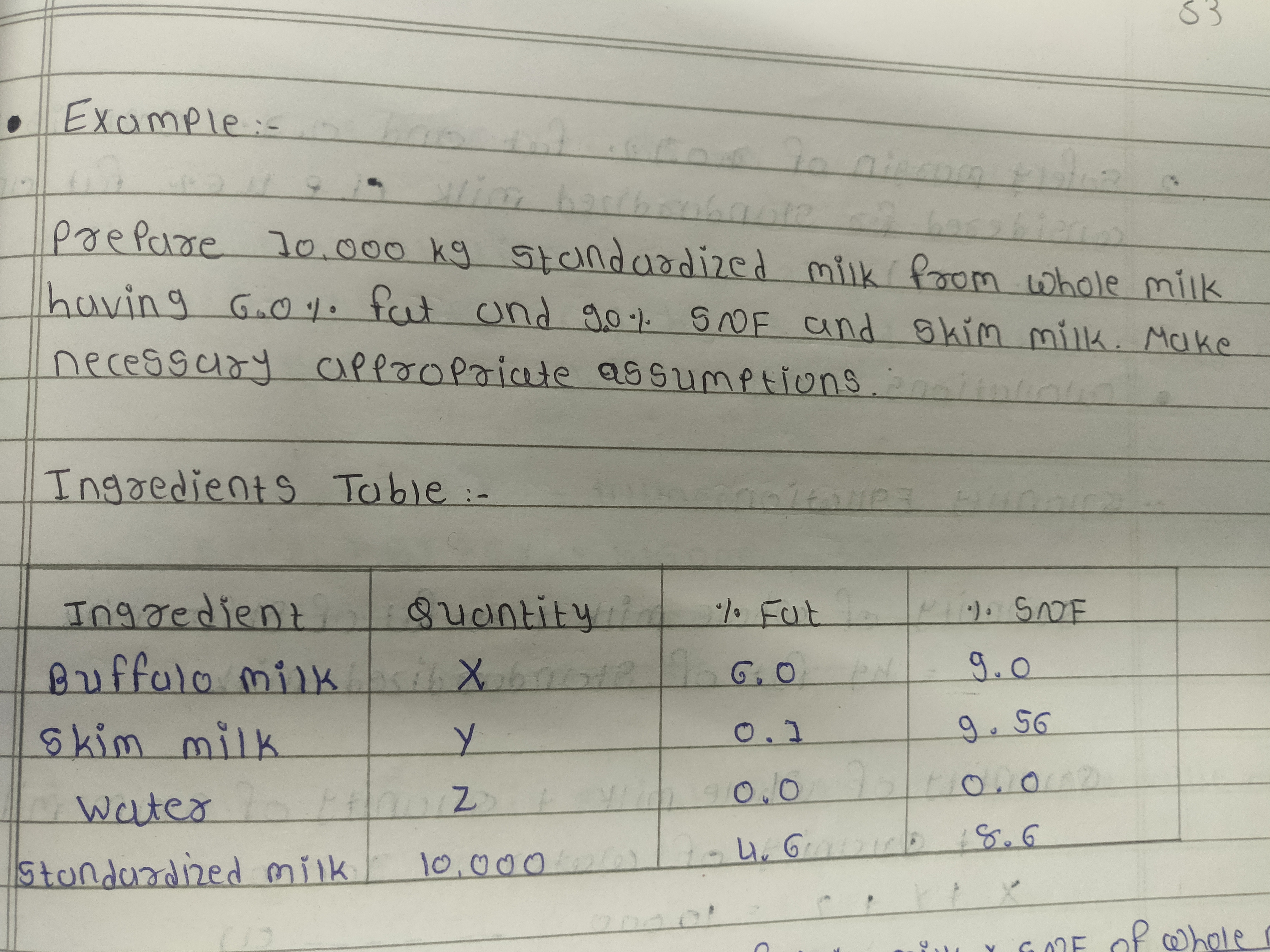
Prepare 10,000 kg standardized milk from whole milk having 6.0% fat and 9.0% SNF and skim milk. Make necessary appropriate assumptions.

Steps to Solve

1. Define the Variables Let:

• ( X ) = quantity of buffalo milk
• ( Y ) = quantity of skim milk
• ( Z ) = quantity of water

We know the total quantity of standardized milk is 10,000 kg. Thus, we have the equation: $$ X + Y + Z = 10,000 $$

2. Set Up the Fat Content Equation Buffalo milk has 6% fat, skim milk has 0.7% fat, and water has 0% fat. The standardized milk needs to have 4.6% fat. The fat contribution from each type of milk is: $$ 0.06X + 0.007Y + 0.00Z = 0.046 \times 10,000 $$

Calculating the right side: $$ 0.046 \times 10,000 = 460 $$

So, the equation becomes: $$ 0.06X + 0.007Y = 460 $$

3. Set Up the SNF Content Equation Buffalo milk has 9% SNF, skim milk has 9.56% SNF, and water has 0% SNF. We need to meet the requirement for standardized milk which is 8.6% SNF. The equation for SNF becomes: $$ 0.09X + 0.0956Y = 0.086 \times 10,000 $$

Calculating the right side: $$ 0.086 \times 10,000 = 860 $$

So, the equation is: $$ 0.09X + 0.0956Y = 860 $$

4. Solve the System of Equations Now we have a system of equations:
5. ( X + Y + Z = 10,000 )
6. ( 0.06X + 0.007Y = 460 )
7. ( 0.09X + 0.0956Y = 860 )

Using the first equation, we can express ( Z ): $$ Z = 10,000 - X - Y $$

We can substitute this in the other equations to solve for ( X ) and ( Y ).

5. Solve for One Variable From equation 2: $$ Y = \frac{460 - 0.06X}{0.007} $$

And substituting ( Y ) into the third equation, we can calculate ( X ).

6. Calculate Remaining Quantities After finding ( X ), substitute back to find ( Y ) and then ( Z ).