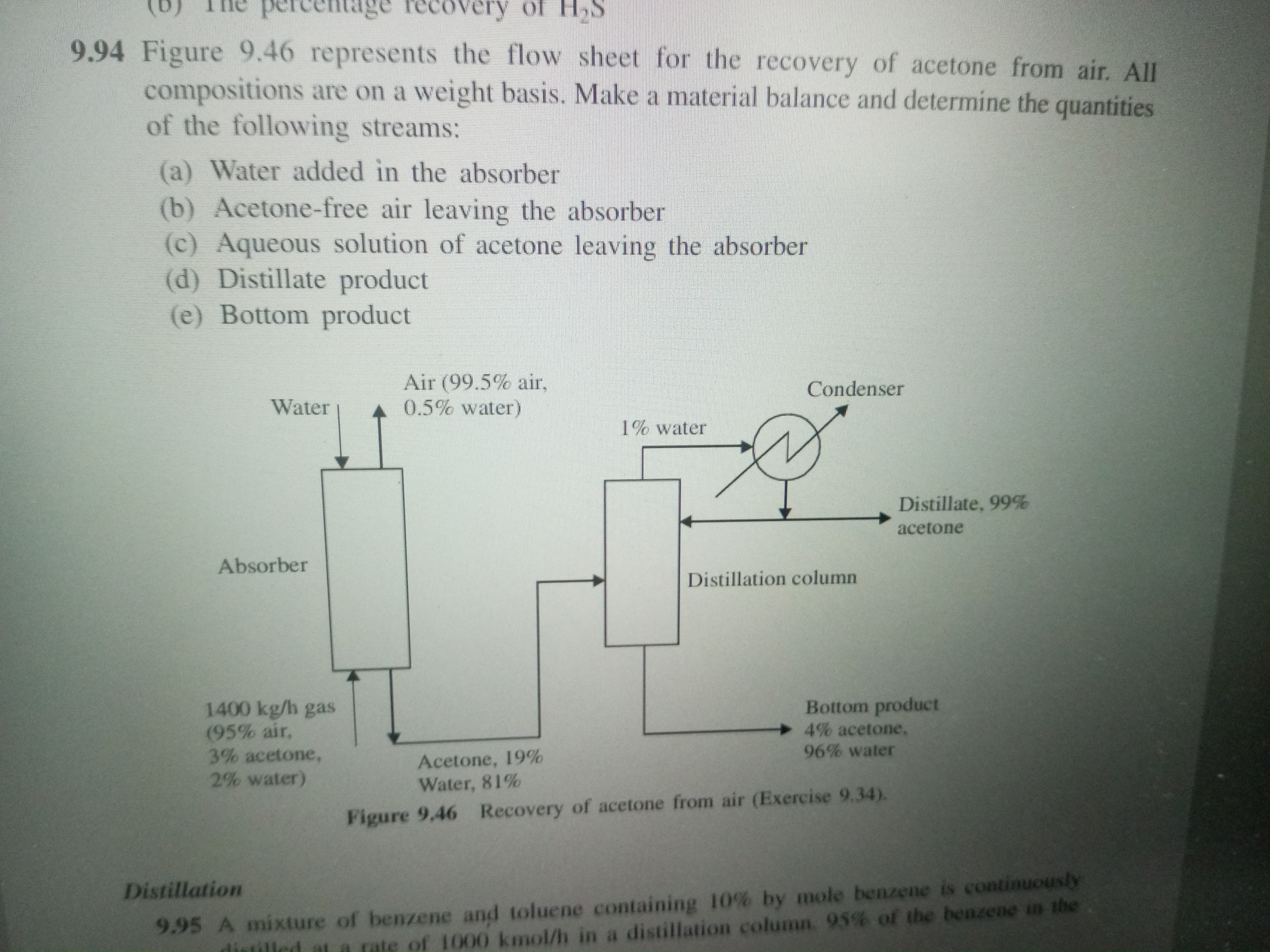
Make a material balance and determine the quantities of the following streams: (a) Water added in the absorber (b) Acetone-free air leaving the absorber (c) Aqueous solution of ace... Make a material balance and determine the quantities of the following streams: (a) Water added in the absorber (b) Acetone-free air leaving the absorber (c) Aqueous solution of acetone leaving the absorber (d) Distillate product (e) Bottom product. Read more

Steps to Solve

1. Understand the Given Data
   We have the following information from the flow sheet:

• The inlet air stream consists of 1400 kg/h with 95% air, 3% acetone, and 2% water.
• The air entering the absorber contains 99.5% air and 0.5% water.
• The composition of the liquid leaving the absorber is 19% acetone and 81% water.

2. Calculate Water Added in the Absorber
   To determine the water added, apply the material balance around the absorber:
   Let $W_a$ be the water added to the absorber, and we assume all the water exits through the absorber.
   The outgoing water can be expressed as:
   $$ W_{out} = 0.005 \times 1400 \text{ kg/h} + W_a $$
   Since the output water is 1% from the liquid leaving the absorber:
   $$ 0.01 \times (W_{out}) = 0.81 \times W_{out} $$

3. Calculate Acetone-Free Air Leaving the Absorber
   To find the acetone-free air leaving the absorber, first calculate the weight of air exiting:
   $$ W_{air, out} = W_{in} - W_{in, acetone} $$
   Where:

• $W_{in} = 1400 \text{ kg/h}$,
• $W_{in, acetone} = 0.03 \times 1400 \text{ kg/h}$.
  Thus, the acetone-free air stream will be:
  $$ W_{air, out} = 1400 \text{ kg/h} - W_{in, acetone} $$

4. Determine Aqueous Solution of Acetone Leaving the Absorber
   The mass of the aqueous solution leaving the absorber can be calculated based on the liquid composition:
   $$ W_{liquid, out} = W_{in, acetone} + W_{a} $$

5. Calculate Distillate Product
   The distillate, which is 99% acetone, can be considered as:
   If $D$ is the mass of distillate:
   $$ D = 0.99 \times \text{Evaporated Acetone} $$
   Where evaporated acetone can be a fraction of the inlet acetone.

6. Calculate Bottom Product
   The bottom product from the distillation is given as containing 4% acetone and 96% water.
   If $B$ is the mass of the bottom product, then:
   $$ B = 0.04B \text{ (for acetone component)} $$

7. Collecting Information
   Bring together the equations to form a complete material balance involving the found variables.