Madison collected a bunch of dimes and quarters for her school fundraiser. She collected a total of 122 coins and a total of $21.50. How many dimes and how many quarters did she co... Madison collected a bunch of dimes and quarters for her school fundraiser. She collected a total of 122 coins and a total of $21.50. How many dimes and how many quarters did she collect?
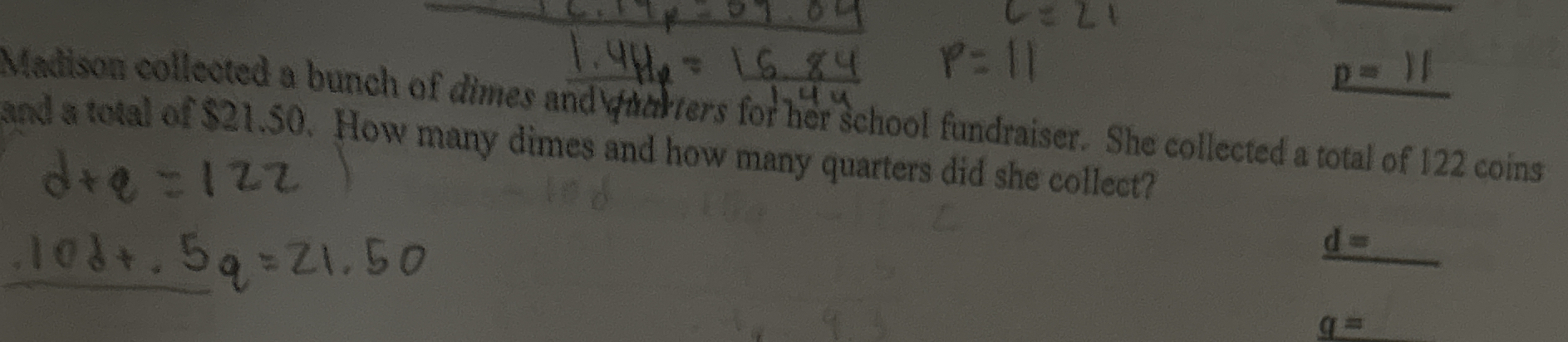
Understand the Problem
The question is asking how many dimes and quarters Madison collected, given that she collected a total of 122 coins and their total value is $21.50. We need to set up a system of equations to find the values.
Answer
60 dimes and 62 quarters.
Answer for screen readers
Madison collected 60 dimes and 62 quarters.
Steps to Solve
-
Define Variables Let $d$ be the number of dimes and $q$ be the number of quarters.
-
Set Up the Equations Based on the information provided:
- The total number of coins is 122: $$ d + q = 122 $$
- The total value of the coins is $21.50. Since dimes are worth $0.10 and quarters are worth $0.25: $$ 0.10d + 0.25q = 21.50 $$
-
Solve the First Equation for One Variable From the first equation, isolate one variable: $$ q = 122 - d $$
-
Substitute Into the Second Equation Substitute $q$ in the second equation: $$ 0.10d + 0.25(122 - d) = 21.50 $$
-
Simplify and Solve the Equation Distribute $0.25$: $$ 0.10d + 30.50 - 0.25d = 21.50 $$
Combine like terms: $$ -0.15d + 30.50 = 21.50 $$
Isolate $d$: $$ -0.15d = 21.50 - 30.50 $$ $$ -0.15d = -9 $$
Divide by $-0.15$: $$ d = \frac{-9}{-0.15} = 60 $$
- Find the Number of Quarters Use the value of $d$ to find $q$: $$ q = 122 - d $$ $$ q = 122 - 60 = 62 $$
Madison collected 60 dimes and 62 quarters.
More Information
Madison's collection of dimes and quarters totals 122 coins with a value of $21.50, which fits the criteria for coin collection problems often encountered in algebra. Each dime adds $0.10 and each quarter adds $0.25 to the total amount.
Tips
- Mixing up the values of the coins in the equations. Ensure to assign the correct values for dimes and quarters.
- Forgetting to substitute properly when solving system equations. Always check each substitution step.
AI-generated content may contain errors. Please verify critical information