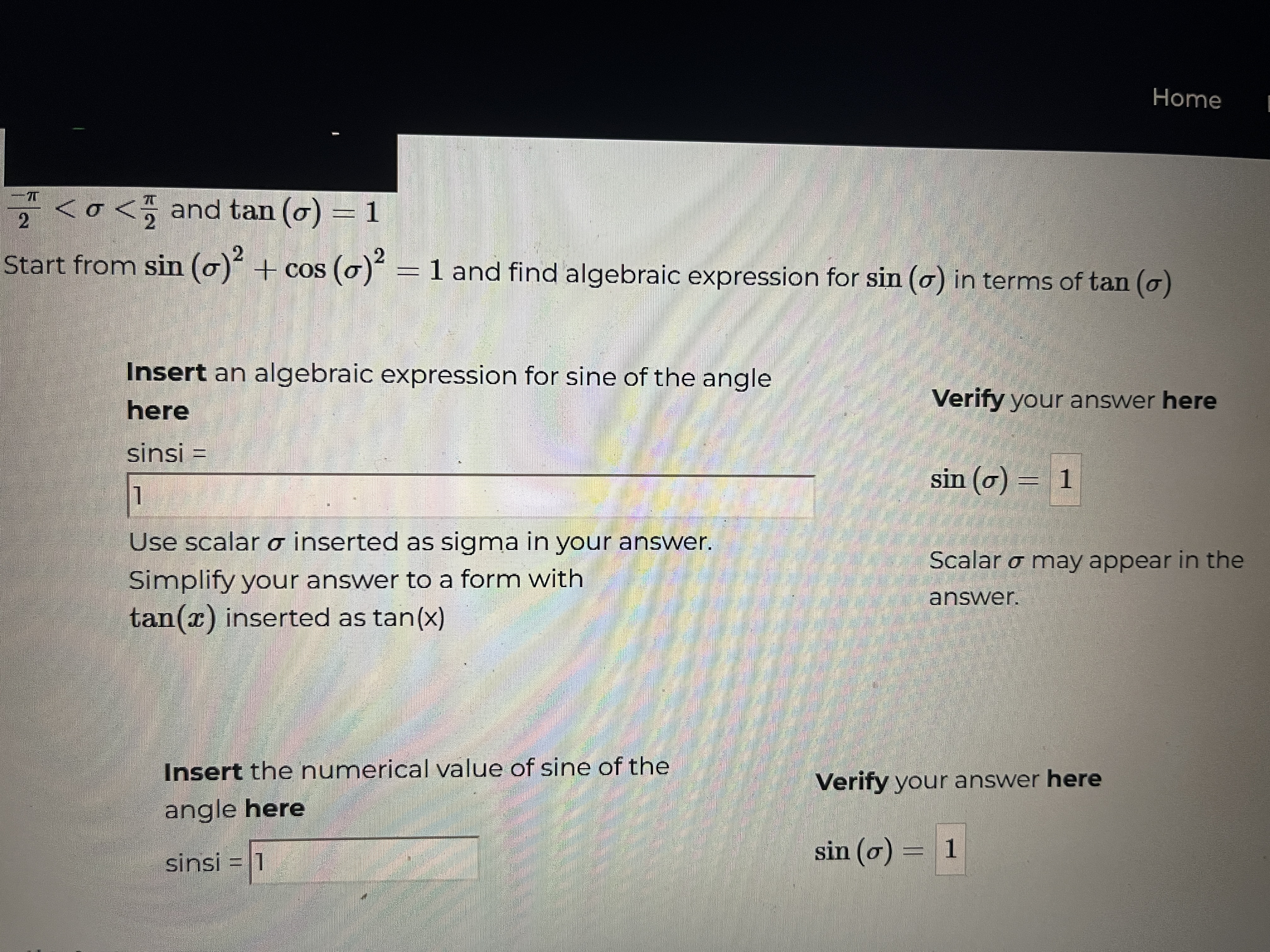
Insert an algebraic expression for sine of the angle here and insert the numerical value of sine of the angle here.

Steps to Solve

1. Start with the Pythagorean Identity

The fundamental identity is given by: $$ \sin^2(\sigma) + \cos^2(\sigma) = 1 $$

2. Express Cosine in terms of Tangent

We know that: $$ \tan(\sigma) = \frac{\sin(\sigma)}{\cos(\sigma)} $$ From this, we can express cosine: $$ \cos(\sigma) = \frac{\sin(\sigma)}{\tan(\sigma)} $$

3. Substitute Cosine into the Identity

Substituting $\cos(\sigma)$ in the Pythagorean identity gives: $$ \sin^2(\sigma) + \left(\frac{\sin(\sigma)}{\tan(\sigma)}\right)^2 = 1 $$

4. Simplify the Equation

This can be rewritten as: $$ \sin^2(\sigma) + \frac{\sin^2(\sigma)}{\tan^2(\sigma)} = 1 $$ Let $y = \sin^2(\sigma)$. We then have: $$ y + \frac{y}{\tan^2(\sigma)} = 1 $$

5. Combine and Solve for Sin^2(σ)

Multiplying through by $\tan^2(\sigma)$ gives: $$ y \tan^2(\sigma) + y = \tan^2(\sigma)$$ Factoring out $y$: $$ y(\tan^2(\sigma) + 1) = \tan^2(\sigma)$$

6. Use the Identity $1 + \tan^2(\sigma) = \sec^2(\sigma)$

This allows us to write: $$ y \sec^2(\sigma) = \tan^2(\sigma)$$ Thus, $$ y = \frac{\tan^2(\sigma)}{\sec^2(\sigma)}$$

7. Final Expression for Sin(σ)

Since $y = \sin^2(\sigma)$, we can take the square root: $$ \sin(\sigma) = \sqrt{\frac{\tan^2(\sigma)}{\sec^2(\sigma)}} $$ This simplifies to: $$ \sin(\sigma) = \frac{\tan(\sigma)}{\sec(\sigma)}$$ Using the identity $\sec(\sigma) = \frac{1}{\cos(\sigma)}$, we can write it as: $$ \sin(\sigma) = \tan(\sigma) \cos(\sigma) $$

8. Numerical Value of Sin(σ)

Given that $\tan(\sigma) = 1$, in the specified range, we find: $$ \sigma = \frac{\pi}{4} \implies \sin(\sigma) = 1 $$