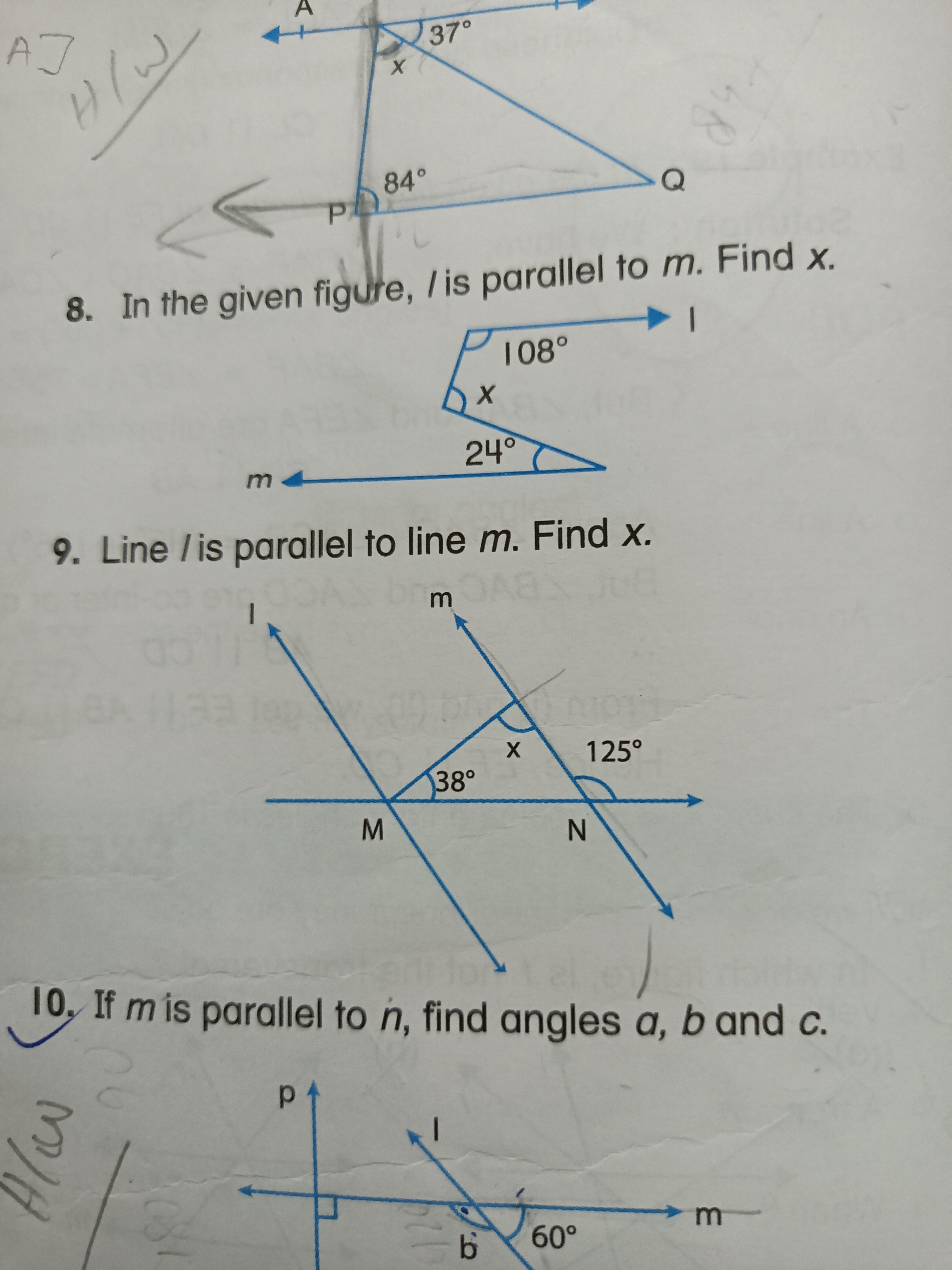
In the given figure, l is parallel to m. Find x. Line l is parallel to line m. Find x. If m is parallel to n, find angles a, b and c.
Understand the Problem
The question is asking to find the unknown angles (x) in parallel lines and transversals using properties of angles formed by parallel lines, along with identifying angles a, b, and c when two lines are parallel. The approach involves using angle relationships such as corresponding angles, alternate interior angles, and supplementary angles.
Answer
$x = 59^\circ, x = 55^\circ, a = 60^\circ, b = 60^\circ, c = 120^\circ$.
Answer for screen readers
- For Figure 8, $x = 59^\circ$.
- For Figure 9, $x = 55^\circ$.
- For Figure 10, $a = 60^\circ$, $b = 60^\circ$, $c = 120^\circ$.
Steps to Solve
-
Finding angle x in Figure 8
Using the fact that angles on a straight line add up to $180^\circ$, we can express the relationship as follows:
$$ 37^\circ + x + 84^\circ = 180^\circ $$
Thus, we can solve for $x$:
$$ x = 180^\circ - 37^\circ - 84^\circ $$ -
Calculate x for Figure 8
Perform the calculation:
$$ x = 180^\circ - 121^\circ = 59^\circ $$
So, $x = 59^\circ$. -
Finding angle x in Figure 9
Apply the same concept where the angles at point N form a linear pair:
$$ x + 125^\circ = 180^\circ $$
Thus, we have:
$$ x = 180^\circ - 125^\circ $$ -
Calculate x for Figure 9
Perform the calculation:
$$ x = 55^\circ $$
So, $x = 55^\circ$. -
Finding angles a, b, and c in Figure 10
Since lines m and n are parallel, use the property of corresponding angles:
$$ a = 60^\circ $$
Also, angle b is equal to angle at point P (alternate interior angles):
$$ b = 60^\circ $$
For angle c, we know it forms a linear pair with angle b:
$$ c + b = 180^\circ $$
Thus, we have:
$$ c = 180^\circ - 60^\circ $$ -
Calculate angle c
Perform the calculation:
$$ c = 120^\circ $$
- For Figure 8, $x = 59^\circ$.
- For Figure 9, $x = 55^\circ$.
- For Figure 10, $a = 60^\circ$, $b = 60^\circ$, $c = 120^\circ$.
More Information
The calculations involve the properties of angles formed by intersecting lines. The properties utilized include supplementary angles and alternate interior angles that arise when dealing with parallel lines and transversals.
Tips
- Misidentifying the relationships between the angles, such as corresponding vs. alternate angles.
- Forgetting that angles on a straight line sum to $180^\circ$.
AI-generated content may contain errors. Please verify critical information