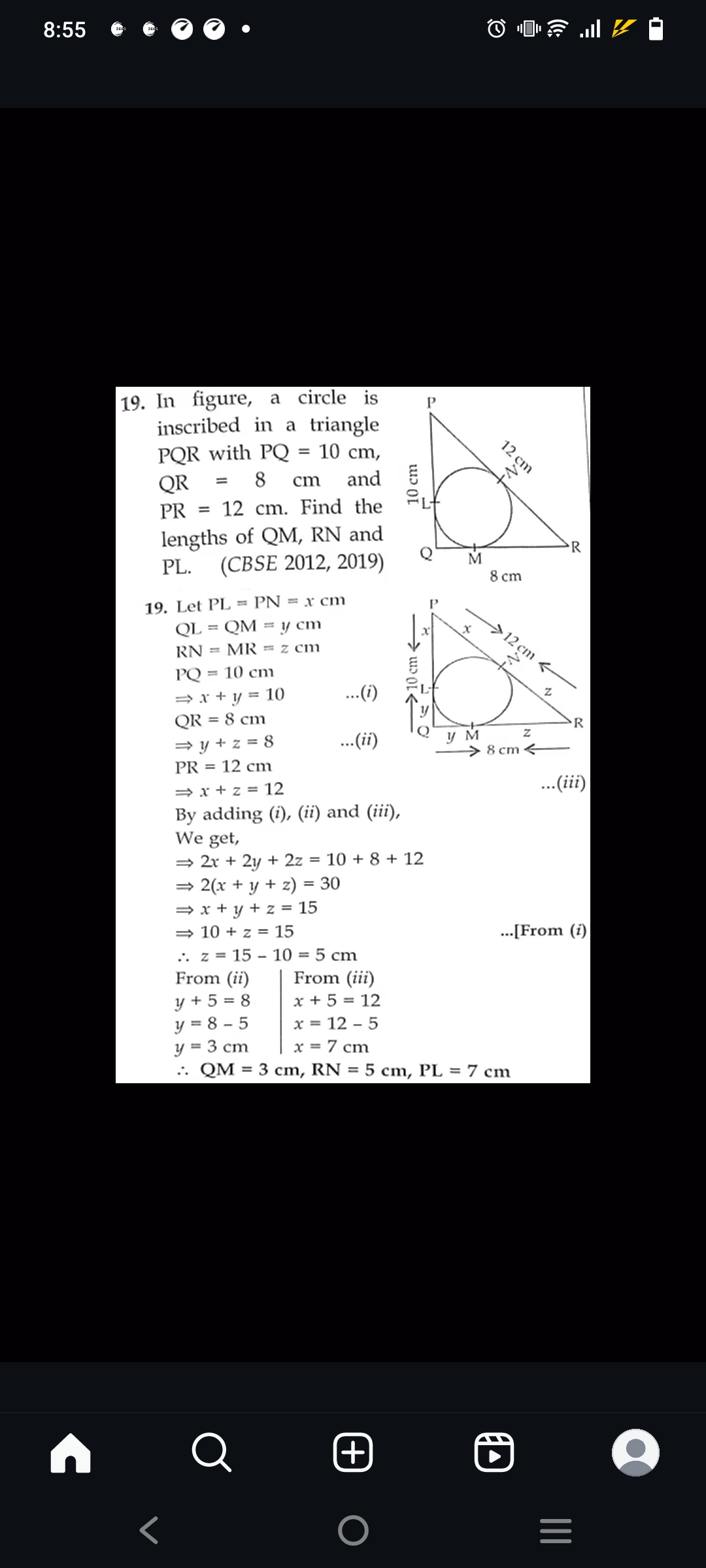
In figure, a circle is inscribed in a triangle PQR with PQ = 10 cm, QR = 8 cm, and PR = 12 cm. Find the lengths of QM, RN, and PL.

Steps to Solve

1. Assign Variables for Segment Lengths

Let the lengths of the segments be assigned as follows:

• ( PL = PN = x )
• ( QM = y )
• ( RN = MR = z )

2. Set Up Equations Based on Triangle Sides

From the triangle side lengths, we can create equations:

• For ( PQ = 10 ) cm: $$ x + y = 10 \quad \text{(i)} $$

• For ( QR = 8 ) cm: $$ y + z = 8 \quad \text{(ii)} $$

• For ( PR = 12 ) cm: $$ x + z = 12 \quad \text{(iii)} $$

3. Combine the Equations

Add equations (i), (ii), and (iii):

$$ 2x + 2y + 2z = 10 + 8 + 12 $$ $$ 2(x + y + z) = 30 $$ $$ x + y + z = 15 $$

4. Substitute to Find Individual Values

Next, we can use the equation ( x + y + z = 15 ) to find ( z ):

From (i): $$ y = 10 - x $$

Substitute ( y ) into (ii): $$ (10 - x) + z = 8 $$ $$ z = 8 - (10 - x) $$ $$ z = x - 2 $$

Now substitute ( z ) back into (iii): $$ x + (x - 2) = 12 $$ $$ 2x - 2 = 12 $$ $$ 2x = 14 $$ $$ x = 7 $$

5. Use the Value of ( x ) to Find ( y ) and ( z )

Now that we have ( x ):

• Substitute ( x ) back into (i) to find ( y ): $$ 7 + y = 10 $$ $$ y = 3 $$

• Substitute ( x ) into the equation for ( z ): $$ z = 7 - 2 = 5 $$

Thus, we find:

• ( QM = y = 3 ) cm
• ( RN = z = 5 ) cm
• ( PL = x = 7 ) cm