How do I categorize these equations based on their number of solutions?

Understand the Problem

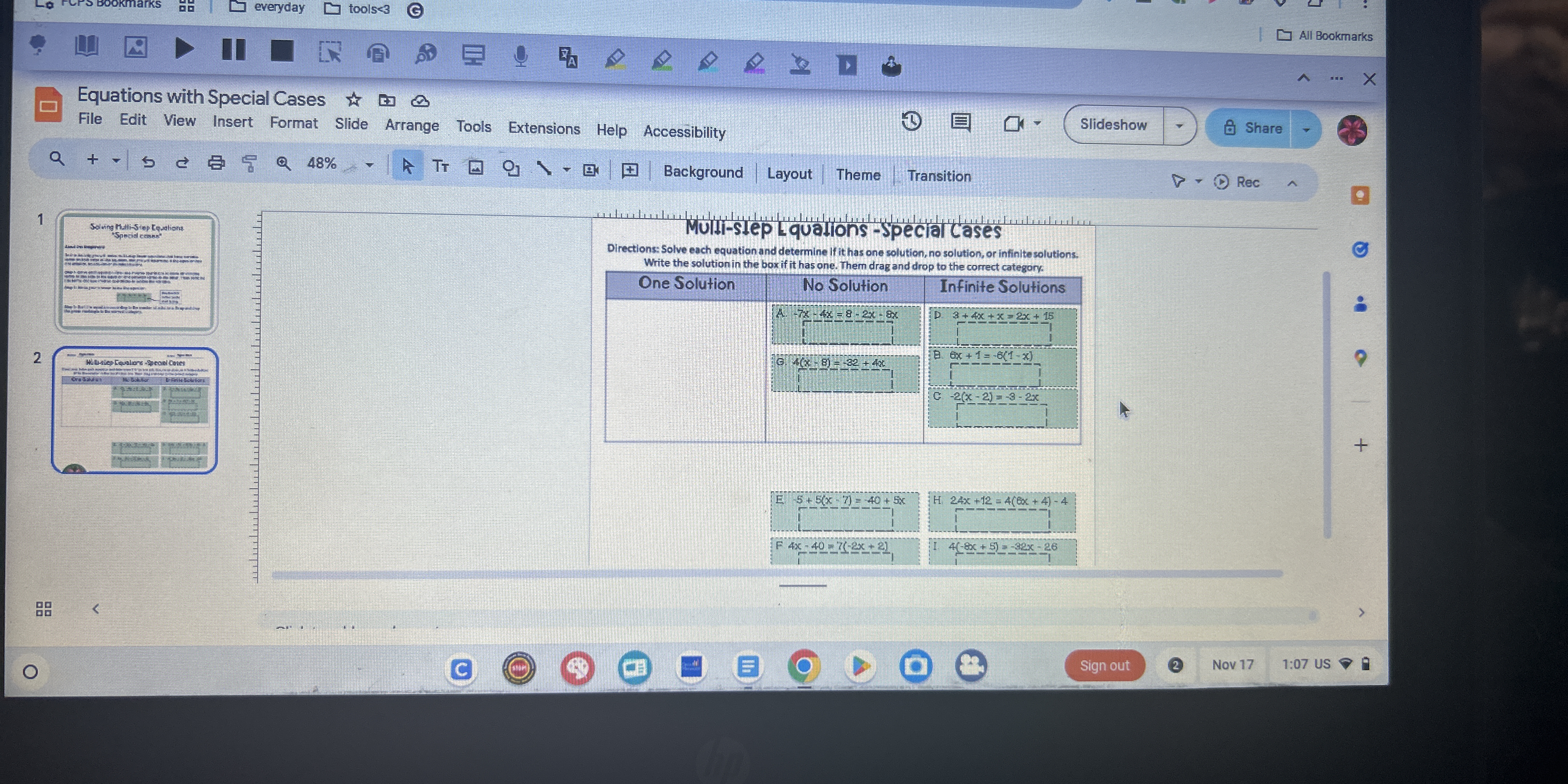
The image displays a worksheet focused on solving multi-step equations with special cases, asking to categorize each equation by the number of solutions it has (one solution, no solution, or infinite solutions).

Answer

Equations categorized as follows: - One Solution: A, C, D, G - No Solution: B, E, F - Infinite Solutions: H

Steps to Solve

Identify the equations Look at each equation in the boxes provided (A to H) and write them down.
Simplify each equation For every equation, combine like terms and simplify both sides as much as possible to see if they can be categorized based on their solutions.
Determine the number of solutions Evaluate each simplified equation:
- One Solution: If the variables can be isolated to give a specific number (e.g., $x = 2$).
- No Solution: If the equation simplifies to a false statement (e.g., $0 = 5$).
- Infinite Solutions: If the equation simplifies to a true statement for all values (e.g., $0 = 0$).
Categorize the equations Place each equation into one of the three categories (One Solution, No Solution, Infinite Solutions) based on the results from the previous step.
Double-check and validate Review each categorized equation to ensure they meet the criteria for the respective categories.

Equations with One Solution: A, C, D, G
Equations with No Solution: B, E, F
Equations with Infinite Solutions: H

More Information

In multi-step equations, identifying the nature of the solutions is crucial. One solution is a single value, no solution indicates a contradiction (like $0 = 5$), while infinite solutions imply the variables cancel out entirely to give a true statement.

Tips

Not simplifying the equation fully before categorizing.
Confusing "no solution" with "infinite solutions".
Misidentifying where like terms can be combined or where terms can be canceled.

AI-generated content may contain errors. Please verify critical information

Thank you for voting!