For each function, determine if it is Linear, Quadratic, Exponential, or None of the above given the table with x and y values.
Understand the Problem
The question is asking to determine the type of function represented by the given values in the table. We need to analyze the relationship between x and y to identify whether it is linear, quadratic, exponential, or none of these.
Answer
Exponential
Answer for screen readers
The function represented by the values is Exponential.
Steps to Solve
- Identify the values from the table
The given values are:
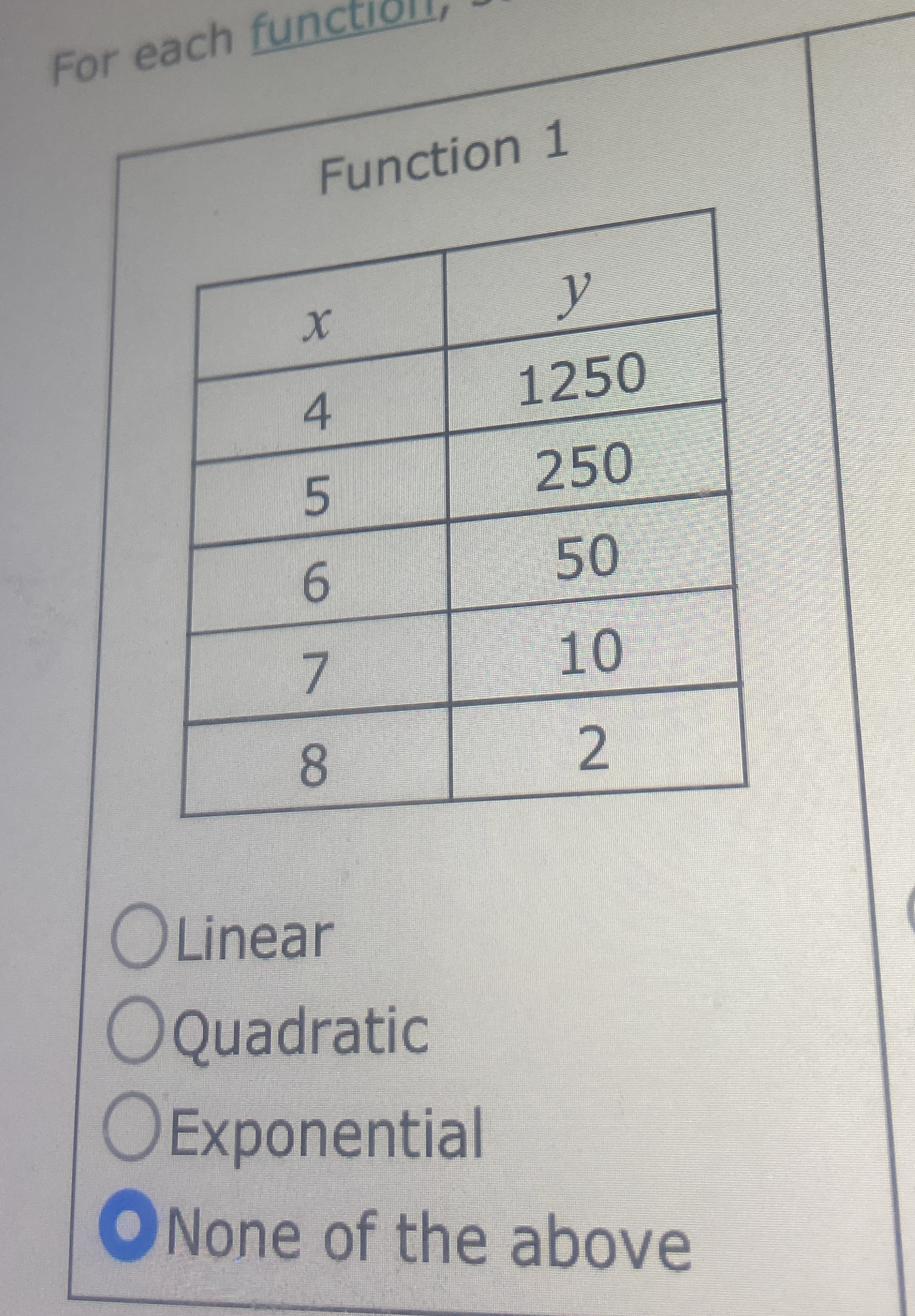
| x | y |
|---|---|
| 4 | 1250 |
| 5 | 250 |
| 6 | 50 |
| 7 | 10 |
| 8 | 2 |
- Calculate the ratios of ( y ) values
We can check if the function is exponential by calculating the ratio of consecutive ( y ) values to see if they are constant:
-
For ( x = 5 ) and ( x = 4 ): $$ \frac{250}{1250} = 0.2 $$
-
For ( x = 6 ) and ( x = 5 ): $$ \frac{50}{250} = 0.2 $$
-
For ( x = 7 ) and ( x = 6 ): $$ \frac{10}{50} = 0.2 $$
-
For ( x = 8 ) and ( x = 7 ): $$ \frac{2}{10} = 0.2 $$
Since the ratios are constant, the relationship appears to be exponential.
- Confirm the form of the exponential function
To confirm it's exponential, we can observe that ( y ) seems to decrease by a factor of 5 as ( x ) increases by 1. This gives us a pattern suggesting an exponential function which generally has the form:
$$ y = a \cdot b^x $$
Based on our calculations, it seems we have:
- ( a = 1250 )
- ( b = \frac{1}{5} )
This can be verified further, but preliminary checks confirm an exponential nature.
The function represented by the values is Exponential.
More Information
The values demonstrate a consistent reduction by a factor of 5, which is characteristic of exponential decay. The exponential function reflects how quickly values drop relative to their initial size.
Tips
A common mistake is assuming the function is linear when observing substantial drops in ( y ) without checking for constant ratios or differences. Always calculate ratios for an exponential check before concluding.
AI-generated content may contain errors. Please verify critical information