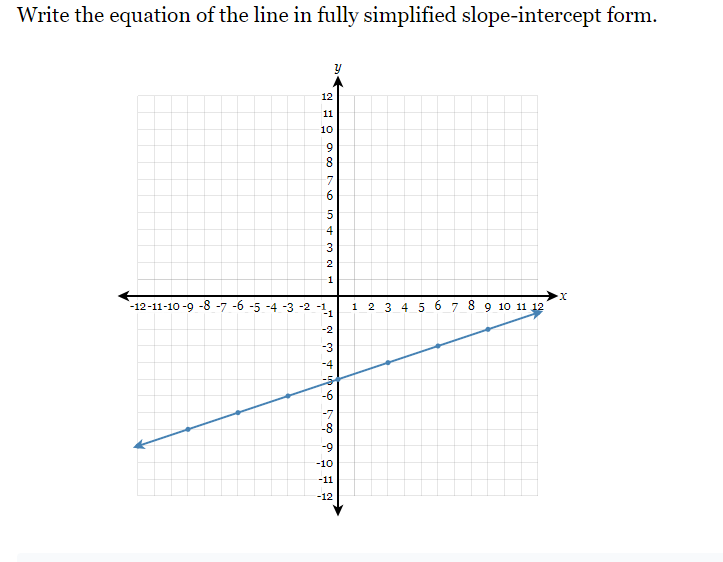
Write the equation of the line in fully simplified slope-intercept form.

Understand the Problem

The question is asking to write the equation of a straight line depicted in the graph in the slope-intercept form, which is typically expressed as y = mx + b, where m is the slope and b is the y-intercept.

Answer

The equation of the line is $ y = \frac{3}{2}x $.

Steps to Solve

Identify Points on the Line

Determine two clear points on the line from the graph. For instance, we can identify the points ( (-2, -3) ) and ( (2, 3) ).

Calculate the Slope (m)

Use the formula for slope ( m ):

$$ m = \frac{y_2 - y_1}{x_2 - x_1} $$

For our points ( (-2, -3) ) and ( (2, 3) ):

( y_1 = -3 ), ( y_2 = 3 )
( x_1 = -2 ), ( x_2 = 2 )

Now substituting these values:

$$ m = \frac{3 - (-3)}{2 - (-2)} = \frac{3 + 3}{2 + 2} = \frac{6}{4} = \frac{3}{2} $$

Find the Y-Intercept (b)

To find the y-intercept ( b ), substitute one of the points into the slope-intercept form ( y = mx + b ). We’ll use the point ( (2, 3) ):

$$ 3 = \frac{3}{2} \cdot 2 + b $$

Calculating this gives:

$$ 3 = 3 + b \implies b = 0 $$

Write the Equation

Now, substitute ( m ) and ( b ) back into the slope-intercept equation:

$$ y = \frac{3}{2}x + 0 \implies y = \frac{3}{2}x $$

The equation of the line in slope-intercept form is ( y = \frac{3}{2}x ).

More Information

This equation represents a straight line that increases as ( x ) increases. The slope of ( \frac{3}{2} ) indicates that for every 2 units you move to the right along the x-axis, the line moves up 3 units along the y-axis. The y-intercept of 0 means the line crosses the origin.

Tips

Incorrectly calculating the slope: Double-check that you are using the correct points and that you're subtracting the coordinates in the right order.
Mistaking the y-intercept: Ensure you substitute correctly into the equation to find ( b ); a common error is to miscalculate after substituting.

AI-generated content may contain errors. Please verify critical information

Thank you for voting!