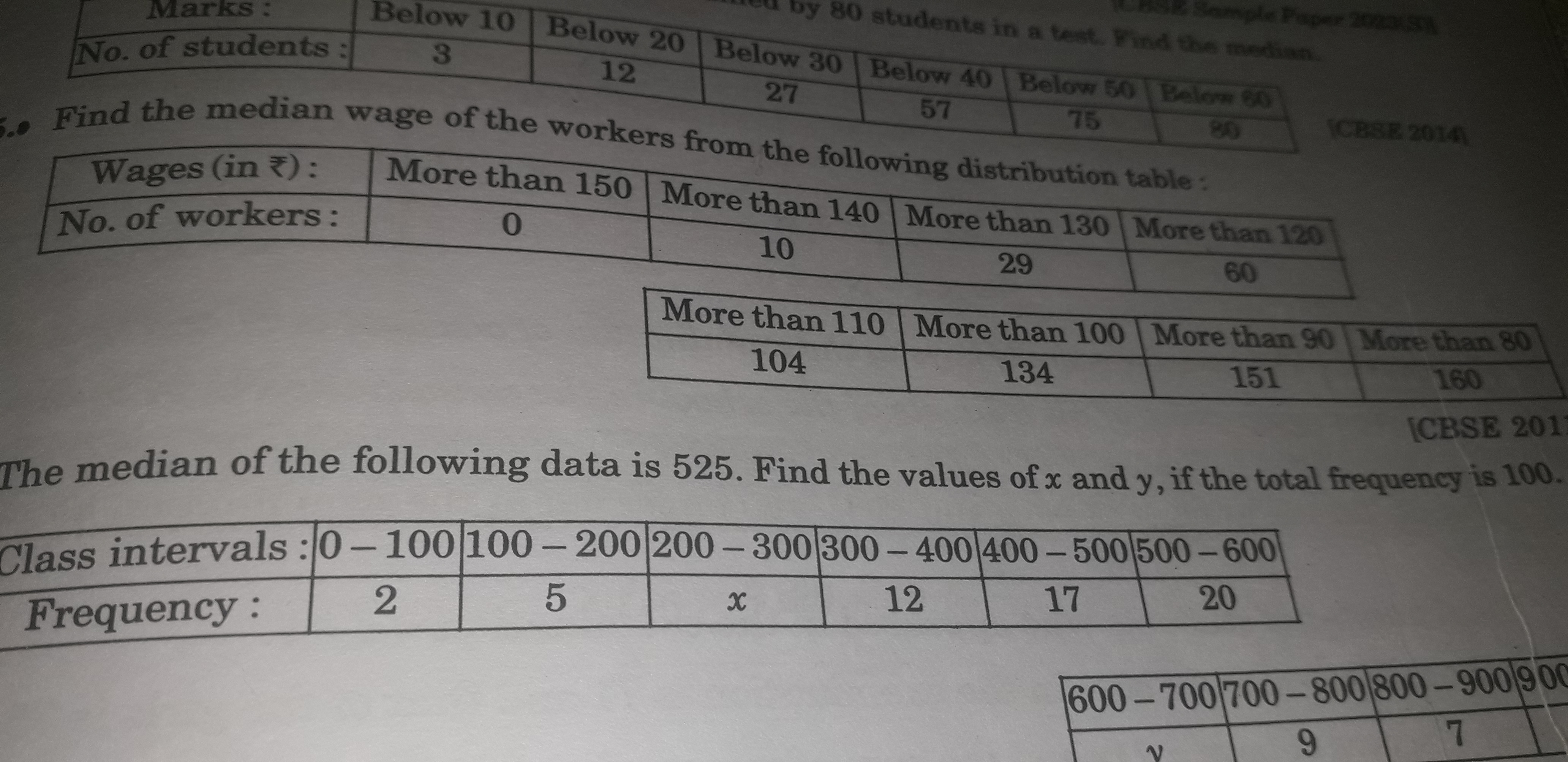
Find the median wage of the workers from the following distribution table: Wages (in ₹): More than 150, More than 140, More than 130, More than 120, More than 110, More than 100, M... Find the median wage of the workers from the following distribution table: Wages (in ₹): More than 150, More than 140, More than 130, More than 120, More than 110, More than 100, More than 90, More than 80. The median of the following data is 525. Find the values of x and y, if the total frequency is 100. Read more

Steps to Solve

1. Understanding Class Intervals and Frequencies

   The table provides class intervals for wages and respective frequencies for the number of workers. The goal is to find the values of $x$ and $y$, given that the total frequency equals 100.

2. Calculating the Total Frequency

   We first sum the known frequencies in the table. The frequencies are:

   • 2 (for 0-100)
   • 5 (for 100-200)
   • $x$ (for 200-300)
   • 12 (for 300-400)
   • 17 (for 400-500)
   • 20 (for 500-600)

   The total frequency equation can be written as: $$ 2 + 5 + x + 12 + 17 + 20 + y = 100 $$

3. Simplifying the Equation

   Now sum the constant terms: $$ 2 + 5 + 12 + 17 + 20 = 56 $$ This gives us the equation: $$ 56 + x + y = 100 $$

4. Finding x and y

   From the simplified equation: $$ x + y = 100 - 56 $$ $$ x + y = 44 $$

5. Using the Median Information

   Given the median is 525, we first determine in which class interval the median falls. With total frequency 100, the median position is: $$ \frac{100 + 1}{2} = 50 $$

   Now we find the cumulative frequencies to identify the median class.

6. Cumulative Frequencies

   • Cumulative frequency for 0-100: 2
   • Cumulative frequency for 100-200: 2 + 5 = 7
   • Cumulative frequency for 200-300: $7 + x$
   • Cumulative frequency for 300-400: $7 + x + 12 = 19 + x$
   • Cumulative frequency for 400-500: $19 + x + 17 = 36 + x$
   • Cumulative frequency for 500-600: $36 + x + 20 = 56 + x$

   For the cumulative frequency to reach 50: $$ 56 + x \geq 50 $$ This means $x$ should be adjusted.

7. Solving for x

   Assuming $x = 44 - y$, to keep the cumulative frequency logic, replace $x$ in the scenarios to yield reasonable values for $y$.

8. Finding Values of x and y

   Start by testing values that satisfy ( x + y = 44 ) and check for median validity until two feasible integers are determined.