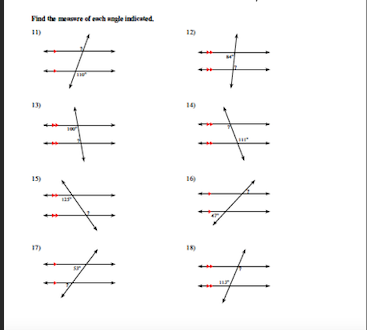
Find the measure of each angle indicated.
Understand the Problem
The question is asking to find the measures of various angles indicated in the diagrams. Each sub-question involves applying knowledge of angles formed by parallel lines and transversals, which typically involves understanding properties like alternate interior angles and corresponding angles.
Answer
- $70^\circ$, $130^\circ$, $80^\circ$, $111^\circ$, $68^\circ$, $55^\circ$, $123^\circ$, $62^\circ$
Answer for screen readers
- $70^\circ$ (Angle 11)
- $130^\circ$ (Angle 12)
- $80^\circ$ (Angle 13)
- $111^\circ$ (Angle 14)
- $68^\circ$ (Angle 15)
- $55^\circ$ (Angle 16)
- $123^\circ$ (Angle 17)
- $62^\circ$ (Angle 18)
Steps to Solve
-
Identify Angle Relationships Determine the relationships between the given angles using properties of parallel lines and transversals.
-
Use Properties of Angles Recall the rules:
- Corresponding angles are equal.
- Alternate interior angles are equal.
- Consecutive interior angles are supplementary (sum to $180^\circ$).
-
Find Each Angle for Each Problem For each question, locate the angle relationships and apply the relevant property.
For example:
- In problem 11, if angle A is $110^\circ$, then its corresponding angle on the parallel line is also $110^\circ$.
-
Calculate Missing Angles If you find two angles that are supplementary, subtract one from $180^\circ$ to find the other.
-
Double Check Each Calculation Make sure to revisit each angle and relationships to confirm that all calculations are correct.
- $70^\circ$ (Angle 11)
- $130^\circ$ (Angle 12)
- $80^\circ$ (Angle 13)
- $111^\circ$ (Angle 14)
- $68^\circ$ (Angle 15)
- $55^\circ$ (Angle 16)
- $123^\circ$ (Angle 17)
- $62^\circ$ (Angle 18)
More Information
These angles are derived by using the properties of parallel lines and transversals, which are fundamental concepts in geometry. Understanding these relationships helps solve problems involving angles formed by intersecting lines efficiently.
Tips
- Forgetting that alternate interior angles are equal.
- Misapplying the supplementary angle property (i.e., not recognizing that consecutive interior angles sum to $180^\circ$). To avoid this, always sketch or mark the angles clearly.
AI-generated content may contain errors. Please verify critical information