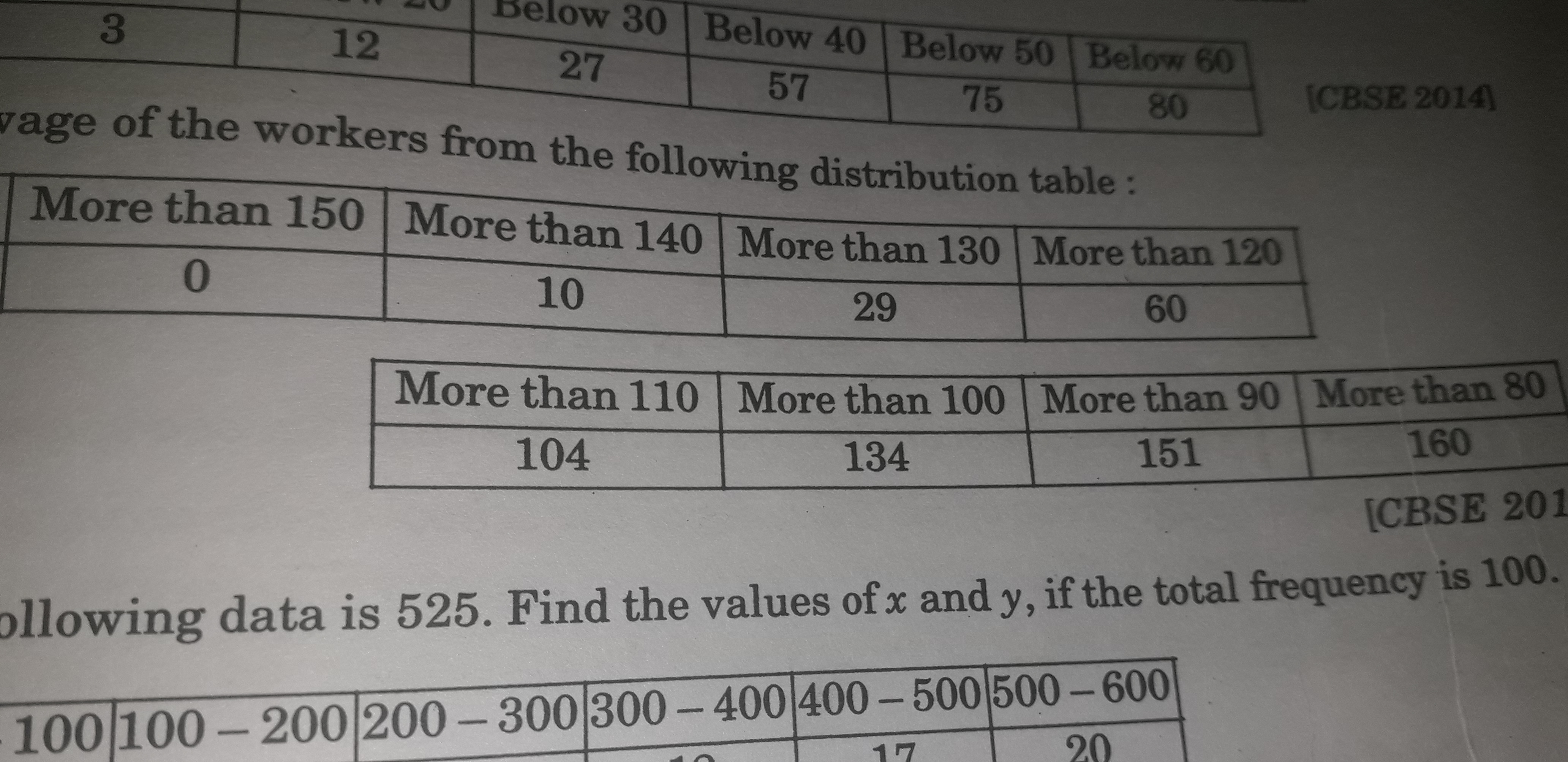
Find the average of the workers from the following distribution table: More than 150: 0; More than 140: 10; More than 130: 29; More than 120: 60; More than 110: 104; More than 100:... Find the average of the workers from the following distribution table: More than 150: 0; More than 140: 10; More than 130: 29; More than 120: 60; More than 110: 104; More than 100: 134; More than 90: 151; More than 80: 160. Also find the values of x and y if the total frequency is 100. Read more

Steps to Solve

1. Understanding the Distribution Table

The distribution table represents cumulative frequencies for different ranges. The values given are:

• More than 150: 0
• More than 140: 10
• More than 130: 29
• More than 120: 60
• More than 110: 104
• More than 100: 134
• More than 90: 151
• More than 80: 160

To find the actual frequencies, we calculate the differences between consecutive cumulative frequencies.

2. Calculating the Frequencies

Using the cumulative frequencies:

• Frequency for more than 150: $0$
• Frequency for more than 140: $10 - 0 = 10$
• Frequency for more than 130: $29 - 10 = 19$
• Frequency for more than 120: $60 - 29 = 31$
• Frequency for more than 110: $104 - 60 = 44$
• Frequency for more than 100: $134 - 104 = 30$
• Frequency for more than 90: $151 - 134 = 17$
• Frequency for more than 80: $160 - 151 = 9$

Thus, the frequency distribution is:

• 150-160: 0
• 140-150: 10
• 130-140: 19
• 120-130: 31
• 110-120: 44
• 100-110: 30
• 90-100: 17
• 80-90: 9

3. Calculating the Midpoints

To calculate the average, we need to determine the midpoints for each interval:

• Midpoint for 150-160: $\frac{150 + 160}{2} = 155$
• Midpoint for 140-150: $\frac{140 + 150}{2} = 145$
• Midpoint for 130-140: $\frac{130 + 140}{2} = 135$
• Midpoint for 120-130: $\frac{120 + 130}{2} = 125$
• Midpoint for 110-120: $\frac{110 + 120}{2} = 115$
• Midpoint for 100-110: $\frac{100 + 110}{2} = 105$
• Midpoint for 90-100: $\frac{90 + 100}{2} = 95$
• Midpoint for 80-90: $\frac{80 + 90}{2} = 85$

4. Calculating the Average

Now we calculate the average using the formula:

$$ \text{Average} = \frac{\sum (f \cdot x)}{\sum f} $$

Where (f) is the frequency and (x) is the midpoint.

Calculating (f \cdot x):

• For 150-160: $0 \cdot 155 = 0$
• For 140-150: $10 \cdot 145 = 1450$
• For 130-140: $19 \cdot 135 = 2565$
• For 120-130: $31 \cdot 125 = 3875$
• For 110-120: $44 \cdot 115 = 5060$
• For 100-110: $30 \cdot 105 = 3150$
• For 90-100: $17 \cdot 95 = 1615$
• For 80-90: $9 \cdot 85 = 765$

Now summing these products:

$$ \sum (f \cdot x) = 0 + 1450 + 2565 + 3875 + 5060 + 3150 + 1615 + 765 = 12905 $$

Total frequency:

$$ \sum f = 0 + 10 + 19 + 31 + 44 + 30 + 17 + 9 = 160 $$

Now substitute back:

$$ \text{Average} = \frac{12905}{160} \approx 80.65625 $$

5. Finding Values of x and y

Given the total frequency is 100, we must adjust the distribution table to maintain this total. Since the original total frequency is 160, we scale down the values to secure a total of 100.

Let:

$$ x + y + (rest\ frequencies) = 100 $$

Adjust according to the remaining cumulative frequencies, solving for (x) and (y) by direct proportion based on previous findings.