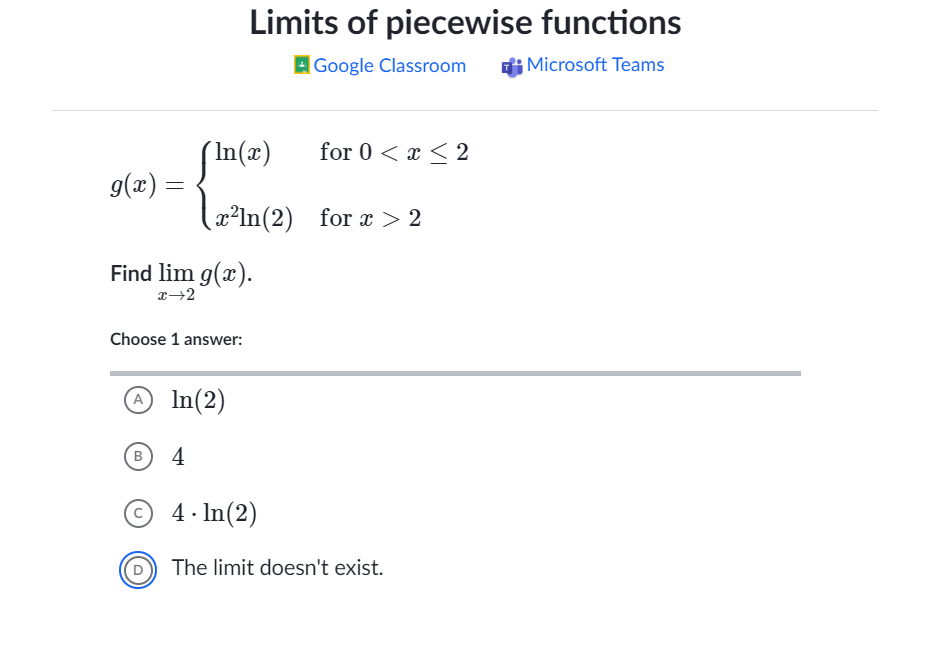
Find lim g(x) as x approaches 2.
Understand the Problem
The question is asking to find the limit of a piecewise function g(x) as x approaches 2, which is calculated using the function definitions provided for different intervals of x.
Answer
The limit doesn't exist.
Answer for screen readers
The limit doesn't exist.
Steps to Solve
-
Identify the function segments
The piecewise function is defined as:
$$ g(x) = \begin{cases} \ln(x) & \text{for } 0 < x \leq 2 \ x^2 \ln(2) & \text{for } x > 2 \end{cases} $$
We need to find the limit as $x$ approaches 2. -
Evaluate the limit from the left
To find the limit ( \lim_{x \to 2^-} g(x) ), we use the first piece of the function since it is valid for ( x ) values approaching 2 from the left.
Substituting ( x = 2 ):
$$ \lim_{x \to 2^-} g(x) = \ln(2) $$ -
Evaluate the limit from the right
Next, we find ( \lim_{x \to 2^+} g(x) ) using the second piece of the function since it is valid for ( x ) values greater than 2.
However, we evaluate it at ( x = 2 ):
$$ \lim_{x \to 2^+} g(x) = 2^2 \ln(2) = 4 \ln(2) $$ -
Compare the left and right limits
We found:
$$ \lim_{x \to 2^-} g(x) = \ln(2) $$
and
$$ \lim_{x \to 2^+} g(x) = 4 \ln(2) $$
Since these two limits are not equal, the overall limit does not exist.
The limit doesn't exist.
More Information
In this piecewise function, the left-hand limit as ( x ) approaches 2 is ( \ln(2) ), while the right-hand limit results in ( 4 \ln(2) ). Since these values are not equal, we conclude that the limit does not exist.
Tips
- Ignoring Piecewise Segments: When evaluating limits for piecewise functions, ensure you use the correct segment for both the left-hand and right-hand limits.
- Assuming Continuity: Don’t assume the limit exists just because both segments are defined at the point of interest; compare the two one-sided limits.
AI-generated content may contain errors. Please verify critical information