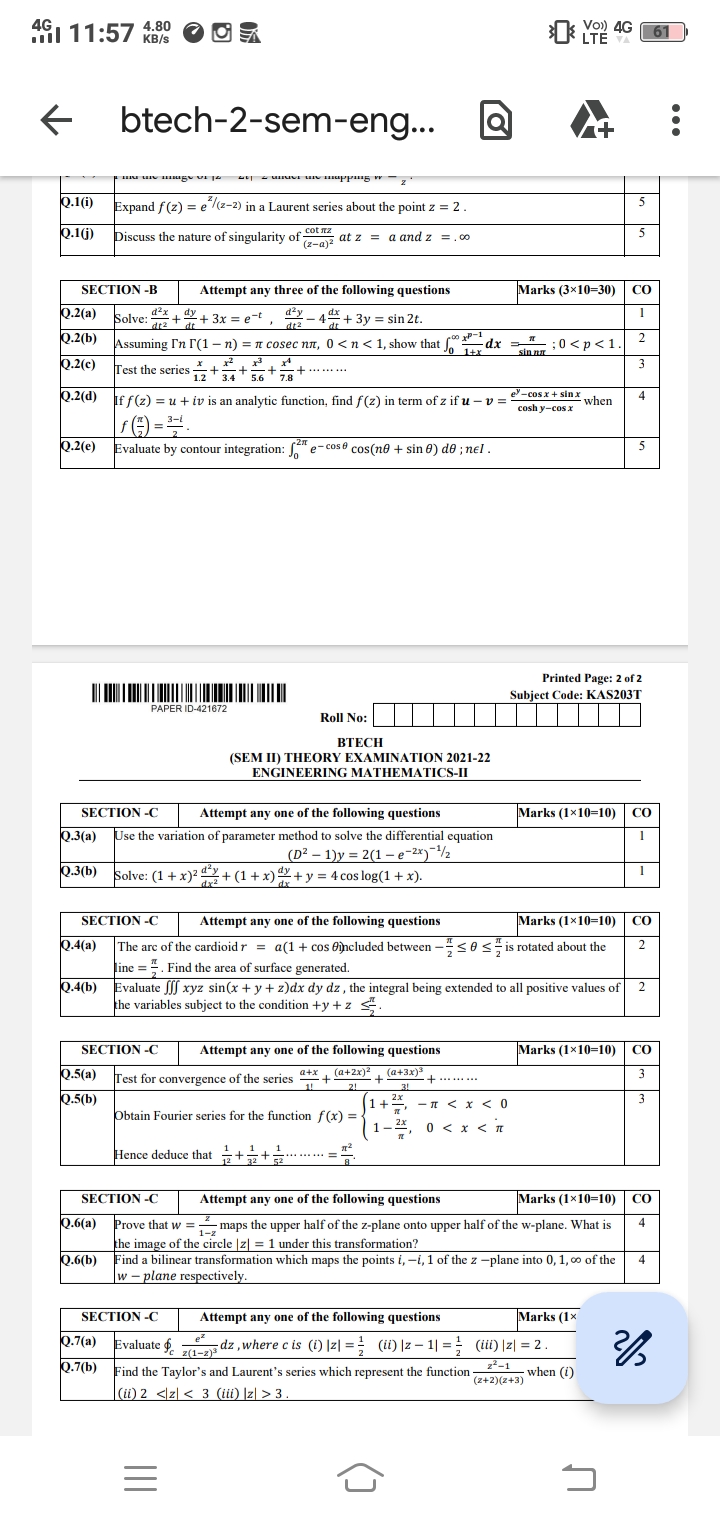
Attached image contains multiple mathematics questions including solving differential equations, finding Laurent series, testing convergence of series, and working with Fourier ser... Attached image contains multiple mathematics questions including solving differential equations, finding Laurent series, testing convergence of series, and working with Fourier series. Read more

Steps to Solve

1. Identify the problem to solve Select one of the questions to solve. For demonstration, let's use Q2(a): Solve the differential equation ( \frac{d^2x}{dt^2} + 3x = e^{-t} ).

2. Write the characteristic equation The first step for solving a second-order differential equation with constant coefficients is to find the characteristic equation. For the given ( \frac{d^2x}{dt^2} + 3x = 0 ), we set up: $$ r^2 + 3 = 0 $$ This gives: $$ r = \pm i\sqrt{3} $$

3. Formulate the general solution of the homogeneous equation The general solution for the homogeneous equation can be expressed as: $$ x_h(t) = C_1 \cos(\sqrt{3}t) + C_2 \sin(\sqrt{3}t) $$

4. Find a particular solution To find the particular solution ( x_p(t) ) for the non-homogeneous part ( e^{-t} ), we use the method of undetermined coefficients. Assume: $$ x_p(t) = A e^{-t} $$ Take the first and second derivatives: $$ x_p'(t) = -A e^{-t} $$ $$ x_p''(t) = A e^{-t} $$

5. Substitute back into the equation Plug the particular solution into the original equation: $$ A e^{-t} + 3A e^{-t} = e^{-t} $$ Thus: $$ 4A e^{-t} = e^{-t} $$ This implies: $$ 4A = 1 \Rightarrow A = \frac{1}{4} $$

6. Combine general and particular solutions The complete solution is given by: $$ x(t) = x_h(t) + x_p(t) $$ Thus: $$ x(t) = C_1 \cos(\sqrt{3}t) + C_2 \sin(\sqrt{3}t) + \frac{1}{4} e^{-t} $$