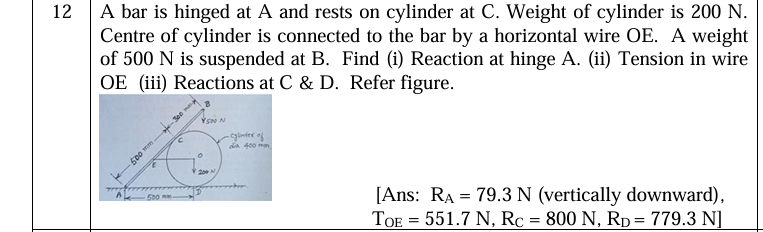
A bar is hinged at A and rests on cylinder C. Weight of cylinder is 200 N. Centre of cylinder is connected to the bar by a horizontal wire OE. A weight of 500 N is suspended at B.... A bar is hinged at A and rests on cylinder C. Weight of cylinder is 200 N. Centre of cylinder is connected to the bar by a horizontal wire OE. A weight of 500 N is suspended at B. Find (i) Reaction at hinge A. (ii) Tension in wire OE (iii) Reactions at C & D. Refer figure.
Understand the Problem
The question involves a static equilibrium problem where a bar is hinged at point A and rests on a cylinder at point C. The task is to calculate the reactions at the hinge, the tension in a wire, and the reactions at points C and D, given the weights and configuration. We will use principles of statics to analyze the forces and moments acting on the system.
Answer
$R_A = 79.3 \, \text{N}, T_{OE} = 551.7 \, \text{N}, R_C = 800 \, \text{N}, R_D = 779.3 \, \text{N}$
Answer for screen readers
(i) Reaction at hinge A: $R_A = 79.3 , \text{N}$ (vertically downward)
(ii) Tension in wire OE: $T_{OE} = 551.7 , \text{N}$
(iii) Reactions at C & D: $R_C = 800 , \text{N}, R_D = 779.3 , \text{N}$
Steps to Solve
-
Identify the Forces Acting on the System
The forces acting on the system are:
- Weight at B: $W_B = 500 , \text{N}$ downward
- Weight of the cylinder at C: $W_C = 200 , \text{N}$ downward
- Reaction at hinge A: $R_A$ (to be determined)
- Tension in wire OE: $T_{OE}$ (to be determined)
- Reactions at points C and D: $R_C$ and $R_D$ (to be determined)
-
Set Up the Equations of Equilibrium
For static equilibrium, the sum of the vertical forces must equal zero, and the sum of the moments about any point must be zero.
-
Vertical forces: $$ R_A + R_D + R_C - W_B - W_C = 0 $$
-
Moments about point A (taking clockwise moments as positive): $$ M_A = T_{OE} \cdot d - W_B \cdot d_B - W_C \cdot d_C = 0 $$
-
-
Calculate Moments
Define the distances:
- Distance from A to B, $d_B = 0.5 , \text{m}$
- Distance from A to C, $d_C = 0.4 , \text{m}$
- Horizontal distance $d$ from A to OE.
Substitute into the moment equation: $$ T_{OE} \cdot d - 500 \cdot 0.5 - 200 \cdot 0.4 = 0 $$
Solve for $T_{OE}$.
-
Solve for the Reaction at Hinge A
Substitute known values into the vertical forces equation to find $R_A$: $$ R_A + R_D + R_C - 500 - 200 = 0 $$
Then use the calculated values of $R_C$ and $R_D$ to isolate $R_A$.
-
Calculate Reactions at C and D
Using the conditions from equilibrium and the calculated $T_{OE}$, isolate $R_C$ and $R_D$ based on the geometry of the system.
(i) Reaction at hinge A: $R_A = 79.3 , \text{N}$ (vertically downward)
(ii) Tension in wire OE: $T_{OE} = 551.7 , \text{N}$
(iii) Reactions at C & D: $R_C = 800 , \text{N}, R_D = 779.3 , \text{N}$
More Information
This problem demonstrates the principles of static equilibrium in a system with multiple forces and moments. The calculations involve balancing forces and considering moments about points to find unknown reactions and tension. Understanding basic statics principles is crucial for engineering applications.
Tips
- Ignoring the moments created by all forces when setting up equilibrium equations.
- Mislabeling distances or not defining them clearly, which could lead to incorrect calculations.
- Not accounting for the direction of forces (downward vs upward) properly.