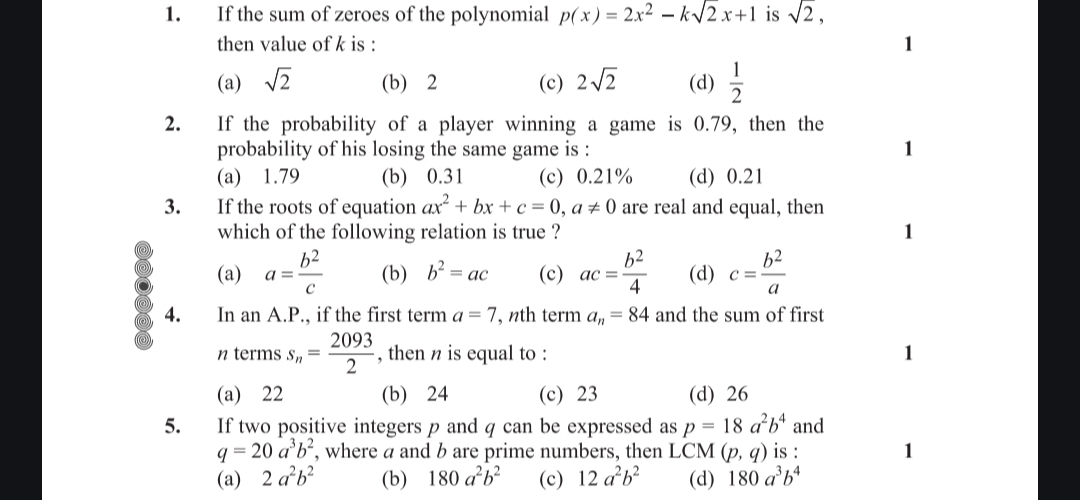
1. If the sum of zeroes of the polynomial p(x) = 2x² - k√2x + 1 is √2, then value of k is: (a) √2 (b) 2 (c) 2√2 (d) 1/2 2. If the probability of a player winning a game is 0.7... 1. If the sum of zeroes of the polynomial p(x) = 2x² - k√2x + 1 is √2, then value of k is: (a) √2 (b) 2 (c) 2√2 (d) 1/2 2. If the probability of a player winning a game is 0.79, then the probability of his losing the same game is: (a) 1.79 (b) 0.31 (c) 0.21% (d) 0.21 3. If the roots of equation ax² + bx + c = 0, a≠0 are real and equal, then which of the following relation is true? (a) a = b²/c (b) b² = ac (c) ac = b²/4 (d) c = b²/a 4. In an A.P., if the first term a = 7, nth term a_n = 84 and the sum of first n terms S_n = 2093/2, then n is equal to: (a) 22 (b) 24 (c) 23 (d) 26 5. If two positive integers p and q can be expressed as p = 18a²b⁴ and q = 20a³b², where a and b are prime numbers, then LCM(p, q) is: (a) 20a³b² (b) 180a²b² (c) 12a²b² (d) 180a³b⁴ Read more

Steps to Solve

1. Sum of Zeroes of the Polynomial

The sum of the zeroes of the polynomial ( p(x) = 2x^2 - k\sqrt{2}x + 1 ) can be found using Vieta's formulas, which states that for a polynomial of the form ( ax^2 + bx + c ), the sum of the roots is given by ( -\frac{b}{a} ). Here, ( a = 2 ), ( b = -k\sqrt{2} ). Thus, the sum of the zeroes is: $$ -\frac{-k\sqrt{2}}{2} = \frac{k\sqrt{2}}{2} $$

Setting this equal to ( \sqrt{2} ): $$ \frac{k\sqrt{2}}{2} = \sqrt{2} $$ Multiplying both sides by 2: $$ k\sqrt{2} = 2\sqrt{2} $$ Dividing both sides by ( \sqrt{2} ): $$ k = 2 $$

2. Probability of Losing

If the probability of winning is 0.79, then the probability of losing can be calculated as: $$ \text{Probability of losing} = 1 - \text{Probability of winning} $$ Substituting in the value: $$ \text{Probability of losing} = 1 - 0.79 = 0.21 $$

3. Roots of the Quadratic Equation

For the quadratic equation ( ax^2 + bx + c = 0 ) with equal real roots, the discriminant ( b^2 - 4ac = 0 ). We can use this to find the required relationship: Thus, using this relation: $$ b^2 = 4ac $$ This corresponds to option (c).

4. Finding ( n ) in A.P.

Given the first term ( a = 7 ), the nth term ( a_n = 84 ), and the sum of the first ( n ) terms: Using the formula for the nth term of an A.P., where ( a_n = a + (n - 1)d ), we can deduce: $$ 84 = 7 + (n - 1)d $$ Thus: $$ (n - 1)d = 84 - 7 = 77 $$

The formula for the sum of the first ( n ) terms is: $$ S_n = \frac{n}{2}(2a + (n - 1)d) $$ Setting ( S_n = \frac{2093}{2} ): $$ \frac{n}{2}(14 + (n-1)d) = \frac{2093}{2} $$ Multiplying both sides by 2: $$ n(14 + (n - 1)d) = 2093 $$ This can be simplified and solved for ( n ) upon substitution of ( d ).

5. Finding LCM of ( p ) and ( q )

Given ( p = 18a^2b^4 ) and ( q = 20a^3b^2 ), we find the LCM using each prime factor's highest power: The prime factorization is:

• ( p = 2^1 \cdot 3^2 \cdot 5^1 \cdot a^2 \cdot b^4 )
• ( q = 2^2 \cdot 5^1 \cdot a^3 \cdot b^2 )

Thus, the LCM is: $$ LCM(p, q) = 2^{\max(1, 2)} \cdot 3^{\max(2, 0)} \cdot 5^{\max(1, 1)} \cdot a^{\max(2, 3)} \cdot b^{\max(4, 2)} $$ Which simplifies to: $$ LCM(p, q) = 2^2 \cdot 3^2 \cdot 5^1 \cdot a^3 \cdot b^4 = 180a^3b^4 $$