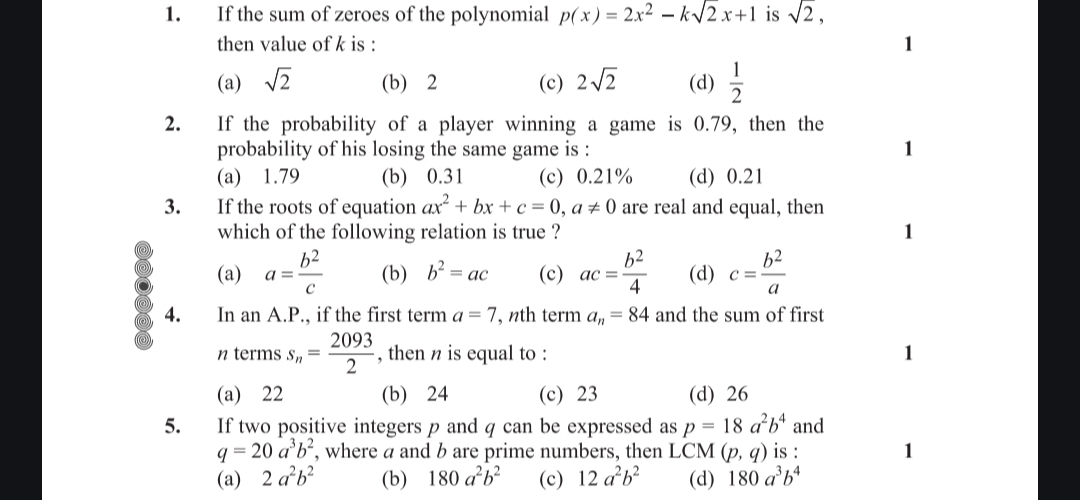
1. If the sum of zeroes of the polynomial p(x) = 2x^2 - k√2x + 1 is √2, then the value of k is: (a) √2 (b) 2 (c) 2√2 (d) 1/2 2. If the probability of a player winning a game is 0.7... 1. If the sum of zeroes of the polynomial p(x) = 2x^2 - k√2x + 1 is √2, then the value of k is: (a) √2 (b) 2 (c) 2√2 (d) 1/2 2. If the probability of a player winning a game is 0.79, then the probability of his losing the same game is: (a) 1.79 (b) 0.31 (c) 0.21 (d) 0.21 3. If the roots of equation ax^2 + bx + c = 0, a ≠ 0 are real and equal, then which of the following relation is true? (a) a = b^2/c (b) b^2 = ac (c) ac = b^2/4 (d) c = b^2/a 4. In an A.P., if the first term a = 7, nth term a_n = 84 and the sum of first n terms S_n = 2093/2, then n is equal to: (a) 22 (b) 24 (c) 23 (d) 26 5. If two positive integers p and q can be expressed as p = 18a^2b^4 and q = 20a^3b^2, where a and b are prime numbers, then LCM(p, q) is: (a) 20a^2b^2 (b) 180a^2b^2 (c) 12a^2b^4 (d) 180a^3b^4 Read more

Steps to Solve

1. Finding the value of ( k )

The sum of the zeroes of a polynomial given by ( p(x) = 2x^2 - k\sqrt{2}x + 1 ) is expressed as ( -\frac{b}{a} ), where ( a = 2 ) and ( b = -k\sqrt{2} ).

[ \text{Sum of zeroes} = -\frac{-k\sqrt{2}}{2} = \frac{k\sqrt{2}}{2} ]

Setting this equal to ( \sqrt{2} ):

[ \frac{k\sqrt{2}}{2} = \sqrt{2} ]

Multiply both sides by 2:

[ k\sqrt{2} = 2\sqrt{2} ]

Dividing by ( \sqrt{2} ):

[ k = 2 ]

2. Finding the probability of losing the game

The probability of winning is 0.79. Since the total probability must equal 1, the probability of losing, ( P(L) ), is given by:

[ P(L) = 1 - P(W) ]

Substituting the values:

[ P(L) = 1 - 0.79 = 0.21 ]

3. Finding the relation for equal roots

For the roots of the equation ( ax^2 + bx + c = 0 ) to be equal, the discriminant must equal zero:

[ D = b^2 - 4ac = 0 ]

This leads to the relation:

[ b^2 = 4ac ]

4. Finding ( n ) in the A.P.

Given:

• First term ( a = 7 )
• Last term ( a_n = 84 )
• Sum of the first ( n ) terms ( S_n = \frac{n}{2}(a + a_n) = 2093 )

Substituting the known values:

[ S_n = \frac{n}{2}(7 + 84) = 2093 ]

This simplifies to:

[ \frac{n \cdot 91}{2} = 2093 ]

Multiplying through by 2:

[ n \cdot 91 = 4186 ]

Dividing both sides by 91 gives:

[ n = \frac{4186}{91} = 46 ]

5. Finding the LCM of ( p ) and ( q )

Given ( p = 18a^2b^4 ) and ( q = 20a^2b^2 ):

First, factor them to their prime bases:

[ p = 2 \times 3^2 \times a^2 \times b^4 ] [ q = 2^2 \times 5 \times a^2 \times b^2 ]

To find the LCM, take the highest power of each prime:

[ \text{LCM}(p, q) = 2^2 \times 3^2 \times 5 \times a^2 \times b^4 = 180a^2b^4 ]