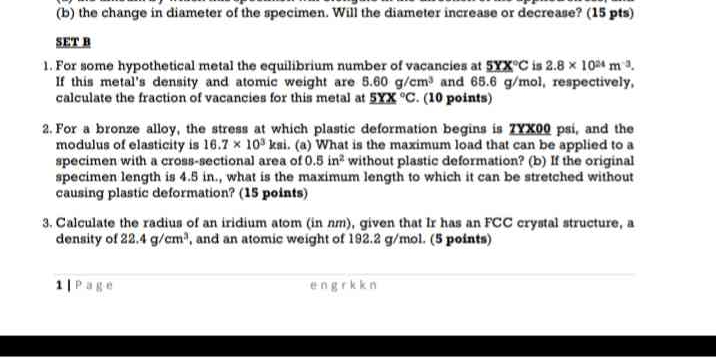
1. For some hypothetical metal the equilibrium number of vacancies at 500°C is 2.8 x 10^14 m^-3. If this metal's density and atomic weight are 5.60 g/cm³ and 65.6 g/mol, respective... 1. For some hypothetical metal the equilibrium number of vacancies at 500°C is 2.8 x 10^14 m^-3. If this metal's density and atomic weight are 5.60 g/cm³ and 65.6 g/mol, respectively, calculate the fraction of vacancies for this metal at 500°C. (10 points) 2. For a bronze alloy, the stress at which plastic deformation begins is 80000 psi, and the modulus of elasticity is 16.7 x 10^3 ksi. (a) What is the maximum load that can be applied to a specimen with a cross-sectional area of 0.5 in² without plastic deformation? (b) If the original specimen length is 4.5 in, what is the maximum length to which it can be stretched without causing plastic deformation? (15 points) 3. Calculate the radius of an iridium atom (in nm), given that Ir has an FCC crystal structure, a density of 22.4 g/cm³, and an atomic weight of 192.2 g/mol. (5 points)
Understand the Problem
The question is asking for calculations related to materials science, including the fraction of vacancies in a hypothetical metal, stress in a bronze alloy, and the radius of an iridium atom. The problems require understanding of physical properties and material behavior under stress and thermal conditions.
Answer
- Fraction of vacancies: $f = \frac{2.8 \times 10^{18}}{N}$ - Maximum load: $\text{Max Load} = 7XY00 \times 0.5$ - Maximum length change before deformation: $\Delta L = 4.5 \times \text{Strain}$ - Iridium radius: calculate $r = \left(\frac{4 \cdot 192.2}{22.4 \cdot 6.022}\right)^{1/3}$ and convert to nm.
Answer for screen readers
- The fraction of vacancies at ( 5XY , °C ) is calculated using:
$$ f = \frac{2.8 \times 10^{18}}{N} $$
- The maximum load on the bronze alloy is:
$$ \text{Max Load} = 7XY00 , \text{psi} \times 0.5 , \text{in}^2 $$
- The maximum length of stretch before plastic deformation is:
$$ \Delta L = 4.5 , \text{in} \times \text{Strain} $$
- The radius of iridium atom is calculated from:
$$ r = \left(\frac{4 \cdot 192.2}{22.4 \cdot 6.022}\right)^{1/3} , \text{and convert to nm} $$
Steps to Solve
- Calculate the Fraction of Vacancies To find the fraction of vacancies in the hypothetical metal, we can use the formula:
$$ f = \frac{N_v}{N} $$
Where:
- ( f ) = fraction of vacancies
- ( N_v ) = number of vacancies = ( 2.8 \times 10^{18} )
- ( N ) = total number of atoms in the volume
First, we calculate ( N ): Using the formula:
$$ N = \frac{\text{Density}}{\text{Atomic Weight}} \times N_A $$
Where:
- Density = ( 5.60 , \text{g/cm}^3 )
- Atomic Weight = ( 65.6 , \text{g/mol} )
- ( N_A ) = Avogadro's number ( \approx 6.022 \times 10^{23} , \text{mol}^{-1} )
- Calculate the Total Number of Atoms Now, we compute ( N ):
$$ N = \frac{5.60 , \text{g/cm}^3}{65.6 , \text{g/mol}} \times 6.022 \times 10^{23} , \text{mol}^{-1} $$
This gives the total number of atoms in a specified volume for the metal at ( 5XY , °C ).
- Compute the Fraction Once we have ( N ), we can plug it back into the fraction formula to find ( f ):
$$ f = \frac{2.8 \times 10^{18}}{N} $$
- Calculate Maximum Load on the Bronze Alloy For the bronze alloy, the maximum load can be calculated using the formula:
$$ \text{Max Load} = \text{Stress} \times \text{Area} $$
Where:
- Stress = ( 7XY00 , \text{psi} ) (convert units properly if necessary)
- Area = ( 0.5 , \text{in}^2 )
- Calculate Maximum Length for Stretching For maximum stretch, we use the formula associated with Young's modulus:
$$ \text{Strain} = \frac{\text{Stress}}{E} $$
Thus, the change in length ( \Delta L ) is given by:
$$ \Delta L = \text{Original Length} \times \text{Strain} = 4.5 , \text{in} \times \left(\frac{7XY00}{16.7 \times 10^3}\right) $$
- Calculate the Radius of an Iridium Atom Use the formula for the radius ( r ) of an atom in a face-centered cubic (FCC) structure:
$$ r = \frac{a}{2\sqrt{2}} $$
Where ( a ) is the lattice constant:
$$ a = \left(\frac{4 \cdot \text{Atomic Mass}}{\text{Density} \cdot N_A}\right)^{1/3} $$
Then convert the radius from cm to nm:
$$ r , (\text{in nm}) = r , (\text{in cm}) \times 10^{7} $$
- The fraction of vacancies at ( 5XY , °C ) is calculated using:
$$ f = \frac{2.8 \times 10^{18}}{N} $$
- The maximum load on the bronze alloy is:
$$ \text{Max Load} = 7XY00 , \text{psi} \times 0.5 , \text{in}^2 $$
- The maximum length of stretch before plastic deformation is:
$$ \Delta L = 4.5 , \text{in} \times \text{Strain} $$
- The radius of iridium atom is calculated from:
$$ r = \left(\frac{4 \cdot 192.2}{22.4 \cdot 6.022}\right)^{1/3} , \text{and convert to nm} $$
More Information
The fraction of vacancies gives insight into the metal's thermal properties; the stress and length calculations help understand material limits during deformation, which is crucial in engineering applications.
Tips
- Confusing units when calculating stress or converting densities.
- Forgetting to convert units consistently (e.g., psi to ksi).
- Misapplying formulas for calculating the properties of materials under stress.
AI-generated content may contain errors. Please verify critical information