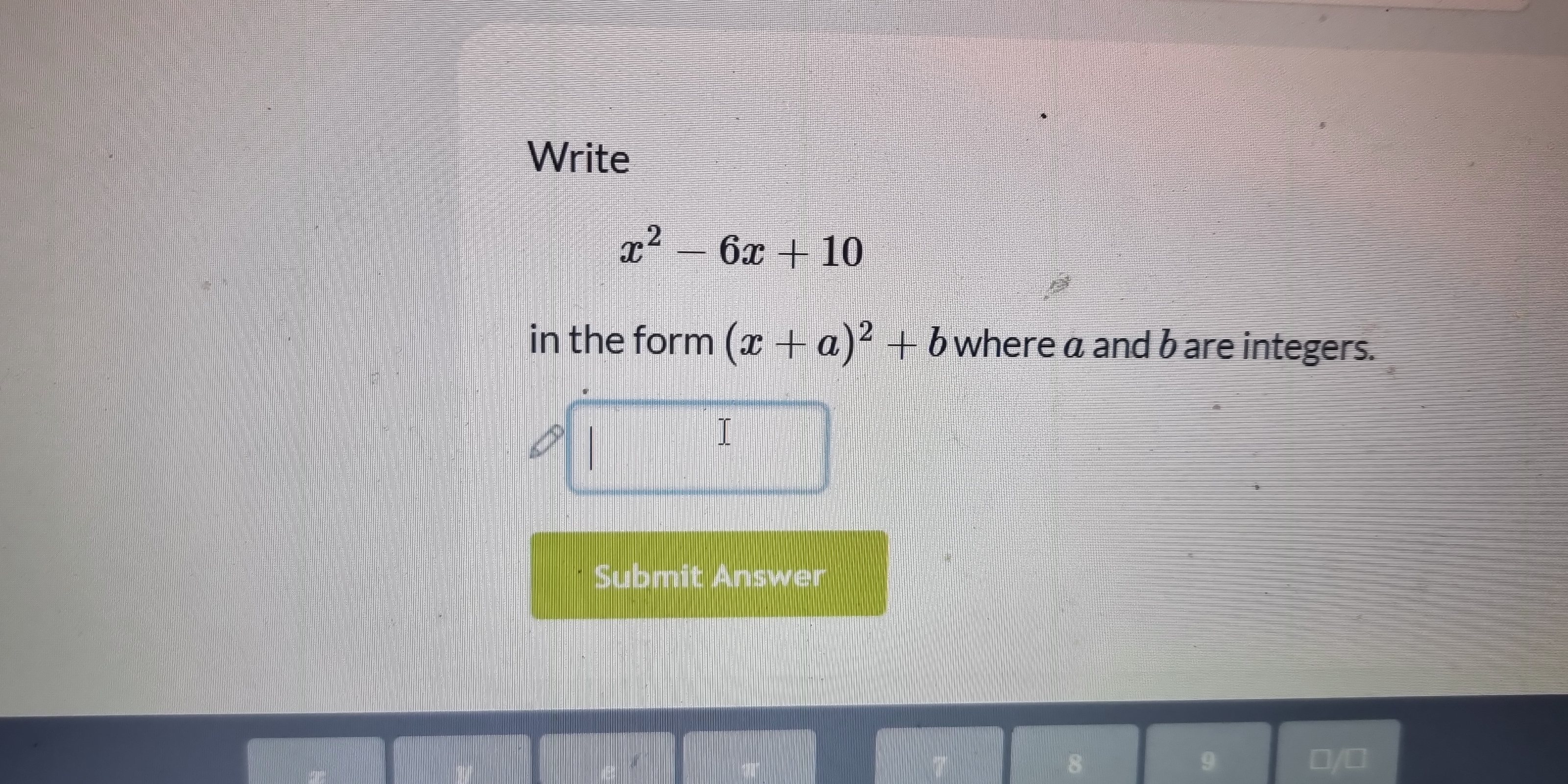
Write x² - 6x + 10 in the form (x + a)² + b where a and b are integers.
Understand the Problem
The question is asking to rewrite the quadratic expression x² - 6x + 10 in a specific form, which is (x + a)² + b, where a and b are integers. This involves completing the square for the quadratic expression.
Answer
The expression is $(x - 3)^2 + 1$.
Answer for screen readers
The expression in the form $(x + a)^2 + b$ is $(x - 3)^2 + 1$ where $a = -3$ and $b = 1$.
Steps to Solve
- Identify the Problem Structure
To rewrite the expression $x^2 - 6x + 10$ in the form $(x + a)^2 + b$, we will complete the square.
- Extract the Coefficient of $x$
We have the quadratic expression: $$ x^2 - 6x + 10 $$
The coefficient of $x$ is -6.
- Complete the Square
To complete the square, take half of the coefficient of $x$, square it, and adjust the expression:
- Half of -6 is -3.
- Squaring -3 gives us $(-3)^2 = 9$.
Now, we rewrite the expression: $$ x^2 - 6x + 9 - 9 + 10 $$
- Rearranging the Expression
Combine the constants: $$ (x - 3)^2 + 1 $$
This shows that $a = -3$ and $b = 1$.
- Final Formulation
The final expression in the desired form is: $$ (x - 3)^2 + 1 $$
The expression in the form $(x + a)^2 + b$ is $(x - 3)^2 + 1$ where $a = -3$ and $b = 1$.
More Information
Completing the square is a useful method for converting a quadratic expression into a form that makes analyzing properties such as vertex and axis of symmetry easier. It can also help in solving quadratic equations.
Tips
- Not squaring the half coefficient correctly. Remember to always square the result from halving the coefficient.
- Forgetting to adjust for the constant term after adding and subtracting the square.
AI-generated content may contain errors. Please verify critical information