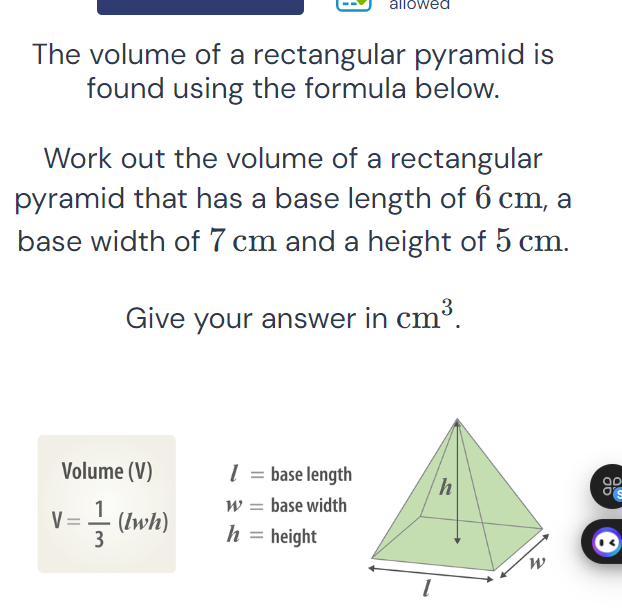
Work out the volume of a rectangular pyramid that has a base length of 6 cm, a base width of 7 cm and a height of 5 cm. Give your answer in cm³.
Understand the Problem
The question is asking to calculate the volume of a rectangular pyramid using the given dimensions: base length (6 cm), base width (7 cm), and height (5 cm), employing the volume formula provided.
Answer
The volume of the rectangular pyramid is $70 \text{ cm}^3$.
Answer for screen readers
The volume of the rectangular pyramid is $70 \text{ cm}^3$.
Steps to Solve
- Identify the dimensions The base length ($l$), base width ($w$), and height ($h$) of the pyramid are given as follows:
- Base length ($l$) = 6 cm
- Base width ($w$) = 7 cm
- Height ($h$) = 5 cm
-
Apply the volume formula The volume $V$ of a rectangular pyramid is calculated using the formula:
$$ V = \frac{1}{3} (lwh) $$
Substituting in the values:
$$ V = \frac{1}{3} (6 \times 7 \times 5) $$ -
Calculate the product of the dimensions First, calculate the product of the dimensions inside the parentheses:
$$ 6 \times 7 \times 5 = 210 $$ -
Calculate the volume Then, apply the fraction to find the volume:
$$ V = \frac{1}{3} (210) = 70 $$ -
State the final answer The volume of the rectangular pyramid is:
$$ V = 70 \text{ cm}^3 $$
The volume of the rectangular pyramid is $70 \text{ cm}^3$.
More Information
The volume of a pyramid is one-third of the base area multiplied by the height. In this case, we used the base dimensions directly to simplify the calculation.
Tips
- Forgetting to divide by 3: It’s essential to remember that the volume formula includes a division by 3, which is often overlooked.
- Miscalculating the dimensions: Double-check multiplication to avoid errors, especially when calculating $l \times w \times h$.
AI-generated content may contain errors. Please verify critical information