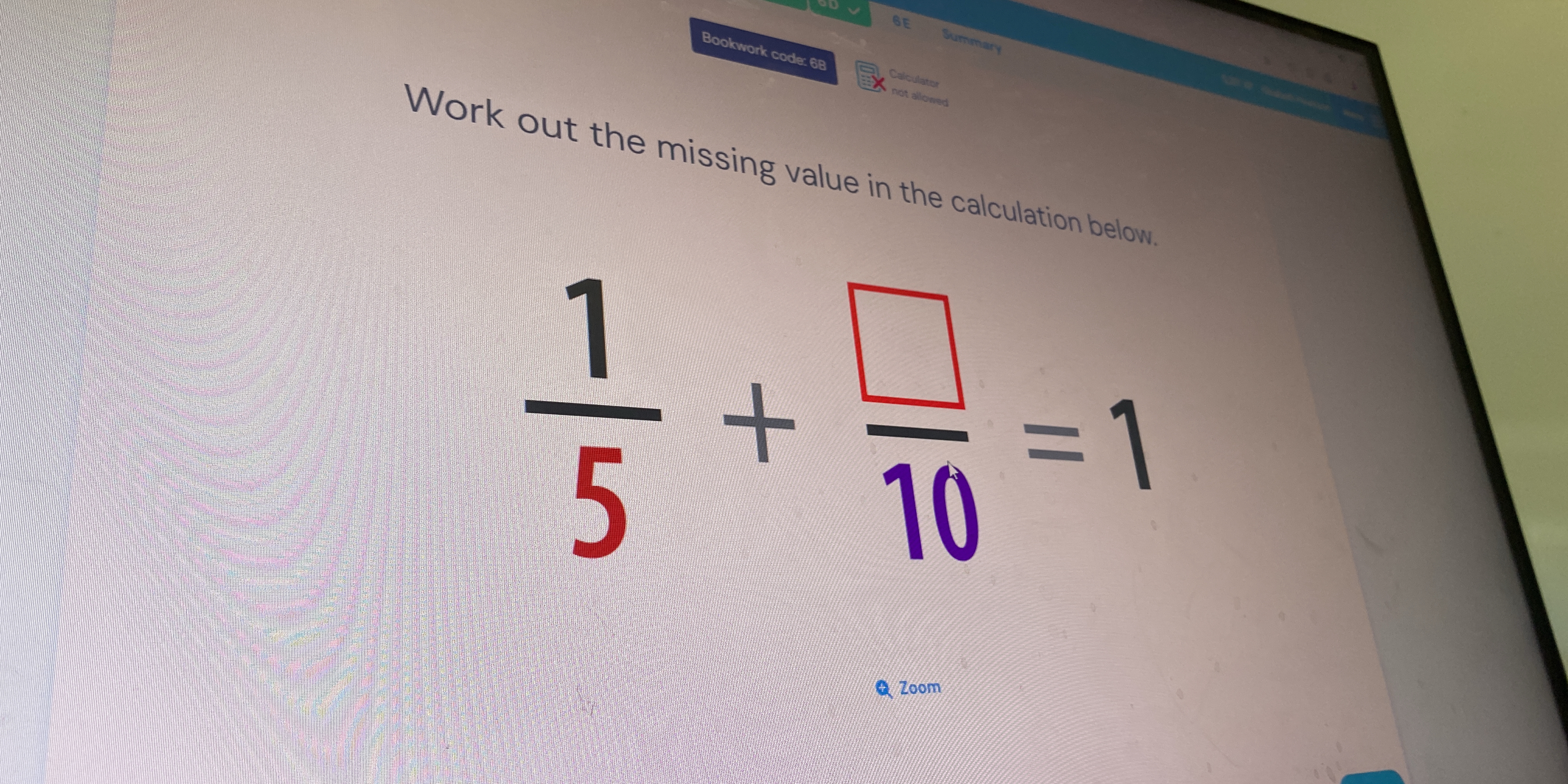
Work out the missing value in the calculation below: 1/5 + ?/10 = 1
Understand the Problem
The question is asking to find the missing value in a mathematical equation, specifically to determine what value should replace the square in the equation 1/5 + ?/10 = 1.
Answer
The missing value is \( x = 8 \).
Answer for screen readers
The missing value is ( x = 8 ).
Steps to Solve
- Convert Fractions to a Common Denominator
To solve the equation ( \frac{1}{5} + \frac{x}{10} = 1 ), we first need a common denominator. The denominator for ( \frac{1}{5} ) can be converted to 10.
We do this by multiplying both the numerator and denominator of ( \frac{1}{5} ) by 2:
$$ \frac{1 \times 2}{5 \times 2} = \frac{2}{10} $$
So, our equation now looks like this:
$$ \frac{2}{10} + \frac{x}{10} = 1 $$
- Combine the Fractions
Next, we can combine the fractions since they now have the same denominator:
$$ \frac{2 + x}{10} = 1 $$
- Isolate the Numerator
To remove the fraction, multiply both sides of the equation by 10:
$$ 2 + x = 10 $$
- Solve for the Missing Value
Now, subtract 2 from both sides to find ( x ):
$$ x = 10 - 2 $$
This simplifies to:
$$ x = 8 $$
The missing value is ( x = 8 ).
More Information
This problem involves finding a missing numerator that will make the sum of two fractions equal to 1. Understanding how to manipulate fractions and recognize common denominators is essential for solving such equations.
Tips
- Not using a common denominator: Ensure that both fractions have the same denominator before combining them.
- Mistakenly subtracting or adding incorrectly: Careful arithmetic is needed to avoid minor calculation errors.
AI-generated content may contain errors. Please verify critical information