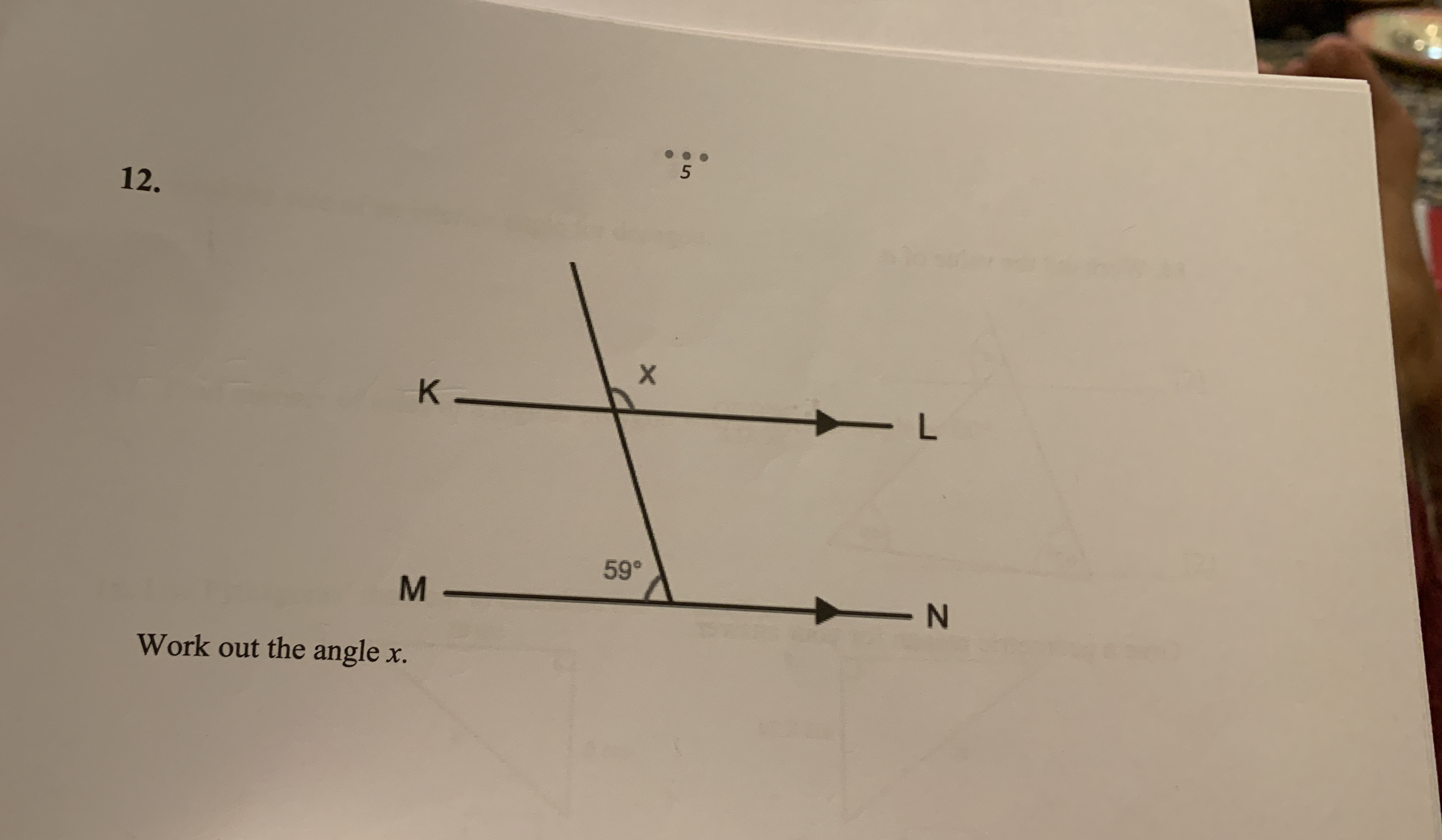
Work out the angle x.
Understand the Problem
The question is asking to calculate the value of angle x, given that one of the angles formed by the intersecting lines is 59 degrees. This requires the application of the properties of angles formed by intersecting lines, particularly the concept of vertically opposite angles or corresponding angles.
Answer
The angle $x$ is $121^\circ$.
Answer for screen readers
The value of angle $x$ is $121^\circ$.
Steps to Solve
- Identify the angles formed by the intersecting lines
When two lines intersect, they form four angles in total. Here, we see that one of the angles is given as $59^\circ$.
- Use properties of vertically opposite angles
The angles that are opposite each other when two lines intersect are equal. Therefore, the angle opposite $59^\circ$ is also $59^\circ$.
- Determine the relationship between angles x and 59 degrees
Since angles on a straight line add up to $180^\circ$, the angles adjacent to $59^\circ$ and $x$ must satisfy the equation: $$ 59^\circ + x = 180^\circ $$
- Solve for x
Rearranging the equation gives: $$ x = 180^\circ - 59^\circ $$
Calculating the right-hand side: $$ x = 121^\circ $$
The value of angle $x$ is $121^\circ$.
More Information
In geometry, the properties of angles formed by intersecting lines are fundamental in solving various problems. Understanding these relationships helps simplify complex angle problems.
Tips
- Confusing adjacent and opposite angles. Remember that vertically opposite angles are equal, whereas adjacent angles add up to $180^\circ$.
AI-generated content may contain errors. Please verify critical information