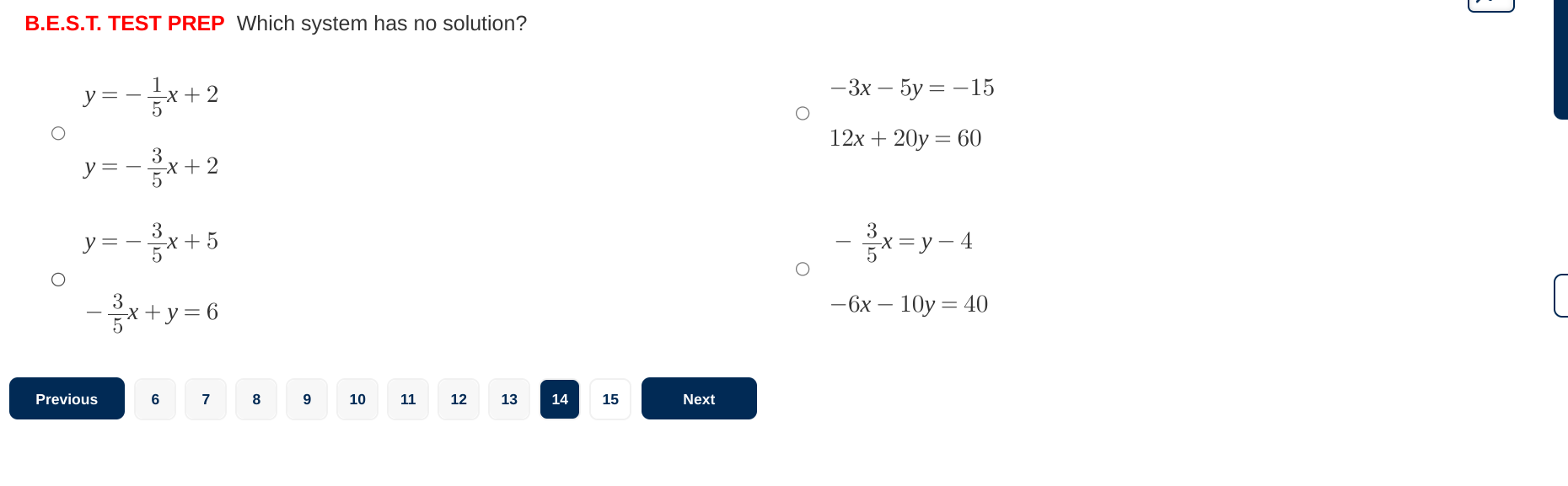
Which system has no solution?
Understand the Problem
The question is asking to identify which of the given systems of equations has no solution, indicating that the lines represented by these equations are parallel and do not intersect.
Answer
The equations with no solution are $y = -\frac{3}{5}x + 2$ and $y = -\frac{3}{5}x + 5$.
Answer for screen readers
The system with no solution is the one represented by the equations:
$y = -\frac{3}{5}x + 2$ and $y = -\frac{3}{5}x + 5$.
Steps to Solve
-
Identify Linear Equations
Extract the equations from the options provided. -
Convert Each Equation to Slope-Intercept Form
Convert all equations to the form $y = mx + b$, where $m$ is the slope:- For example, for $y = -\frac{1}{5}x + 2$, the slope is $-\frac{1}{5}$.
-
Compare Slopes of the Equations
Identify the slopes from each equation:- First equation: Slope = $-\frac{1}{5}$
- Second equation: Slope = $-\frac{3}{5}$
- Third equation: Slope = $-\frac{3}{5}$
- Fourth equation: Rearranging to $y = \frac{3}{5}x + 6$, Slopes = $\frac{3}{5}$
-
Determine Parallel Conditions
Two lines are parallel if they have the same slope but different intercepts. From the slopes identified:- The second and third equations have the same slope ($-\frac{3}{5}$).
-
Check for Different Intercepts
Examine their constant terms:- Second equation: intercept = 2
-
Third equation: intercept = 5
Since they have the same slope but different intercepts, they are parallel.
-
Conclusion
The system of equations that has no solution is the one represented by the second and third equations.
The system with no solution is the one represented by the equations:
$y = -\frac{3}{5}x + 2$ and $y = -\frac{3}{5}x + 5$.
More Information
Parallel lines do not intersect, which means the corresponding system of equations has no solution. This situation often arises in linear algebra, particularly in the study of systems of equations.
Tips
- Assuming that lines with different slopes do not guarantee parallelism. Lines with the same slope and different y-intercepts are the ones that do not intersect at any point.
- Not converting all equations to slope-intercept form can lead to incorrect evaluations of their relationship.
AI-generated content may contain errors. Please verify critical information