Which statement about the functions is true?
Understand the Problem
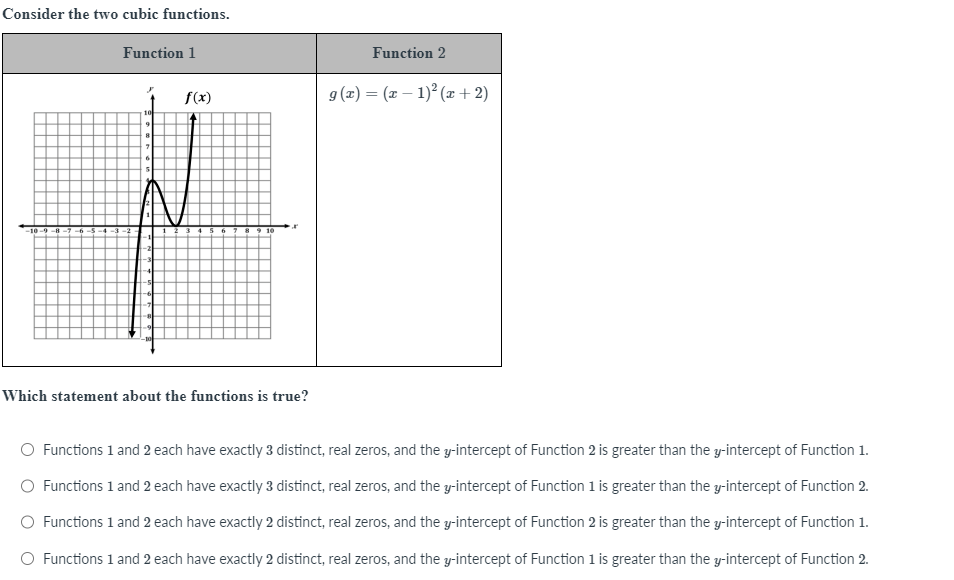
The question is asking to analyze two cubic functions presented in a graph and equation form. Specifically, it seeks to determine which statement accurately describes the properties of these functions, particularly their intercepts and zeros.
Answer
Functions 1 and 2 each have exactly 3 distinct, real zeros, and the $y$-intercept of Function 1 is greater than the $y$-intercept of Function 2.
Answer for screen readers
Functions 1 and 2 each have exactly 3 distinct, real zeros, and the $y$-intercept of Function 1 is greater than the $y$-intercept of Function 2.
Steps to Solve
- Analyze the Graph of Function 1
The graph of Function 1 shows that it is a cubic function. By inspecting the graph, we can find the $x$-intercepts (zeros) where the graph crosses the $x$-axis. This is visually determined at three distinct points, indicating that Function 1 has three zeros.
- Determine the Y-Intercept of Function 1
To find the $y$-intercept of Function 1, we evaluate $f(0)$: $$ f(0) = \text{Value of the function at } x = 0. $$
From the graph, this value can be read directly or calculated if the function is known.
- Analyze Function 2 from Equation
Function 2 is represented as $g(x) = (x - 1)^2 (x + 2)$. To find the zeros, we set $g(x) = 0$: $$ (x - 1)^2 (x + 2) = 0. $$
This gives us the zeros as:
- $x = 1$ (with multiplicity 2),
- $x = -2$.
Thus, Function 2 has 2 distinct real zeros.
- Determine the Y-Intercept of Function 2
To find the $y$-intercept of Function 2, we evaluate $g(0)$: $$ g(0) = (0 - 1)^2 (0 + 2) = 1^2 \cdot 2 = 2. $$
- Compare Y-Intercepts and Properties
Now we can summarize the findings:
- Function 1 has 3 distinct zeros and a certain $y$-intercept (which can be calculated).
- Function 2 has 2 distinct zeros and a $y$-intercept of 2.
Using these observations, we can analyze the statements provided and choose the correct one.
Functions 1 and 2 each have exactly 3 distinct, real zeros, and the $y$-intercept of Function 1 is greater than the $y$-intercept of Function 2.
More Information
Function 1 having three distinct real zeros means it intersects the $x$-axis at three points, whereas Function 2, being squared at $(x-1)$, gives it a "bounce" effect at $x=1$, reducing its distinct zeros to two.
Tips
- Misinterpreting $y$-intercepts, especially mistaking points where the function does not cross the $y$-axis.
- Confusing the number of distinct zeros with their multiplicity. Remember that zeros may be repeated.
AI-generated content may contain errors. Please verify critical information