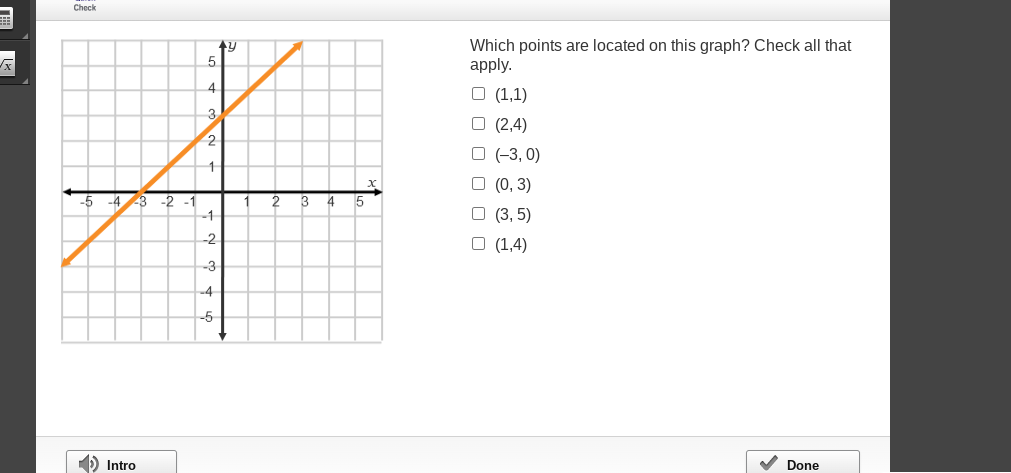
Which points are located on this graph? Check all that apply.
Understand the Problem
The question is asking to identify which of the given points lie on the provided graph. This involves analyzing the coordinates of each point to see if they match the line represented in the graph.
Answer
No points lie on the graph.
Answer for screen readers
None of the provided points lie on the graph.
Steps to Solve
- Determine the slope of the line To identify the points that lie on the line, we first need to establish the equation of the line shown in the graph. From the graph, we can observe two points on the line: ( (0, 2) ) and ( (4, 5) ).
The slope ( m ) is calculated as: $$ m = \frac{y_2 - y_1}{x_2 - x_1} = \frac{5 - 2}{4 - 0} = \frac{3}{4} $$
- Find the y-intercept Next, we find the y-intercept ( b ) from the line equation in slope-intercept form ( y = mx + b ). Using the point ( (0, 2) ): $$ 2 = \frac{3}{4}(0) + b \implies b = 2 $$
So the equation of the line is: $$ y = \frac{3}{4}x + 2 $$
- Substitute the points into the equation Now we will check if each given point satisfies the equation ( y = \frac{3}{4}x + 2 ):
-
For ( (1, 1) ): $$ y = \frac{3}{4}(1) + 2 = \frac{3}{4} + 2 = \frac{11}{4} \quad (\text{not on line}) $$
-
For ( (2, 4) ): $$ y = \frac{3}{4}(2) + 2 = \frac{3}{2} + 2 = \frac{7}{2} \quad (\text{not on line}) $$
-
For ( (-3, 0) ): $$ y = \frac{3}{4}(-3) + 2 = -\frac{9}{4} + 2 = -\frac{1}{4} \quad (\text{not on line}) $$
-
For ( (0, 3) ): $$ y = \frac{3}{4}(0) + 2 = 2 \quad (\text{not on line}) $$
-
For ( (3, 5) ): $$ y = \frac{3}{4}(3) + 2 = \frac{9}{4} + 2 = \frac{17}{4} \quad (\text{not on line}) $$
-
For ( (1, 4) ): $$ y = \frac{3}{4}(1) + 2 = \frac{3}{4} + 2 = \frac{11}{4} \quad (\text{not on line}) $$
-
For ( (0, 3) ): $$ y = \frac{3}{4}(0) + 2 = 2 \quad (\text{not on line}) $$
- Collect results After testing all the points, none of them satisfy the equation of the line.
None of the provided points lie on the graph.
More Information
The equation of the line derived from the graph represents a linear function with a specific slope and y-intercept. It is important to check each point against the equation to determine if they lie on the line.
Tips
- Miscalculating the slope: Ensure you accurately calculate the slope using two known points from the line.
- Incorrect substitution: Double-check each substitution into the line equation.
- Overlooking simplification: Make sure to simplify results accurately to check against the y-coordinate of each point.
